A block of mass m is placed at rest on a horizontal rough surface with angle of friction ϕ. The block is pulled with a force F at an angle θ with the horizontal. The minimum value of F required to move the block is
a. mgsinϕcos(θ−ϕ)
b. mgcosϕcos(θ−ϕ)
c. mgtanϕ
d. mgsinϕ
The correct Answer is:A
To find the minimum value of the force F required to move a block of mass m placed on a horizontal rough surface with an angle of friction ϕ, we can follow these steps:
Step 1: Draw the Free Body Diagram (FBD)
- Consider the block on the horizontal surface.
- The forces acting on the block are:
- The gravitational force mg acting downwards.
- The normal force N acting upwards.
- The applied force F at an angle θ with the horizontal, which can be resolved into two components:
- Horizontal component: Fcosθ
- Vertical component: Fsinθ
- The frictional force fr acting opposite to the direction of motion.
Step 2: Set Up the Equations of Motion
- In the vertical direction, the forces must balance:
N+Fsinθ=mg
Rearranging gives:
N=mg−Fsinθ(Equation 1)
- In the horizontal direction, the applied force must equal the frictional force:
Fcosθ=fr
The frictional force can be expressed as:
fr=μN
where μ=tanϕ. Substituting N from Equation 1 into this gives:
fr=μ(mg−Fsinθ)
Step 3: Substitute and Rearrange
- Substitute fr into the horizontal force equation:
Fcosθ=μ(mg−Fsinθ)
Expanding this gives:
Fcosθ=μmg−μFsinθ
Step 4: Collect Like Terms
- Rearranging the equation:
Fcosθ+μFsinθ=μmg
Factor out F:
F(cosθ+μsinθ)=μmg
Step 5: Solve for F
- Finally, solving for F:
F=μmgcosθ+μsinθ
Step 6: Substitute μ
- Since μ=tanϕ:
F=tanϕ⋅mgcosθ+tanϕsinθ
Final Result
Thus, the minimum value of F required to move the block is:
F=mgtanϕcosθ+tanϕsinθ
- Consider the block on the horizontal surface.
- The forces acting on the block are:
- The gravitational force mg acting downwards.
- The normal force N acting upwards.
- The applied force F at an angle θ with the horizontal, which can be resolved into two components:
- Horizontal component: Fcosθ
- Vertical component: Fsinθ
- The frictional force fr acting opposite to the direction of motion.
- In the vertical direction, the forces must balance:
N+Fsinθ=mg
Rearranging gives:
N=mg−Fsinθ(Equation 1)
Fcosθ=fr
The frictional force can be expressed as:
fr=μN
where μ=tanϕ. Substituting N from Equation 1 into this gives:
fr=μ(mg−Fsinθ)
- Substitute fr into the horizontal force equation:
Fcosθ=μ(mg−Fsinθ)
Expanding this gives:
Fcosθ=μmg−μFsinθ
- Rearranging the equation:
Fcosθ+μFsinθ=μmg
Factor out F:
F(cosθ+μsinθ)=μmg
- Finally, solving for F:
F=μmgcosθ+μsinθ
- Since μ=tanϕ:
F=tanϕ⋅mgcosθ+tanϕsinθ
Thus, the minimum value of F required to move the block is:
F=mgtanϕcosθ+tanϕsinθ
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Objective Question
32 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Comprehension
9 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
28 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
Two block mandM tied together with an inextensible string are placed at rest on a rought horizontal surface with coefficient of friction μ The block m is pulled with a variable force F at a varying angle θ with the horizontal .The value of θ at which the least value of F is required to move the blocks given by

View SolutionA block of mass m is placed on a horizontla surface. If the block is pulled by applying a force of magnitude F=5mg at an angle θ=37∘, with horizontal as shown in fig. find the acceleration of the block at the given instant.

View SolutionA block of mass m is attached with a massless spring of force constant k. The block is placed over a rough inclined surface for which the coefficient of friction is μ=34. The minimum value of M required to move the block up the plane is (Neglect mass of string, mass of pulley and friction in pulley)
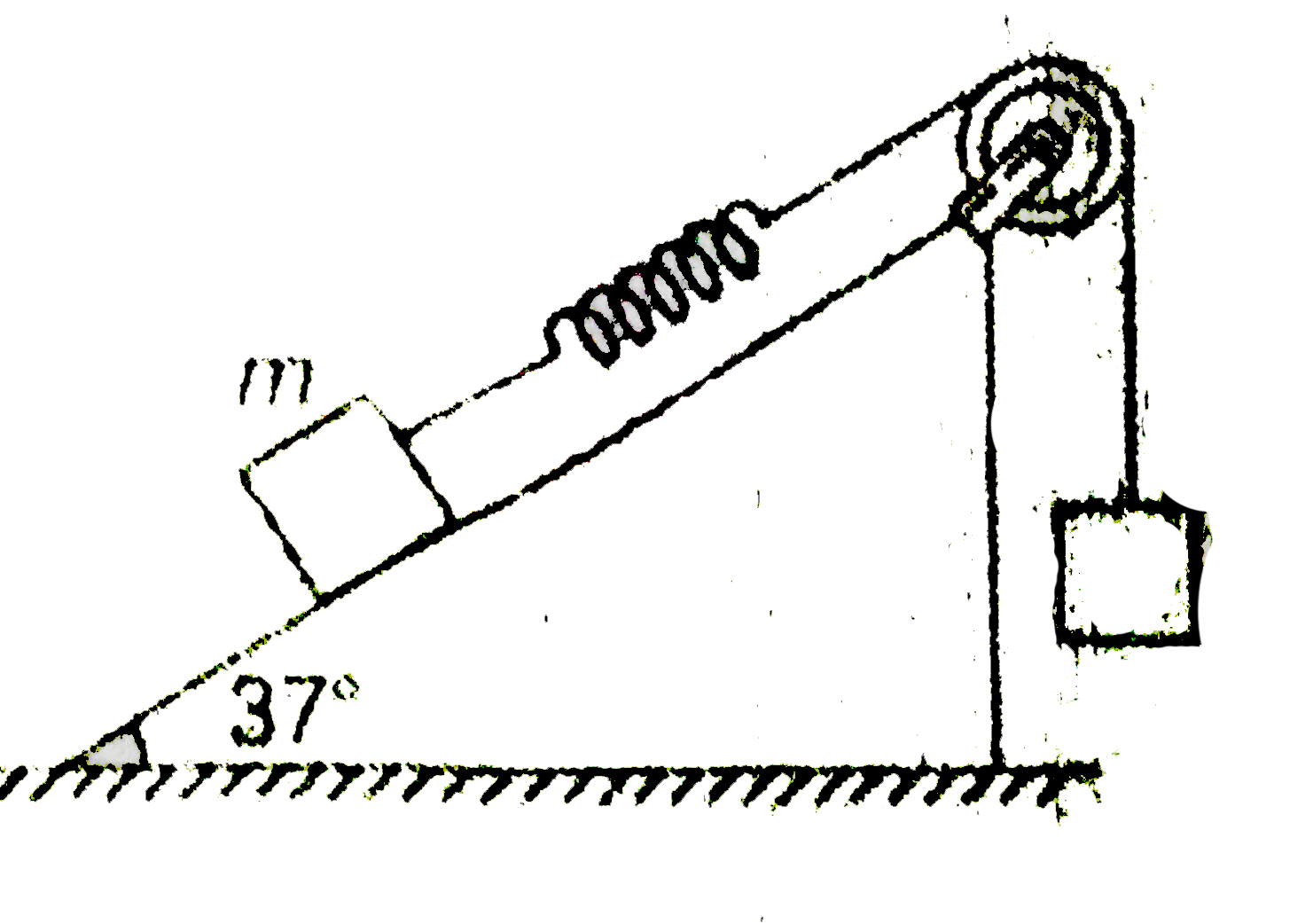
View SolutionA force P acts on a smooth block of mass m placed on a horizontal floor at an angle θ with with horizontal on the block

View SolutionA block of mass m lying on a horizontal surface (coefficient of static friction =μs) is to be brought into motion by a pulling force F At what angle θ with the horizontal should the force F be applied so that its magnitude is minimum ? Also find the minimum magnitude

View SolutionA block of mass m is attached with a massless spring of force constant K. the block is placed over a rough inclined surface for which the coefficient of friction is μ=34 find the minimum value of M required to move the block up the place. (Neglect mass of string and pulley. Ignore friction in pulley).
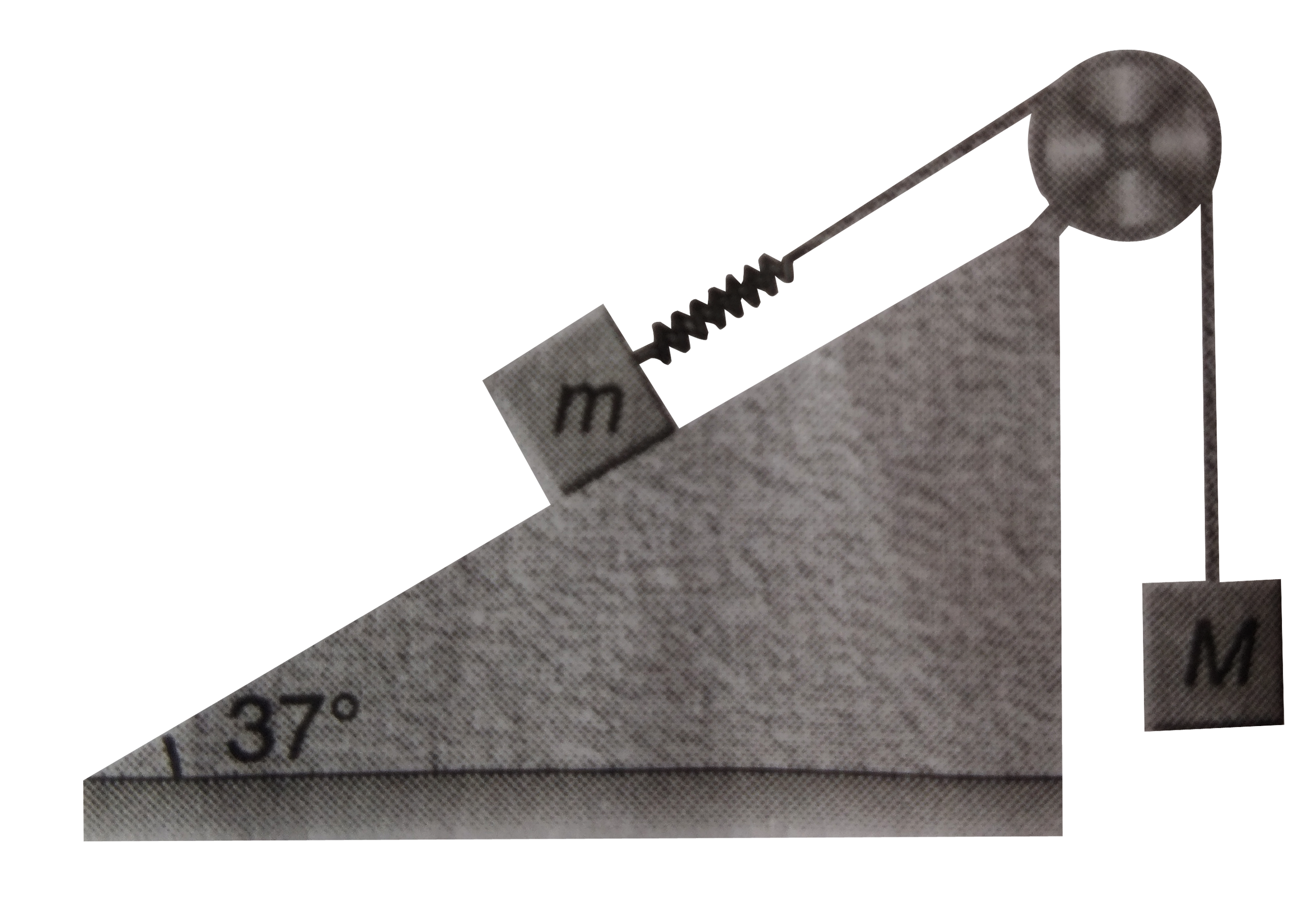 .
.
View SolutionA block of mass 10 kg is placed on the rough horizontal surface. A pulling force F is acting on the block which makes an angle θ above the horizontal. If coefficient of friction between block and surface is 43 then minimum value of force required to just move the block is (g=10ms2)
View SolutionA block of mass m lying on a rough horizontal plane is acted upon by a horizontal force P and another force Q inclined at an angle θ to the vertical. The minimum value of coefficient of friction of friction between the block and the surface for which the block will remain in equilibrium is

View SolutionA block of mass m lying on a rough horizontal plane is acted upon by a horizontal force P and another force Q inclined at an angle θ to the vertical. The minimum value of coefficient of friction of friction between the block and the surface for which the block will remain in equilibrium is

View SolutionA block of mass m lying on a rough horizontal plane is acted upon by a horizontal force P and another force Q inclined at an angle θ to the vertical. The minimum value of coefficient of friction of friction between the block and the surface for which the block will remain in equilibrium is

View Solution
