Question

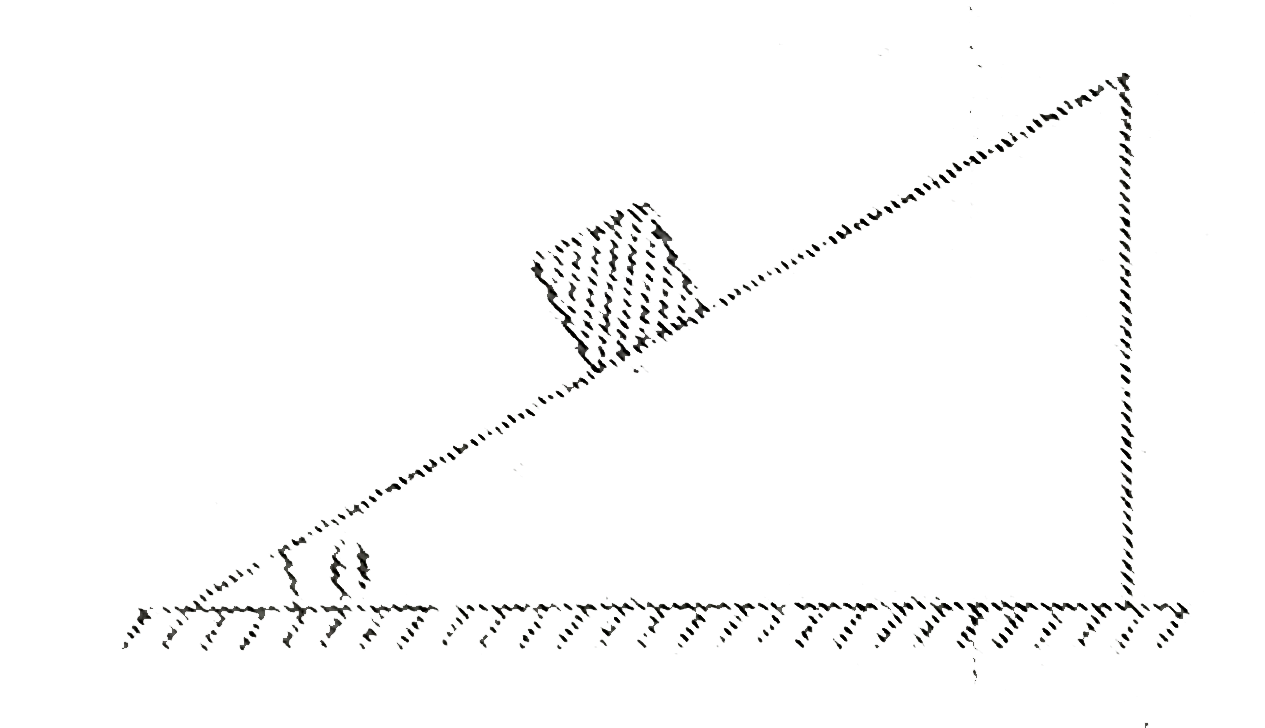
A cube is resting on an inclinded plane. If the angle of inclination is gradually increased. What must be the coefficient of friction between the cube and plane so that,
(a). Cube slides before toppling?
(b). Cube topples before sliding?

Explanation

To solve the problem of determining the coefficient of friction between a cube and an inclined plane such that the cube either slides before toppling or topples before sliding, we can analyze the forces and torques acting on the cube.

Step 1: Identify Forces Acting on the Cube
When the cube is resting on the inclined plane at an angle θ, the following forces act on it:
- Weight (mg) acting vertically downward.
- Normal force (N) acting perpendicular to the inclined plane.
- Frictional force (F) acting parallel to the inclined plane, opposing the motion.

Step 2: Resolve the Weight into Components
The weight of the cube can be resolved into two components:
- Perpendicular to the incline: \( mg \cos \theta \)
- Parallel to the incline: \( mg \sin \theta \)

Step 3: Condition for Sliding Before Toppling
For the cube to slide before toppling, the force causing sliding (which is \( mg \sin \theta \)) must be greater than the maximum static frictional force (which is \( F = \mu N \)):
\( mg \sin \theta > F \)
Since the normal force \( N \) is equal to \( mg \cos \theta \), we can substitute:
\( mg \sin \theta > \mu (mg \cos \theta) \)
Dividing both sides by \( mg \) (assuming \( mg \neq 0 \)):
\( \sin \theta > \mu \cos \theta \)
Dividing both sides by \( \cos \theta \):
\( \tan \theta > \mu \)
Thus, for the cube to slide before toppling, the coefficient of friction must satisfy:
\( \mu < \tan \theta \)

Step 4: Condition for Toppling Before Sliding
For the cube to topple before sliding, we need to analyze the torques about the edge of the cube that is in contact with the inclined plane. The torque due to the normal force (N) must be greater than the torque due to the frictional force (F).

The torque due to the normal force about the edge is:
\( \tau_N = N \cdot \frac{a}{2} \)
The torque due to friction is:
\( \tau_F = F \cdot \frac{a}{2} \)

Setting these two torques equal for rotational equilibrium:
\( N \cdot \frac{a}{2} = F \cdot \frac{a}{2} \)
This simplifies to:
\( N = F \)
Substituting \( F = \mu N \):
\( N = \mu N \)
For this to hold true, we must have:
\( \mu > 1 \)
Thus, for the cube to topple before sliding, the coefficient of friction must satisfy:
\( \mu > 1 \)

Final Results:
(a) The condition for the cube to slide before toppling is:
\( \mu < \tan \theta \)

(b) The condition for the cube to topple before sliding is:
\( \mu > 1 \)