Assertion: Straight line on (p - T) graph for an ideal gas represents isochoric process.
Reason: If p∝T,V=constant.
If both Assertion and Reason are true and the reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true, but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:D
To solve the question, we need to analyze the assertion and the reason provided.
Step 1: Understand the Assertion
The assertion states that a straight line on a (p - T) graph for an ideal gas represents an isochoric process. An isochoric process is one where the volume remains constant.
Step 2: Analyze the (p - T) Graph
In a (p - T) graph:
- Pressure (p) is plotted on the y-axis.
- Temperature (T) is plotted on the x-axis.
For an ideal gas, the relationship between pressure, volume, and temperature is given by the ideal gas law:
PV=nRT
Where:
- P = Pressure
- V = Volume
- n = Number of moles of gas
- R = Universal gas constant
- T = Temperature
Step 3: Determine the Nature of the Line
If we consider the case where volume (V) is constant (as in an isochoric process), we can rearrange the ideal gas law to express pressure in terms of temperature:
P=nRVT
This equation shows that pressure is directly proportional to temperature when volume is constant. Therefore, if we plot this relationship, we will get a straight line that passes through the origin.
Step 4: Evaluate the Assertion
The assertion claims that any straight line on the (p - T) graph represents an isochoric process. However, this is not entirely accurate. A straight line on the (p - T) graph indicates a linear relationship between pressure and temperature, but it does not necessarily indicate that the volume is constant unless it specifically passes through the origin. Thus, the assertion is false.
Step 5: Evaluate the Reason
The reason states that if p∝T, then V must be constant. This is true because, from the ideal gas law, if pressure is directly proportional to temperature, the volume must remain constant for a specific amount of gas. Therefore, the reason is true.
Conclusion
- The assertion is false.
- The reason is true.
Thus, the correct answer is that the assertion is false, and the reason is true.
Final Answer
The assertion is false, and the reason is true.
---
The assertion states that a straight line on a (p - T) graph for an ideal gas represents an isochoric process. An isochoric process is one where the volume remains constant.
In a (p - T) graph:
- Pressure (p) is plotted on the y-axis.
- Temperature (T) is plotted on the x-axis.
PV=nRT
Where:
- P = Pressure
- V = Volume
- n = Number of moles of gas
- R = Universal gas constant
- T = Temperature
If we consider the case where volume (V) is constant (as in an isochoric process), we can rearrange the ideal gas law to express pressure in terms of temperature:
P=nRVT
This equation shows that pressure is directly proportional to temperature when volume is constant. Therefore, if we plot this relationship, we will get a straight line that passes through the origin.
The assertion claims that any straight line on the (p - T) graph represents an isochoric process. However, this is not entirely accurate. A straight line on the (p - T) graph indicates a linear relationship between pressure and temperature, but it does not necessarily indicate that the volume is constant unless it specifically passes through the origin. Thus, the assertion is false.
The reason states that if p∝T, then V must be constant. This is true because, from the ideal gas law, if pressure is directly proportional to temperature, the volume must remain constant for a specific amount of gas. Therefore, the reason is true.
- The assertion is false.
- The reason is true.
The assertion is false, and the reason is true.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESEXERCISE - Level 1 Objective10videos
THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES
- BOOK - DC PANDEY ENGLISHCHAPTER - THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESEXERCISE - Level 1 Subjective48videos
THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES
- BOOK - DC PANDEY ENGLISHCHAPTER - THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESEXERCISE - Exercise 20.57videos
THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES
- BOOK - DC PANDEY ENGLISHCHAPTER - THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESEXERCISE - Medical entrance gallary30videos
THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASES
- BOOK - DC PANDEY ENGLISHCHAPTER - UNIT AND DIMENSIONSEXERCISE - Assertion And Reason2videos
UNIT AND DIMENSIONS
Similar Questions
Straight line on (p - T) graph for an ideal gas represents isochoric process.
If p∝T,V=constant.View SolutionAssertion Straight line on V-T graph represents isobaric process.
Reason If V ∝ T, then p= constant i.e., orocess is isobaric.View SolutionAssertion Any straight line on V-T diagram represents iisobaric process.
Reason In isobaric process, if V is doubled, then T will also become two times.View SolutionDraw at P-T curve for isochoric process.
View SolutionDraw at P-T curve for isochoric process.
View SolutionOn the P−T graph of an ideal gas, choose the correct option(s):
View SolutionThe slope of P-V graph for isochoric process is
View SolutionFor the given P-V graph for an ideal gas, chose the correct V-T graph. Process BC is adiabatic.
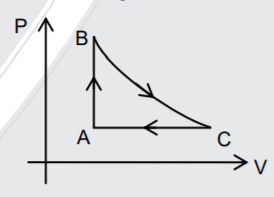 View Solution
View SolutionAssertion: In isochoric process p-V graph is straight line parallel to p-axis.
Reason: In isochoric process density ρ remains constant.View SolutionP-V graph for an ideal gas undergoing polytropic process PVm=constant is shown here. Find the value of m.
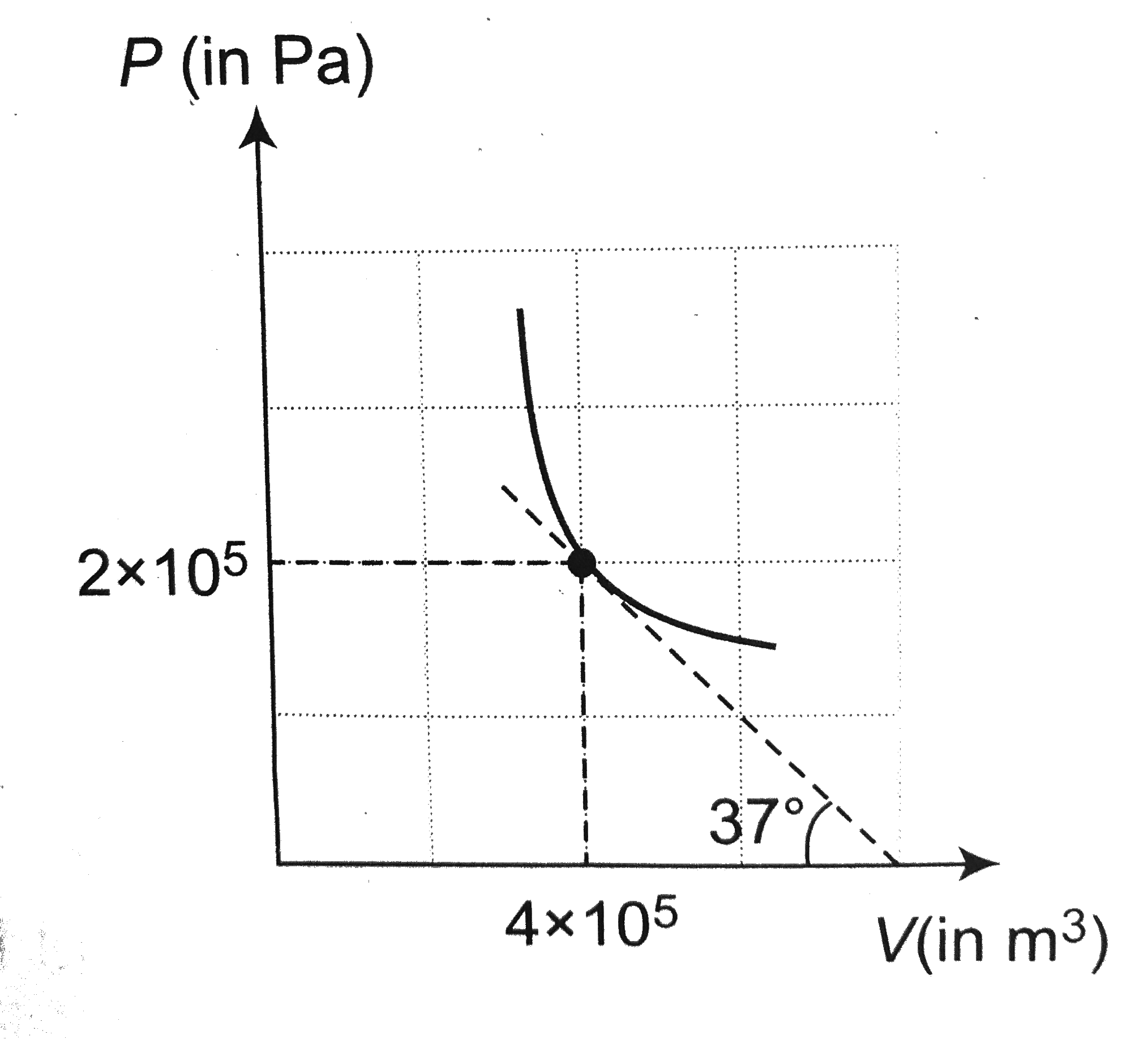 View Solution
View Solution

