Straight line on (p - T) graph for an ideal gas represents isochoric process.
If p∝T,V=constant.
To solve the question regarding the (p - T) graph for an ideal gas during an isochoric process, we will break down the concepts step by step.
1. Understanding Isochoric Process:
An isochoric process is one where the volume (V) of the gas remains constant. This means that any change in pressure (P) must be accompanied by a change in temperature (T).
Hint: Recall that "isochoric" means constant volume.
2. Relationship Between Pressure and Temperature:
For an ideal gas undergoing an isochoric process, we can use the ideal gas law:
PV=nRT
Since volume (V) is constant, we can express pressure in terms of temperature:
P=nRTV
This shows that pressure (P) is directly proportional to temperature (T) when V is constant.
Hint: Remember that the ideal gas law relates pressure, volume, and temperature.
3. Graphical Representation:
If we plot pressure (P) on the y-axis and temperature (T) on the x-axis, the relationship P∝T indicates that the graph will be a straight line. The line will pass through the origin if we consider absolute temperatures and pressures.
Hint: Think about how direct proportionality translates to a straight line in a graph.
4. Evaluating the Statements:
- The first statement claims that a straight line on a (p - T) graph represents an isochoric process. This is not necessarily true because a straight line could also represent other processes (like isobaric) depending on where it intersects the axes.
- The second statement correctly states that pressure is directly proportional to temperature for a constant volume, which is true for an isochoric process.
Hint: Analyze each statement carefully to determine if they hold true under the definitions provided.
5. Conclusion:
- The first statement is incorrect because not all straight lines on a (p - T) graph represent isochoric processes.
- The second statement is correct as it accurately describes the relationship between pressure and temperature in an isochoric process.
Final Answer:
- First statement: Incorrect
- Second statement: Correct
An isochoric process is one where the volume (V) of the gas remains constant. This means that any change in pressure (P) must be accompanied by a change in temperature (T).
For an ideal gas undergoing an isochoric process, we can use the ideal gas law:
PV=nRT
Since volume (V) is constant, we can express pressure in terms of temperature:
P=nRTV
This shows that pressure (P) is directly proportional to temperature (T) when V is constant.
If we plot pressure (P) on the y-axis and temperature (T) on the x-axis, the relationship P∝T indicates that the graph will be a straight line. The line will pass through the origin if we consider absolute temperatures and pressures.
- The first statement claims that a straight line on a (p - T) graph represents an isochoric process. This is not necessarily true because a straight line could also represent other processes (like isobaric) depending on where it intersects the axes.
- The second statement correctly states that pressure is directly proportional to temperature for a constant volume, which is true for an isochoric process.
- The first statement is incorrect because not all straight lines on a (p - T) graph represent isochoric processes.
- The second statement is correct as it accurately describes the relationship between pressure and temperature in an isochoric process.
- First statement: Incorrect
- Second statement: Correct
Topper's Solved these Questions
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assignment (Section-F) (Matrix-match type questions)
3 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assignment (Section-G) (Integer Answer Type Question)
4 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assignment (Section-D) (Linked Comprehension Type Questions)
6 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
15 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
Similar Questions
Assertion: Straight line on (p - T) graph for an ideal gas represents isochoric process.
Reason: If p∝T,V=constant.
View SolutionAssertion Straight line on V-T graph represents isobaric process.
Reason If V ∝ T, then p= constant i.e., orocess is isobaric.
View SolutionDraw at P-T curve for isochoric process.
View SolutionDraw at P-T curve for isochoric process.
View SolutionOn the P−T graph of an ideal gas, choose the correct option(s):
View SolutionAssertion Any straight line on V-T diagram represents iisobaric process.
Reason In isobaric process, if V is doubled, then T will also become two times.
View SolutionThe slope of P-V graph for isochoric process is
View SolutionAssertion: In isochoric process p-V graph is straight line parallel to p-axis.
Reason: In isochoric process density ρ remains constant.
View SolutionFor the given P-V graph for an ideal gas, chose the correct V-T graph. Process BC is adiabatic.
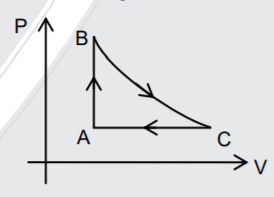
View SolutionP-V graph for an ideal gas undergoing polytropic process PVm=constant is shown here. Find the value of m.
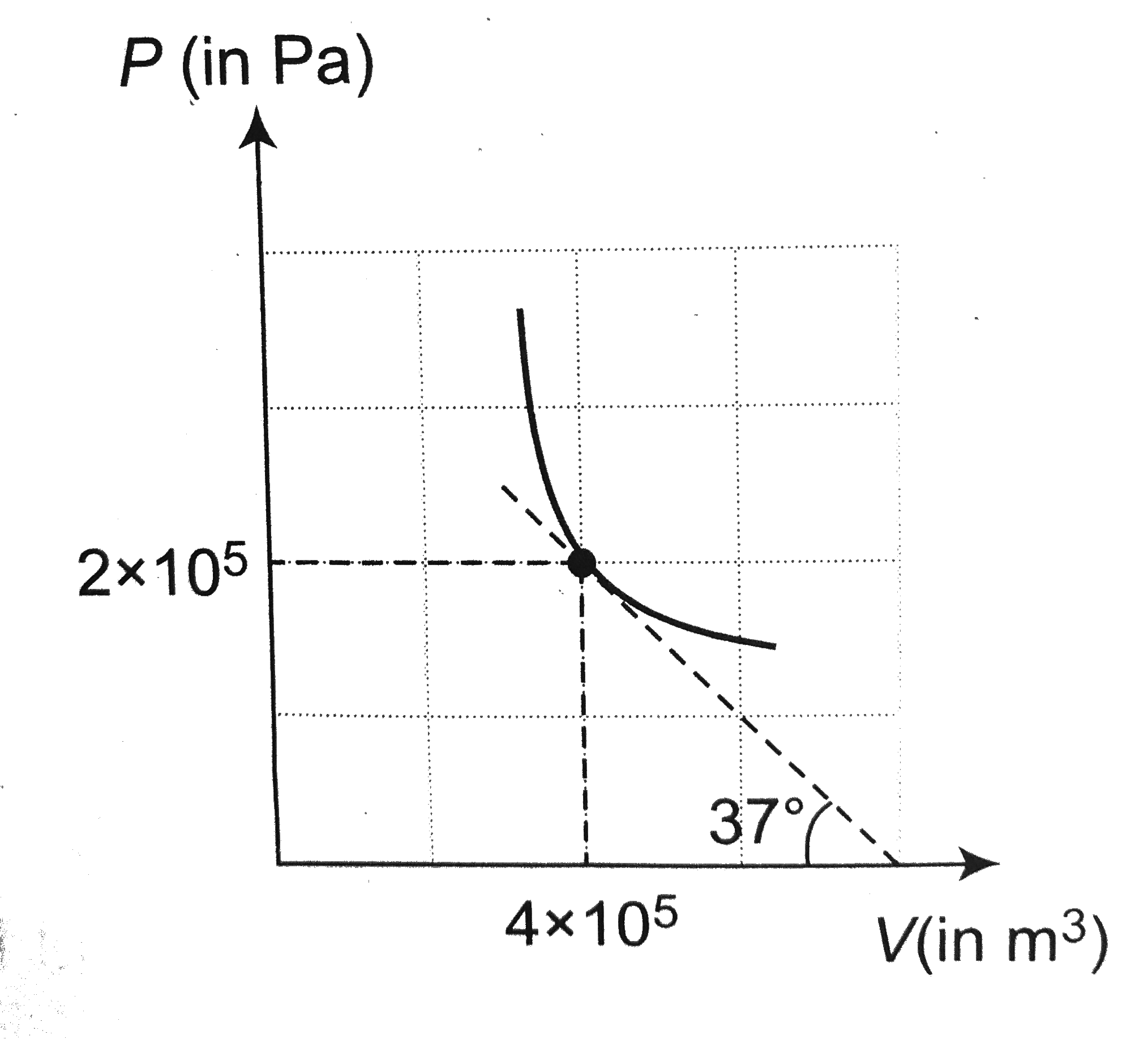
View Solution
