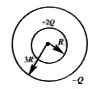
A solid metallic sphere of radius a is surrounded by a conducting spherical shell of radius b(b>a). The solid sphere is given a charge Q. A student measures the potential at the surface of the solid sphere as V and the potential at the surface of spherical shell as Vb. After taking these readings, he decides . to put charge of −4Q on the shell. He then noted the readings of the potential of solid sphere and the shell and found that the potential difference is △V .
He then connected the outer spherical shell to the earth by a conducting wire and found that the charge on the outer surface of the shell as He then decides to remove the earthing connection from the shell and earthed the inner solid sphere. Connecting the inner sphere with the earth he observes the charge on the solid sphere as q2. He then wanted to check what happens if the two are connected by the conducting wire. So he removed the earthing connection and connected a conducting wire between the solid sphere and the spherical shelll. After the connections were made he found the charge on the outer shell as q3.
q2 is
Q
Q(ab)
−4Q
zero
The correct Answer is:B
To solve the problem step by step, we will analyze the situation involving the solid metallic sphere and the conducting spherical shell, considering the charge distributions and potentials at each stage.
Step 1: Initial Setup
- We have a solid metallic sphere of radius a with a charge Q.
- It is surrounded by a conducting spherical shell of radius b where b>a.
- The potential at the surface of the solid sphere is V and at the surface of the shell is Vb.
Step 2: Charge on the Shell
- The student then puts a charge of −4Q on the shell.
- This means the total charge on the shell is now −4Q.
Step 3: Potential Difference
- The potential difference between the solid sphere and the shell is noted as ΔV=V−Vb.
Step 4: Earthing the Shell
- The outer shell is then connected to the earth. When a conductor is earthed, its potential becomes zero.
- Therefore, the charge on the outer shell will adjust such that its potential becomes zero. This will cause the charge on the outer shell to be −Q (as derived from the potential equation).
Step 5: Charge Distribution After Earthing
- The inner surface of the shell will have a charge of −Q (to balance the charge Q on the inner sphere).
- The outer surface of the shell will have a charge of +Q (since the total charge on the shell must remain constant).
Step 6: Earthing the Inner Sphere
- The inner solid sphere is then earthed. When this happens, the potential of the inner sphere becomes zero.
- This means that the charge on the inner sphere will adjust to maintain zero potential. The charge on the inner sphere becomes q2.
Step 7: Charge on the Inner Sphere
- The charge q2 on the inner sphere can be calculated using the formula for potential:
V=kQa+kq2a=0
From this, we can derive:
q2=−Q⋅ab
Step 8: Connecting the Two Spheres
- Finally, the inner sphere and the outer shell are connected by a conducting wire.
- The charges will redistribute until both spheres reach the same potential.
Step 9: Final Charge on the Outer Shell
- After the connection, the charge on the outer shell becomes q3.
- The final charge distribution will depend on the total charge and the radii of the spheres.
Final Answer
The charge on the inner solid sphere, q2, is given by:
q2=−Q⋅ab
- We have a solid metallic sphere of radius a with a charge Q.
- It is surrounded by a conducting spherical shell of radius b where b>a.
- The potential at the surface of the solid sphere is V and at the surface of the shell is Vb.
Step 2: Charge on the Shell
- The student then puts a charge of −4Q on the shell.
- This means the total charge on the shell is now −4Q.
- The potential difference between the solid sphere and the shell is noted as ΔV=V−Vb.
- The outer shell is then connected to the earth. When a conductor is earthed, its potential becomes zero.
- Therefore, the charge on the outer shell will adjust such that its potential becomes zero. This will cause the charge on the outer shell to be −Q (as derived from the potential equation).
- The inner surface of the shell will have a charge of −Q (to balance the charge Q on the inner sphere).
- The outer surface of the shell will have a charge of +Q (since the total charge on the shell must remain constant).
- The inner solid sphere is then earthed. When this happens, the potential of the inner sphere becomes zero.
- This means that the charge on the inner sphere will adjust to maintain zero potential. The charge on the inner sphere becomes q2.
- The charge q2 on the inner sphere can be calculated using the formula for potential:
V=kQa+kq2a=0
From this, we can derive:
q2=−Q⋅ab
- Finally, the inner sphere and the outer shell are connected by a conducting wire.
- The charges will redistribute until both spheres reach the same potential.
- After the connection, the charge on the outer shell becomes q3.
- The final charge distribution will depend on the total charge and the radii of the spheres.
The charge on the inner solid sphere, q2, is given by:
q2=−Q⋅ab
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.14videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.29videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Example Type 83videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises50videos
ELECTROSTATIC POTENTIAL AND CAPACITORS
- BOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions135videos
GRAVITATION
Similar Questions
A solid metallic sphere of radius a is surrounded by a conducting spherical shell of radius b(b>a). The solid sphere is given a charge Q. A student measures the potential at the surface of the solid sphere as V and the potential at the surface of spherical shell as Vb. After taking these readings, he decides . to put charge of −4Q on the shell. He then noted the readings of the potential of solid sphere and the shell and found that the potential difference is △V .
He then connected the outer spherical shell to the earth by a conducting wire and found that the charge on the outer surface of the shell as He then decides to remove the earthing connection from the shell and earthed the inner solid sphere. Connecting the inner sphere with the earth he observes the charge on the solid sphere as q2. He then wanted to check what happens if the two are connected by the conducting wire. So he removed the earthing connection and connected a conducting wire between the solid sphere and the spherical shelll. After the connections were made he found the charge on the outer shell as q3.
Potential difference (△CV) measured by the student between the inner solid shere and outer shell after putting a charge −4Q isView SolutionA solid metal sphere of radius R has a charg +2Q. A hollow spherical shell of radius 3R placed concentric with the frist sphere has net charge -Q.
What would be the final distribution of charges if the sphres are joined by a conducting wireView SolutionA hollow metal sphere of radius 10 cm is charged such that the potential on its surface is 5 V. What is the potential at the centre of the sphere?
View SolutionA hollow metal sphere of radius 10 cm is charged such that the potential on its surface is 5 V. What is the potential at the centre of the sphere?
View SolutionA uniform metal sphere of radius R and mass m is surrounded by a thin uniform spherical shell of same mass and radius 4R. The centre of the shell C falls on the surface of the inner sphere. Find the gravitational fields at points A and B.
 View Solution
View SolutionA uniform metal sphere of radius a and mass M is surrounded by a thin uniform spherical shell of equal mass and redius 4a. The centre of the shell falls on the surface of the iner sphere
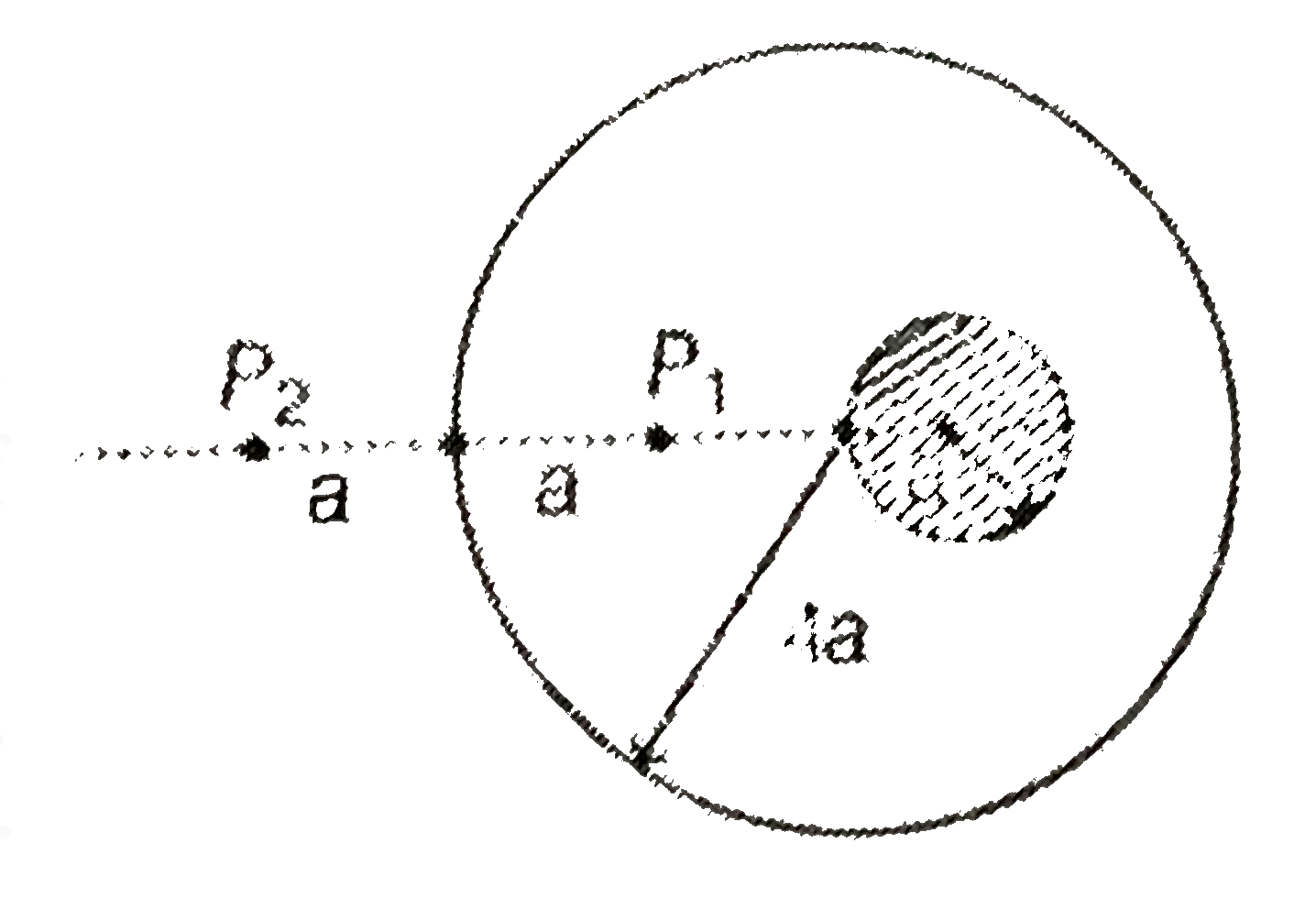 View Solution
View SolutionA hollow metal sphere of radius 6 cm is charged such that the potential on its surface is 12 V.What is the potential at the centre of the sphere?
View SolutionA uniform solid sphere of mass m and radius r is suspended symmetrically by a uniform thin spherical shell of radius 2r and mass m.
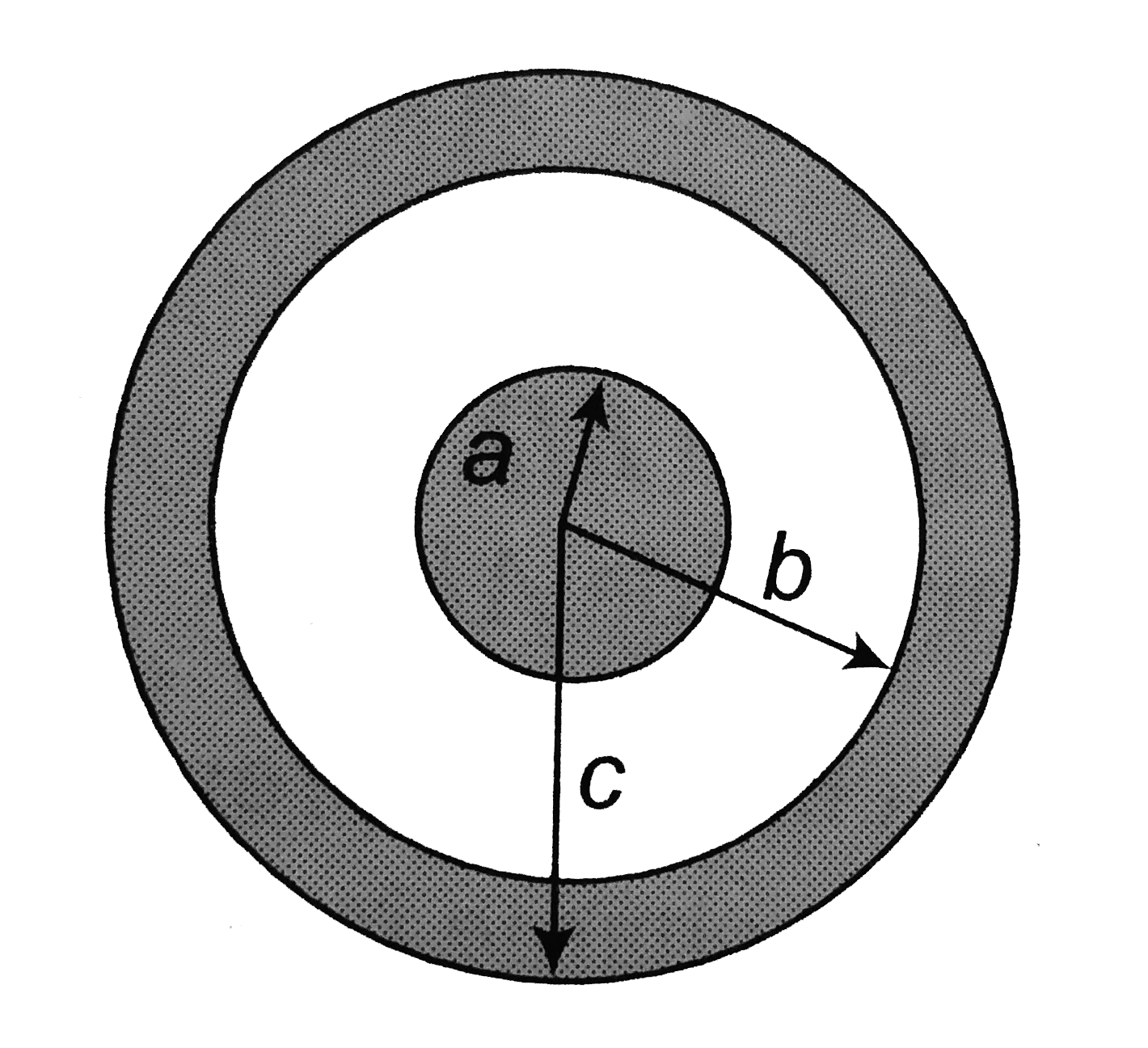
View SolutionA solid conducting sphere of radius a has a net positive charge 2Q. A conducting spherical shell of inner radius b and outer radius c is concentric with the solid sphere and has a net charge −Q. The surface charge density on the inner and outer surfaces of the spherical shell will be
 View Solution
View SolutionA metal sphere of radius 'a' is having charge +Q. Now it is connected by a conducting wire concentric spherical shell of radius '2a'. Then the potential at the surface of outer shell is

Here, K=14πε0View Solution

