A parallel plate capacitor is charged and then the battery is disconnected. When the plates of the capacitor are brought closer, then
energy stored in the capacitor decreases
the potential differences between the plates decreases
the capacitance increases
the electric field between the plates decreases
The correct Answer is:A, B, C
To solve the problem regarding the behavior of a parallel plate capacitor when the plates are brought closer after being charged and disconnected from the battery, we can follow these steps:
1. Understanding the Initial Condition:
- A parallel plate capacitor is charged and then disconnected from the battery. This means that the charge (Q) on the capacitor plates remains constant because there is no external circuit to allow charge to flow away or accumulate.
Hint: Remember that disconnecting the battery means that the charge on the capacitor is fixed.
2. Capacitance Formula:
- The capacitance (C) of a parallel plate capacitor is given by the formula:
C=ε0Ad
where ε0 is the permittivity of free space, A is the area of the plates, and d is the distance between the plates.
Hint: Identify the variables in the capacitance formula and note how they relate to each other.
3. Effect of Bringing Plates Closer:
- When the plates are brought closer together, the distance d decreases. Since capacitance is inversely proportional to the distance d, a decrease in d leads to an increase in capacitance C.
Hint: Think about how changing the distance affects the capacitance based on the formula.
4. Energy Stored in the Capacitor:
- The energy (U) stored in a capacitor is given by the formula:
U=Q22C
Since the charge Q remains constant and capacitance C is increasing, the energy U must decrease because U is inversely proportional to C.
Hint: Consider how energy changes when capacitance changes while keeping charge constant.
5. Potential Difference:
- The potential difference (V) across the capacitor is given by:
V=QC
Again, since Q is constant and C is increasing, the potential difference V must decrease.
Hint: Relate the potential difference to charge and capacitance to see how it changes.
6. Summary of Changes:
- Capacitance (C) increases.
- Energy (U) decreases.
- Potential difference (V) decreases.
Hint: Summarize the relationships you've established to answer the original question.
Final Conclusion:
- The correct statements based on the analysis are:
- The energy stored in the capacitor decreases.
- The potential difference between the plates decreases.
- The capacitance increases.
Thus, options A, B, and C are correct, while the electric field between the plates does not decrease.
- A parallel plate capacitor is charged and then disconnected from the battery. This means that the charge (Q) on the capacitor plates remains constant because there is no external circuit to allow charge to flow away or accumulate.
- The capacitance (C) of a parallel plate capacitor is given by the formula:
C=ε0Ad
where ε0 is the permittivity of free space, A is the area of the plates, and d is the distance between the plates.
- When the plates are brought closer together, the distance d decreases. Since capacitance is inversely proportional to the distance d, a decrease in d leads to an increase in capacitance C.
- The energy (U) stored in a capacitor is given by the formula:
U=Q22C
Since the charge Q remains constant and capacitance C is increasing, the energy U must decrease because U is inversely proportional to C.
- The potential difference (V) across the capacitor is given by:
V=QC
Again, since Q is constant and C is increasing, the potential difference V must decrease.
- Capacitance (C) increases.
- Energy (U) decreases.
- Potential difference (V) decreases.
- The correct statements based on the analysis are:
- The energy stored in the capacitor decreases.
- The potential difference between the plates decreases.
- The capacitance increases.
Thus, options A, B, and C are correct, while the electric field between the plates does not decrease.
Topper's Solved these Questions
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Videos
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY
42 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
Similar Questions
Knowledge Check
A parallel plate capacitor without any dielectric within its plates, has a capacitance C, and is connected to a battery of emf V. The battery is disconnected and the plates of the capacitor are pulled apart until the separation between the plates is doubled. What is the work done by the agent pulling the plates apart, in this process ?
A12CV2B32CV2C−32CV2DCV2
A parallel plate capacitor is charged. If the plates are pulled apart
View SolutionA parallel plate capacitor is charged by a battery. After sometime the battery is disconnected and a dielectric slab with its thickness equal to the plate separation is inserted between the plates. How will (i) the capacitance of the capacitor, (ii) potential difference between the plates and (iii) the energy stored in the capacitor be affected ?
Justify your answer in each case.
View SolutionA parallel plate air capacitor is connected to a battery. If the plates of the capacitor are pulled farther apart, then state whether the following statements are true or false.
a. Strength of the electric field inside the capacitor remains unchanged, if the battery is disconnected before pulling the plates.
b. During the process, work is done by the external force applied to pull the plates irrespective of whether the battery is disconnected or not.
c. Strain energy in the capacitor decreases if the battery remains connected.
 .
.
View SolutionConsider a parallel plate capacitor having charge Q. Then,
View SolutionA parallel plate air capacitor is connected to a battery. After charging fully, the battery is disconnected and the plates are pulled apart to increase their separation. Which of the following statements is correct ?
View SolutionA parallel plate capacitor is charged by a battery. After some time the battery is disconnected and a dielectric slab of dielectric constant K is inserted between the plates. How would (i) the capacitance, (ii) the electric field between the plates and (iii) the energy stored in the capacitor, be affected ? Justify your answer.
View SolutionWhich one is not correct for a cyclic process as shown in the figure ?
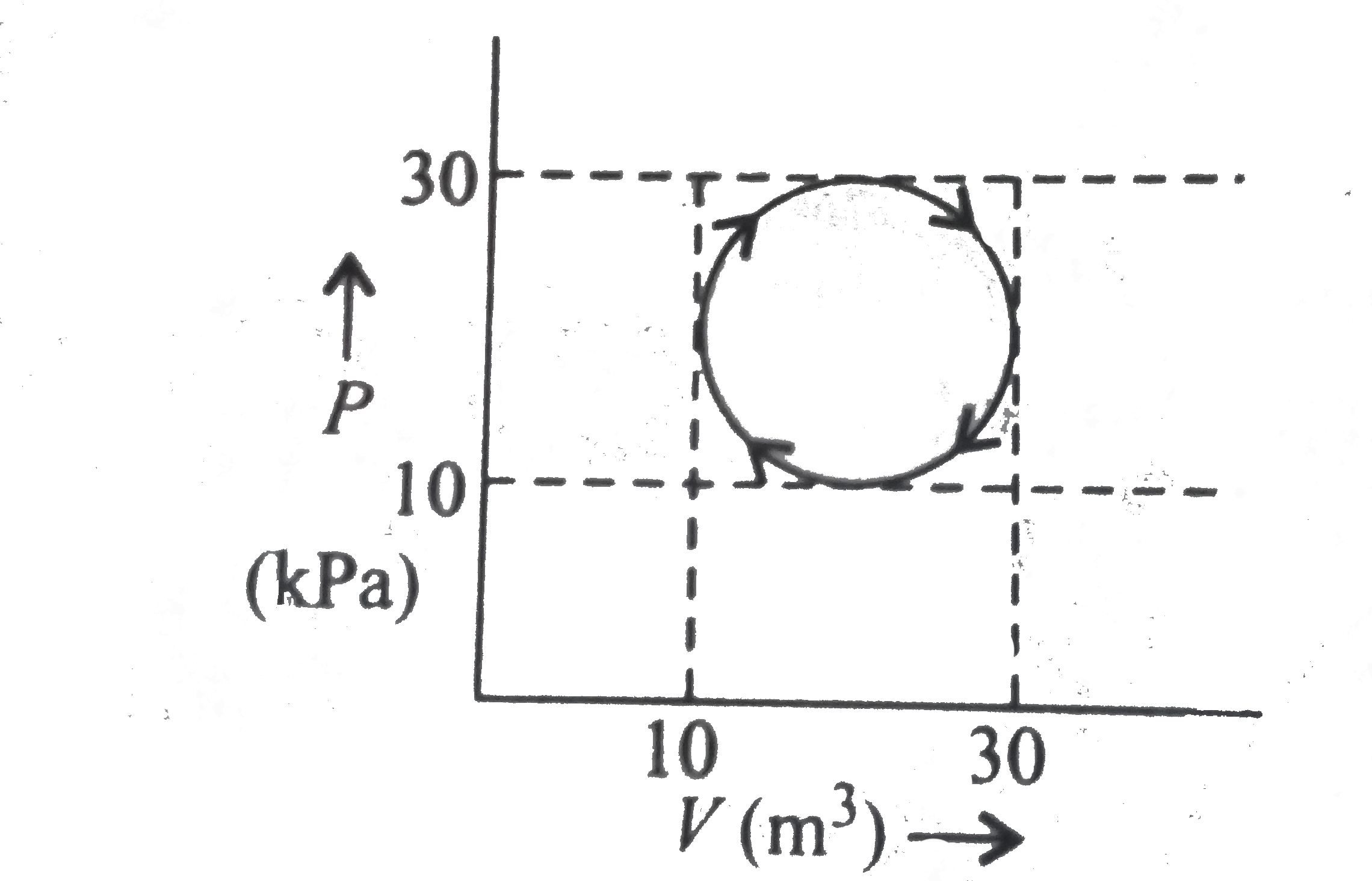
View Solution
