When a loop moves towards a stationary magnet with speed v, the induced emf in the loop is E. If the magnet also moves away from the lop with the same speed, then the emf inducted in the loop is
E
2E
E2
zero
The correct Answer is:D
To solve the problem, we need to analyze the two scenarios presented:
1. When the loop moves towards a stationary magnet.
2. When both the loop and the magnet move away from each other with the same speed.
Step 1: Understand the first scenario.
- In the first scenario, the loop is moving towards the stationary magnet with speed v. This motion causes a change in magnetic flux through the loop, which induces an electromotive force (emf) E in the loop.
Step 2: Apply Faraday's Law of Electromagnetic Induction.
- According to Faraday's Law, the induced emf (E) in a loop is given by:
\(
E = -\frac{d\Phi}{dt}
\)
where \(\Phi\) is the magnetic flux through the loop.
Step 3: Analyze the second scenario.
- In the second scenario, both the loop and the magnet are moving away from each other at the same speed v. This means that the distance between the loop and the magnet is increasing, but since both are moving at the same speed, the relative speed between the loop and the magnetic field created by the magnet is zero.
Step 4: Determine the change in magnetic flux.
- Since the loop is moving away from the magnet at the same speed as the magnet is moving away from the loop, the magnetic field lines that pass through the loop do not change. Therefore, there is no change in magnetic flux (\(d\Phi = 0\)).
Step 5: Calculate the induced emf in the second scenario.
- Applying Faraday's Law again, since there is no change in magnetic flux:
\(
E = -\frac{d\Phi}{dt} = 0
\)
Thus, the induced emf in the loop when both the loop and the magnet move away from each other with speed v is zero.
Final Answer:
The induced emf in the loop when the magnet moves away from it with the same speed v is 0.
---
2. When both the loop and the magnet move away from each other with the same speed.
- In the first scenario, the loop is moving towards the stationary magnet with speed v. This motion causes a change in magnetic flux through the loop, which induces an electromotive force (emf) E in the loop.
- According to Faraday's Law, the induced emf (E) in a loop is given by:
\(
E = -\frac{d\Phi}{dt}
\)
where \(\Phi\) is the magnetic flux through the loop.
- In the second scenario, both the loop and the magnet are moving away from each other at the same speed v. This means that the distance between the loop and the magnet is increasing, but since both are moving at the same speed, the relative speed between the loop and the magnetic field created by the magnet is zero.
- Since the loop is moving away from the magnet at the same speed as the magnet is moving away from the loop, the magnetic field lines that pass through the loop do not change. Therefore, there is no change in magnetic flux (\(d\Phi = 0\)).
- Applying Faraday's Law again, since there is no change in magnetic flux:
\(
E = -\frac{d\Phi}{dt} = 0
\)
Thus, the induced emf in the loop when both the loop and the magnet move away from each other with speed v is zero.
The induced emf in the loop when the magnet moves away from it with the same speed v is 0.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective Questions
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 1 Subjective
21 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 1 Assertion And Reason
10 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
A magnet is moving towards a coil along its axis and the emf induced in the coil is ε . If the coil also strats moving towards the magnet with the same speed, the induced emf will be
A. ε2
B. ε
C. 2ε
D. 4ε
View SolutionA constant current I flows through a long straight wire as shown in figure. A square loop starts moving towards righ with as constant speed v.

a Find induced emf produced i the loop as a function x
b. If total resistance of the loop is R, then find induced current in the loop,
View SolutionAn electron is moving near to a conducting loop then the induced current in the loop is-
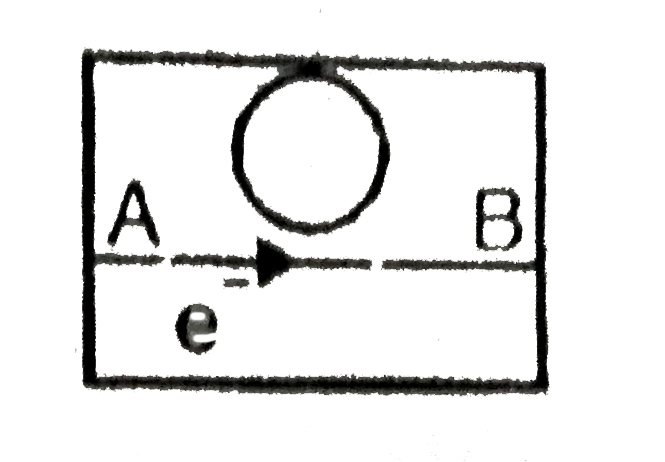
View SolutionAssertion If a loop is placed in a non-uniform (with respect to position) magnetic field, then induced emf is produced in the loop.
Reason In a non-uniform magnetic field, magnetic flux passing through the loop will change. Therefore, induced emf is produced.
View SolutionA conducting circular loop is in a perpendicular magnetic field of induction 10−2T If the radius of the loop starts shrinking at a uniform rate of 1 mm/s, then the e.m.f. induced in the loop at the instant when its radius is 10 cm will be
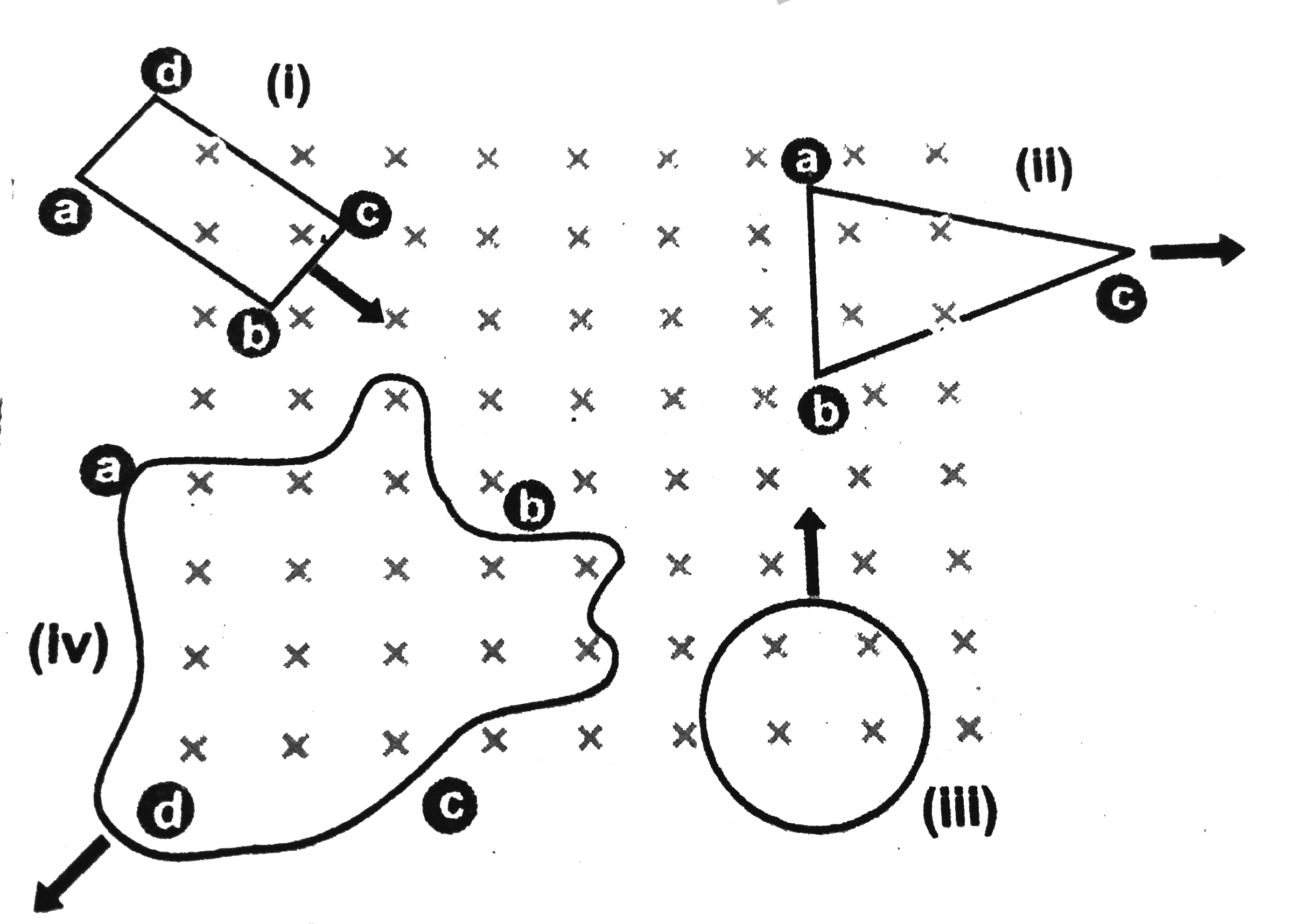
View SolutionFig. shows planer loops of different shapes moving out of or into a region of magnetic field which is directed normal to the plane of the loops away from the reader. Determine the direction of induced current in each loop using Lenz's law. Check if you would obtain the same answers by considering magnetic force on the charge inside the moving loops.
View SolutionA conducting loop of radius R is present in a uniform magnetic field B perpendicular to the plane of the ring. If radius R varies as a function of time t, as R=R0+t. The emf induced in the loop is

View SolutionFigure shows planar loops of different shapes moving out of or into a region of a magnetic field which is directed normal to the plane of the loop away from the reader. Determine the direction of induced current in each loop using Lenz's law.

View SolutionCurrent in a long current carrying wire is I=2t
A conducting loop is placed to the right of this wire. Find
a. magnetic flux ϕB passing through the loop.
b. induced emf|e| prodced in the loop.
c. if total resistance of the loop is R, then find induced current Iin in the loop.
View SolutionA metallic loop is placed in a nonuniform magnetic field. Will an emf be induced in the loop?
View Solution
