In an AC circuit, the impedance is √3 times the reactance, then the phase angle is
60∘
30∘
zero
none of these
The correct Answer is:D
To solve the problem step by step, we will analyze the relationship between impedance (Z), reactance (X), and phase angle (φ) in an AC circuit.
Step 1: Understand the relationship given in the problem
We are given that the impedance (Z) is equal to √3 times the reactance (X). This can be expressed mathematically as:
Z=√3X
Step 2: Recall the impedance triangle
In an AC circuit, the impedance triangle relates the resistance (R), reactance (X), and impedance (Z). The triangle is formed as follows:
- The base represents the resistance (R).
- The perpendicular side represents the reactance (X).
- The hypotenuse represents the impedance (Z).
Step 3: Use the definition of sine in the impedance triangle
From the impedance triangle, we can relate the sine of the phase angle (φ) to the reactance and impedance:
sinϕ=XZ
Step 4: Substitute the given relationship into the sine equation
Substituting Z=√3X into the sine equation, we get:
sinϕ=X√3X
This simplifies to:
sinϕ=1√3
Step 5: Find the phase angle (φ)
To find the phase angle (φ), we take the inverse sine:
ϕ=sin−1(1√3)
Step 6: Calculate the value of φ
Using a calculator or trigonometric tables, we find:
ϕ≈35.26∘
Step 7: Determine the correct option
The options given were 60°, 30°, 0°, and none of these. Since 35.26° is not listed among the options, the correct answer is:
None of these.
Summary of the Solution
The phase angle (φ) in the given AC circuit is approximately 35.26°, which corresponds to the answer "none of these."
---
We are given that the impedance (Z) is equal to √3 times the reactance (X). This can be expressed mathematically as:
Z=√3X
In an AC circuit, the impedance triangle relates the resistance (R), reactance (X), and impedance (Z). The triangle is formed as follows:
- The base represents the resistance (R).
- The perpendicular side represents the reactance (X).
- The hypotenuse represents the impedance (Z).
From the impedance triangle, we can relate the sine of the phase angle (φ) to the reactance and impedance:
sinϕ=XZ
Substituting Z=√3X into the sine equation, we get:
sinϕ=X√3X
This simplifies to:
sinϕ=1√3
To find the phase angle (φ), we take the inverse sine:
ϕ=sin−1(1√3)
Using a calculator or trigonometric tables, we find:
ϕ≈35.26∘
The options given were 60°, 30°, 0°, and none of these. Since 35.26° is not listed among the options, the correct answer is:
None of these.
The phase angle (φ) in the given AC circuit is approximately 35.26°, which corresponds to the answer "none of these."
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Level - 1 Subjective
6 Videos
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Level- 2 Single Correct
18 Videos
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Level 1 Assertion-Reason
11 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY
42 Videos
Similar Questions
Knowledge Check
Assertion : In series LCR resonance circuit, the impedance is equal to the ohmic resistance.
Reason : At resonance, the inductive reactance exceeds the capacitive reactance.AIf both assertion ans reason are true ans reaason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion istrue but reason is false.DIf both assertion and reason are false.In the series LCR circuit shown the impedance is
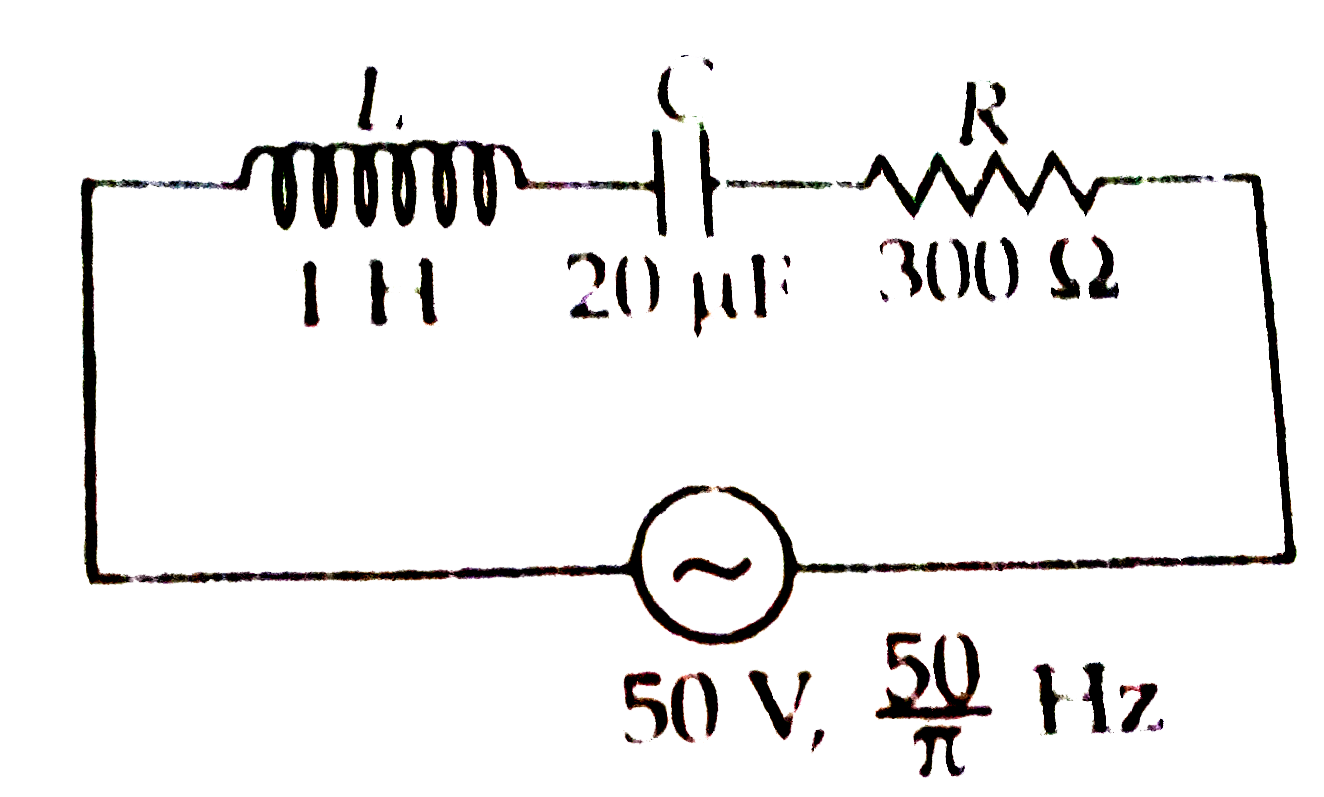 A200ΩB100ΩC300ΩD500Ω
A200ΩB100ΩC300ΩD500ΩThe vertical component of earth's magnetic field at a place is √3 times the horizontal component the value of angle of dip at this place is
A30∘B45∘C60∘D75∘
If for above circuit the capacitive reactance is two times of inductive Reactance, and resistance R is equal to inductance Reactance then power factor of circuit is .

View SolutionIn L-R circuit, resistance is 8Ω and inductive reactance is 6Ω then impedance is:
View SolutionThe vertical component of earth's magnetic field at a place is √3 times the horizontal component the value of angle of dip at this place is
View SolutionAt a certain place , horizontal component of earth's field is √3 times the vertical component of earth's field . The angle of dip at this place is
View SolutionIn an AC circuit, a resistance of Rohm is connected is series with an inductance L. If phase angle between volage and current be 45∘, the value of inductive reactance will be
View Solution
