If for above circuit the capacitive reactance is two times of inductive Reactance, and resistance R is equal to inductance Reactance then power factor of circuit is .

1
zero
12
2√5
The correct Answer is:D
XX=2XL=2R
1z2=1R2+(1XL−1XC)2
=1R2+(12R−1R)2=54R2z=2R√5
power factor in parallel combination
=cosθ=zR=2√5
Alternate solution :
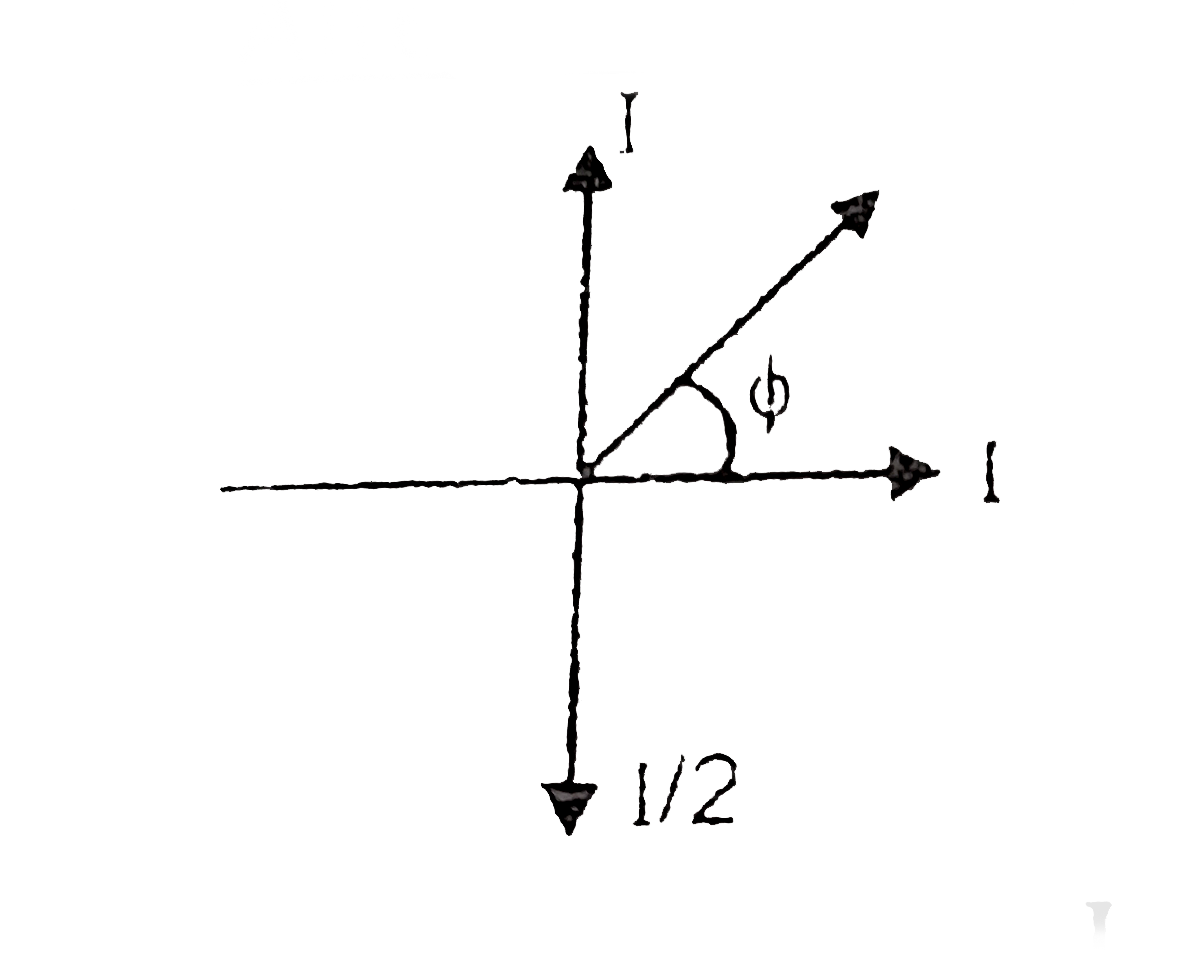
cosϕ=I√I2+I24+2√5.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.59
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.60
8 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.57
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
The capacitive reactance in an A.C. circuit is
View SolutionThe impedance of a circuit consister of 3Ωresistance and 4Ω reactance. The power factor of the circuit is
View SolutionIn an AC circuit, the impedance is √3 times the reactance, then the phase angle is
View SolutionIn L-R circuit, resistance is 8Ω and inductive reactance is 6Ω then impedance is:
View SolutionThe impedance of a series circuit consists of 3ohm resistance and 4ohm reactance.The power factor of the circuit is
View SolutionA wire of reistance R is connected in series with an inductor of reactance ω L. Then quality factor of RL circuit is
View SolutionZero reactance is possible in which circuit?
View SolutionA series AC circuit has a resistance of 4Ω and a reactance of 3Ω. The impedance of the circuit is
View SolutionA series AC circuit has a resistance of 4Ω and a reactance of 3Ω. The impedance of the circuit is
View Solution200V A.C. is applied at the ends of an LCR circuit. The circuit consists of an inductive reactance XL=50Ω capacitive reactance Xc=50Ω and ohmic resistance R = 10 Ω . Calculate the impedance of the circuit and also potential differences across L and R What will be the potential difference across L-C
View Solution
