In a double slit pattern (λ=6000Å), the first order and tenth order maxima fall at 12.50 mm and 14.75mm from a particular reference point. If λ is changed to 5500Å, find the position of zero order and tenth order fringes, other arrangements remaining the same.
To solve the problem step by step, we will use the information provided regarding the positions of the maxima and the relationship between fringe width and wavelength.
Step 1: Determine the original fringe width
Given:
- Position of the first order maximum (y1) = 12.50 mm
- Position of the tenth order maximum (y10) = 14.75 mm
The distance between the first and tenth order maxima can be calculated as:
y10−y1=14.75mm−12.50mm=2.25mm
The number of fringes between the first and tenth order maxima is:
10−1=9
Thus, the fringe width (ω) can be calculated as:
ω=y10−y19=2.25mm9=0.25mm
Step 2: Calculate the new fringe width for the new wavelength
The original wavelength (λ) is given as 6000 Å and the new wavelength (λ') is 5500 Å. The fringe width is directly proportional to the wavelength, so we can set up the following ratio:
ωω′=λλ′
Substituting the known values:
0.25mmω′=6000Å5500Å
Now, solving for ω':
ω′=0.25mm×55006000
ω′=0.25mm×1112
ω′=0.25mm×0.9167≈0.2292mm
Step 3: Calculate the position of the central maximum (zero order)
The position of the central maximum (y0) can be found using the position of the first order maximum (y1) and the original fringe width:
y0=y1−ω
y0=12.50mm−0.25mm=12.25mm
Step 4: Calculate the position of the tenth order maximum with the new fringe width
The position of the tenth order maximum (y10') can be calculated using the new fringe width:
y′10=y0+10×ω′
y′10=12.25mm+10×0.2292mm
y′10=12.25mm+2.292mm
y′10≈14.542mm
Final Answers:
- Position of the zero order maximum (y0) = 12.25 mm
- Position of the tenth order maximum (y10') ≈ 14.54 mm
Given:
- Position of the first order maximum (y1) = 12.50 mm
- Position of the tenth order maximum (y10) = 14.75 mm
y10−y1=14.75mm−12.50mm=2.25mm
10−1=9
ω=y10−y19=2.25mm9=0.25mm
The original wavelength (λ) is given as 6000 Å and the new wavelength (λ') is 5500 Å. The fringe width is directly proportional to the wavelength, so we can set up the following ratio:
ωω′=λλ′
0.25mmω′=6000Å5500Å
ω′=0.25mm×55006000
ω′=0.25mm×1112
ω′=0.25mm×0.9167≈0.2292mm
The position of the central maximum (y0) can be found using the position of the first order maximum (y1) and the original fringe width:
y0=y1−ω
y0=12.50mm−0.25mm=12.25mm
The position of the tenth order maximum (y10') can be calculated using the new fringe width:
y′10=y0+10×ω′
y′10=12.25mm+10×0.2292mm
y′10=12.25mm+2.292mm
y′10≈14.542mm
- Position of the zero order maximum (y0) = 12.25 mm
- Position of the tenth order maximum (y10') ≈ 14.54 mm
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Exercise 32.1
3 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Exercise 32.2
6 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Example Type 6
3 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
Similar Questions
In a double slit experiment, the distance between the slits is 5.0 mm and the slits are 1.0m from the screen. Two interference patterns can be seen on the screen one due to light with wavelength 480nm, and the other due to light with wavelength 600nm. What is the separation on the screen between the third order bright fringes of the two intergerence patterns?
View SolutionA parallel beam of light of all wavelength greater than 3000Å falls on a double slit in a Young's double slit experiment. It is observed that the wavelength 3600Å and 6000Å are absent at a distance of 31.5 mm from the position of the centre maximum and the orders of the interference at this point for the two wavelength differ by 7. If the distance between the slit and the screen in 1m, the separation between the two slits is
View SolutionA young's double slit apporatus is immersed in a liquid of refractive index 1.33.It has slit separation of 1 mm and interference pattern is observed on the screen at a distance 1.33 m from plane of slits.The wavelength in air is 6300Å
Find the distance of seventh bright fringe from third bright fringe lying on the same side of central bright fringe.
View SolutionLight of wavelength 6000Å is incident on a slit of width 0.30 mm. The screen is placed 2 m from the slit. Find (a) the position of the first dark fringe and (b). The width of the central bright fringe.
View SolutionIn the YDSE, the monochromatic source of wavelength λ is placed at a distance d2 from the central axis (as shown in the figure), where d is the separation between the two slits S1andS2 .
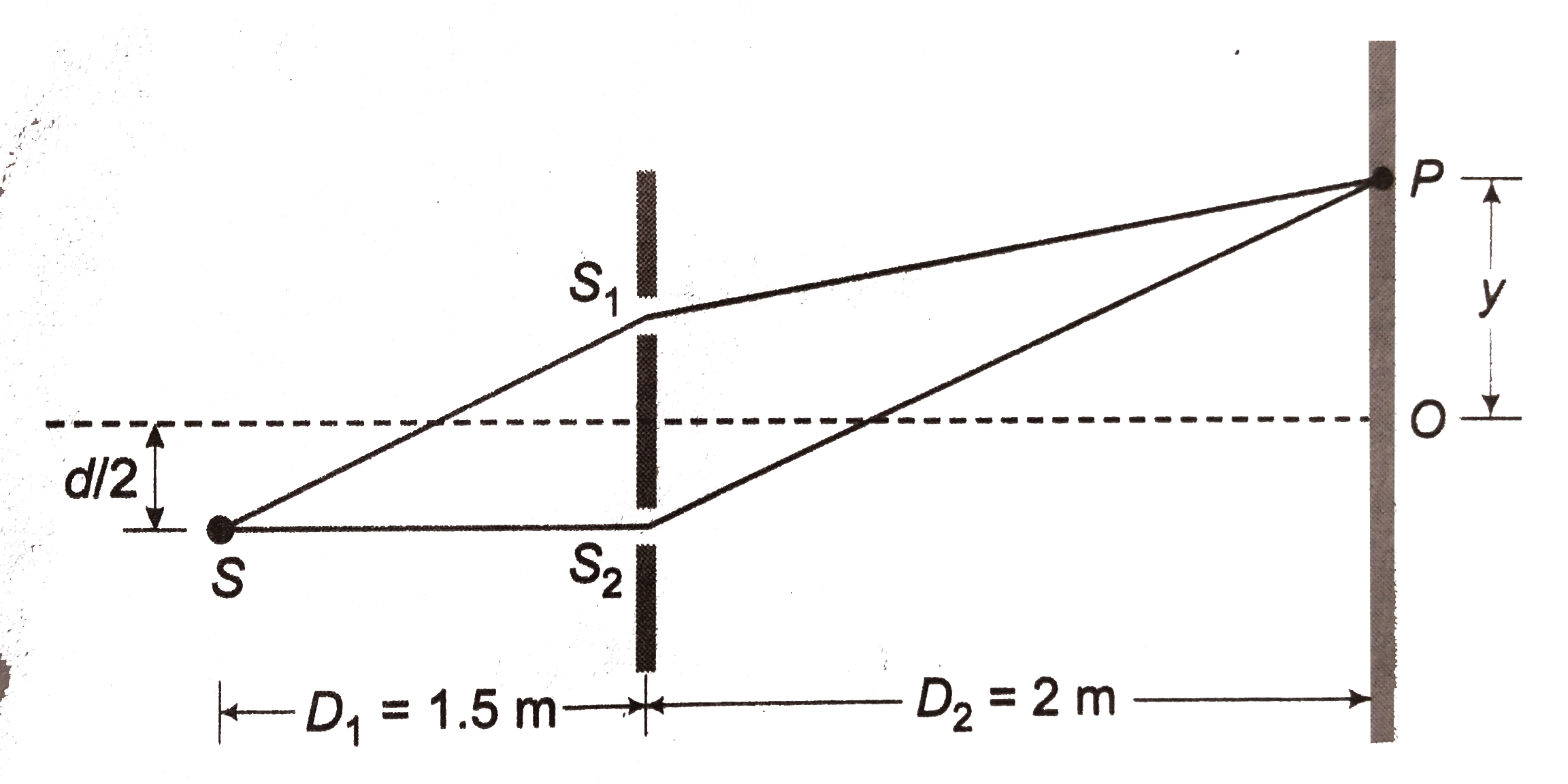
(a)Find the position of the central maxima.
(b) Find the order of interference formed at O.
(c)Now, S is placed on centre dotted line. Find the minimum thickness of the film of refractive indes μ=1.5 to be placed in front of S2 so that intensity at O becomes 34th of the maximum intensity.
(Take λ=6000Å,d=6mm.)
View Solution(a) Describe any two characteristic features which distinguish interference and diffraction phenomenon. Derive the expression for the intensity at a point of the interference pattern in Young.s double slit experiment.
(b) In the diffraction due to a simple slit experiment, the aperture of the slit is 3 mm. If monochromatic of wavelength 620 nm is incident normally on the slit, calculate the separation between the first order minima and the third order maxima on one side of the screen. The distance between the slit and the screen is 1.5 m.
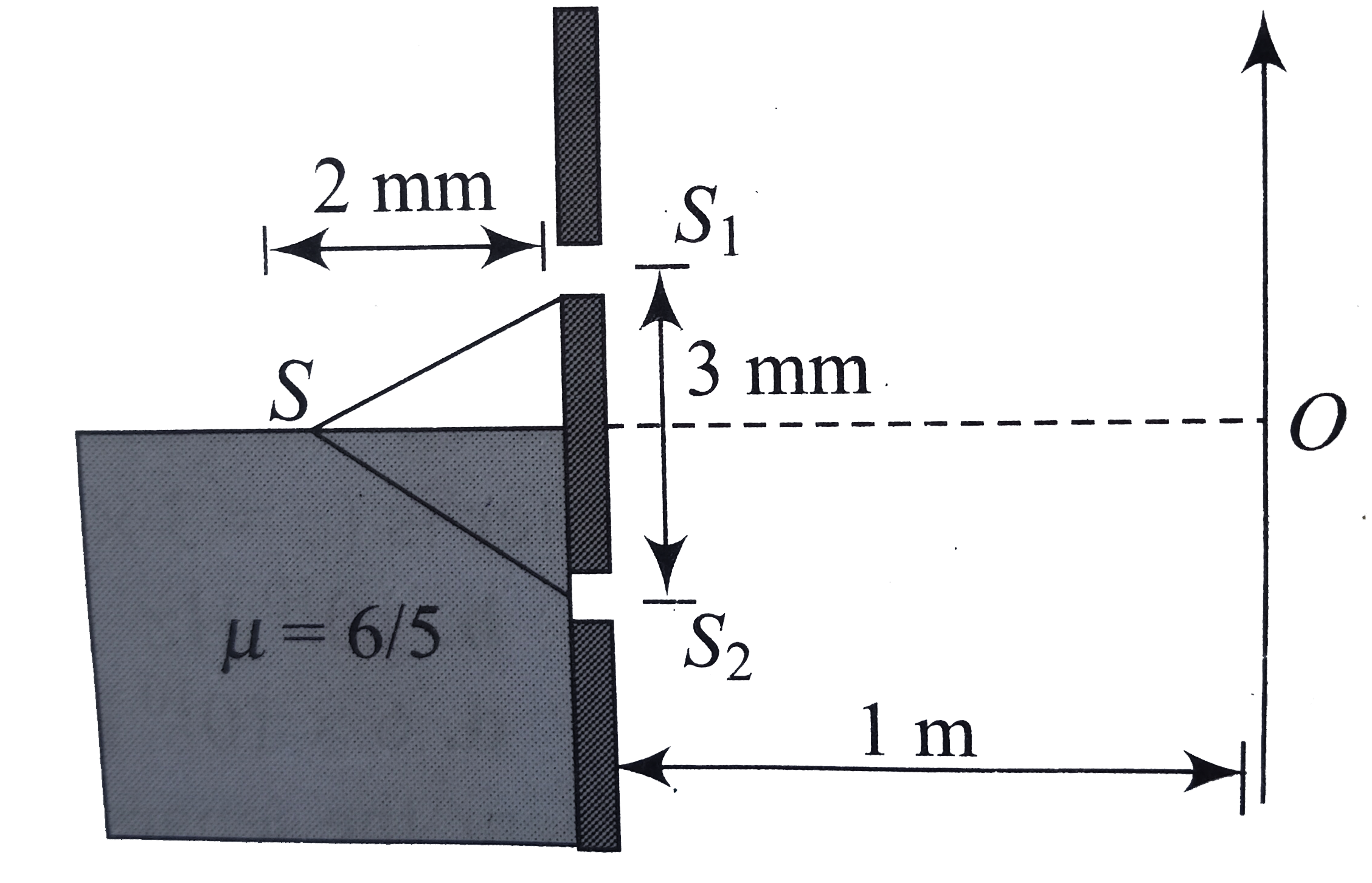
View SolutionIn Young's double-slit experiment, a point source is placed on a solid slab of refractive index 6/5 at a distance of 2 mm from two slits spaced 3 mm apart as shown and at equal distacne from both the slits. The screen is at a distance of 1 m from the slits. Wavelength of light used is 500 nm.
a. Find the position of the central maximum.
b. Find the order of the fringe formed at O.
c. A film of refractive index 1.8 is to be placed in front of S1 so that central maxima is formed where 200th maxima was formed. Find the thickness of film.
View SolutionA Lloyd's mirror or length 5 cm is illuminated with monochromatic light of wavelength λ(=6000Å) from a narrow 1 mm slit in its plane and 5 cm plane from its near edge. Find the fringe width on a screen 120 cm from the slit and width of interference pattern on the screen.

View SolutionCoherent light with wavelength 600nm passes through two very narrow slits and the interference pattern is observed on a screen 3.00 m from the slits. The first order bright fringe is at 4.94 mm from the centre of the central bright fringe. For what wavelength of light will the first order dark fringe be observed at this same point on the screen?
View SolutionIn Young's experiment, light of wavelength 600 nm falls on the double slits separated by 0.1 mm. What is the highest order of maximum intensity in the interference pattern obtained on a screen kept 3 m from the slits? How does the highest order change if the distance of screen from the slits is changed?
View Solution
