Comprehension # 5
One particle of mass 1kg is moving along positive x-axis with velocity 3m/s. Another particle of mass 2kg is moving along y-axis with 6m/s. At time t=0,1kg mass is at (3m,0) and 2kg at (0,9m),x−y plane is the horizontal plane. (Surface is rough for question, if cofficient of friction is 0.2 in both axis)
Co-ordinates of center of mass where it will stop finally are :-
(2.0 m,14.25 m)
(2.25 m,10 m)
(3.75 m,9 m)
(1.75 m,12 m)
To solve the problem step by step, we will follow these steps:
Step 1: Determine Initial Positions and Velocities
We have two particles:
- Particle A (mass m1=1kg) is at position (3m,0) with velocity →v1=(3m/s,0).
- Particle B (mass m2=2kg) is at position (0,9m) with velocity →v2=(0,6m/s).
Step 2: Calculate the Initial Center of Mass
The center of mass →RCM can be calculated using the formula:
→RCM=m1→r1+m2→r2m1+m2
Substituting the values:
→RCM=1⋅(3^i+0^j)+2⋅(0^i+9^j)1+2=(3^i+18^j)3=(1^i+6^j)m
Step 3: Determine the Forces Acting on the Particles
The only force acting on the particles is the frictional force, which can be calculated as:
Fr=μ⋅N
Where μ=0.2 and N=mg. For particle A:
Fr1=μm1g=0.2⋅1⋅10=2N
For particle B:
Fr2=μm2g=0.2⋅2⋅10=4N
Step 4: Calculate the Acceleration Due to Friction
Using Newton's second law F=ma:
- For particle A:
a1=−Fr1m1=−21=−2m/s2
- For particle B:
a2=−Fr2m2=−42=−2m/s2
Step 5: Calculate the Time Until Each Particle Stops
Using the equation v=u+at:
- For particle A:
0=3−2t⟹t1=32=1.5s
- For particle B:
0=6−2t⟹t2=62=3s
Step 6: Calculate the Distance Each Particle Travels
Using the equation s=ut+12at2:
- For particle A:
s1=3(1.5)+12(−2)(1.5)2=4.5−1.5=3m
- For particle B:
s2=6(3)+12(−2)(3)2=18−9=9m
Step 7: Calculate Final Positions of Each Particle
- Final position of particle A:
→r1=(3+3,0)=(6,0)m
- Final position of particle B:
→r2=(0,9+9)=(0,18)m
Step 8: Calculate the Final Center of Mass
Using the same formula for center of mass:
→RCM=1⋅(6^i+0^j)+2⋅(0^i+18^j)1+2=(6^i+36^j)3=(2^i+12^j)m
Final Answer
The coordinates of the center of mass where it will stop finally are:
(2,12)
We have two particles:
- Particle A (mass m1=1kg) is at position (3m,0) with velocity →v1=(3m/s,0).
- Particle B (mass m2=2kg) is at position (0,9m) with velocity →v2=(0,6m/s).
The center of mass →RCM can be calculated using the formula:
→RCM=m1→r1+m2→r2m1+m2
Substituting the values:
→RCM=1⋅(3^i+0^j)+2⋅(0^i+9^j)1+2=(3^i+18^j)3=(1^i+6^j)m
The only force acting on the particles is the frictional force, which can be calculated as:
Fr=μ⋅N
Where μ=0.2 and N=mg. For particle A:
Fr1=μm1g=0.2⋅1⋅10=2N
For particle B:
Fr2=μm2g=0.2⋅2⋅10=4N
Using Newton's second law F=ma:
- For particle A:
a1=−Fr1m1=−21=−2m/s2
- For particle B:
a2=−Fr2m2=−42=−2m/s2
Using the equation v=u+at:
- For particle A:
0=3−2t⟹t1=32=1.5s
- For particle B:
0=6−2t⟹t2=62=3s
Using the equation s=ut+12at2:
- For particle A:
s1=3(1.5)+12(−2)(1.5)2=4.5−1.5=3m
- For particle B:
s2=6(3)+12(−2)(3)2=18−9=9m
- Final position of particle A:
→r1=(3+3,0)=(6,0)m
- Final position of particle B:
→r2=(0,9+9)=(0,18)m
Using the same formula for center of mass:
→RCM=1⋅(6^i+0^j)+2⋅(0^i+18^j)1+2=(6^i+36^j)3=(2^i+12^j)m
The coordinates of the center of mass where it will stop finally are:
(2,12)
Similar Questions
Comprehension # 5
One particle of mass 1kg is moving along positive x-axis with velocity 3m/s. Another particle of mass 2kg is moving along y-axis with 6m/s. At time t=0,1kg mass is at (3m,0) and 2kg at (0,9m),x−y plane is the horizontal plane. (Surface is smooth for question 1 and rough for question 2 and 3)
The centre of mass of the two particles is moving in a straight line for which equation is :
View SolutionComprehension # 5
One particle of mass 1kg is moving along positive x-axis with velocity 3m/s. Another particle of mass 2kg is moving along y-axis with 6m/s. At time t=0,1kg mass is at (3m,0) and 2kg at (0,9m),x−y plane is the horizontal plane. (Surface is smooth for question 1 and rough for question 2 and 3)
If both the particles have the same value of coefficient of friction μ=0.2. The centre of mass will stop at time t= ..........s :-
View SolutionThe position (X)-time (t) graph for a particle of mass 1 kg moving along x-axis is shown in figure. Find impulse on particle at t = 4 s.
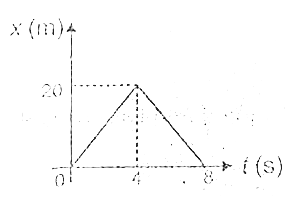
View SolutionA block of mass 1kg is at x=10m and moving towards negative x−aξs with velocity 6m/s. Another block of mass 2kg is at x=12m and moving towards positive x−axis with velocity 4m/s at the same instant. Find position of their centre of mass after 2s.
View SolutionTwo particles of masses 1kg and 2kg are located at x=0 and x=3m. Find the position of their centre of mass.
View SolutionTwo particles of masses 1kg and 2kg are located at x=0 and x=3m. Find the position of their centre of mass.
View SolutionA ball of mass 1kg moving with 4m−1 along +x-axis collides elastically with an another ball of mass 2kg moving with 6m/s is opposite direction. Find their velocities after collision.
View SolutionA time varying force, F=2t is acting on a particle of mass 2kg moving along x-axis. velocity of the particle is 4m/s along negative x-axis at time t=0. Find the velocity of the particle at the end of 4s.
View SolutionA particle of mass 3 kg is moving along x - axis and its position at time t is given by equation x=(2t2+5)m. Work done by all the force acting on it in time interval t=0 to t=3s is
View SolutionA particle of mass 2kg located at the position (ˆi+ˆj)m has velocity 2(ˆi−ˆj+ˆk)m/s . Its angular momentum about Z-axis in kgm2/s is
View Solution
