A string of length 0.4m and mass 10−2kg is tightly clamped at its ends. The tension in the string is 1.6N. Identical wave pulse are produced at one end at equal intervals of time, Δt. The minimum value of Δt which allows constructive interference of successive pulse is
0.05 s
0.10 s
0.20 s
0.40 s
The correct Answer is:B
To solve the problem step by step, we will follow these steps:
Step 1: Calculate the mass per unit length (μ) of the string
The mass per unit length (μ) is calculated using the formula:
μ=mL
where m=10−2kg (mass of the string) and L=0.4m (length of the string).
μ=10−2kg0.4m=0.025kg/m
Step 2: Calculate the wave speed (v) on the string
The wave speed (v) on a string under tension can be calculated using the formula:
v=√Tμ
where T=1.6N (tension in the string).
Substituting the values:
v=√1.6N0.025kg/m=√64=8m/s
Step 3: Determine the wavelength (λ) for constructive interference
For constructive interference of successive pulses, the path difference must be a multiple of the wavelength (λ). The minimum path difference for constructive interference occurs when it is equal to the wavelength.
In the fundamental mode, the wavelength (λ) can be related to the length of the string (L) as:
λ=2Ln
where n is the harmonic number. For the fundamental frequency, n=1:
λ=2L=2×0.4m=0.8m
Step 4: Relate the time interval (Δt) to the wave speed and wavelength
The time interval (Δt) between successive pulses can be related to the wave speed and wavelength by:
Δt=λv
Substituting the values of λ and v:
Δt=0.8m8m/s=0.1s
Final Answer
The minimum value of Δt which allows constructive interference of successive pulses is:
Δt=0.1s
---
The mass per unit length (μ) is calculated using the formula:
μ=mL
where m=10−2kg (mass of the string) and L=0.4m (length of the string).
The wave speed (v) on a string under tension can be calculated using the formula:
v=√Tμ
where T=1.6N (tension in the string).
v=√1.6N0.025kg/m=√64=8m/s
For constructive interference of successive pulses, the path difference must be a multiple of the wavelength (λ). The minimum path difference for constructive interference occurs when it is equal to the wavelength.
λ=2Ln
where n is the harmonic number. For the fundamental frequency, n=1:
λ=2L=2×0.4m=0.8m
The time interval (Δt) between successive pulses can be related to the wave speed and wavelength by:
Δt=λv
Δt=0.8m8m/s=0.1s
The minimum value of Δt which allows constructive interference of successive pulses is:
Δt=0.1s
Topper's Solved these Questions
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - More Than One Option is Correct
23 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Comprehion Type Questions
20 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE MAINS
50 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
Similar Questions
A string of length 0.4 m and mass 10−2 kg is tightly clamped at its ends. The tension in the string is 1. 6 N. identical wave pulses are produced at one end at equal intervals of time Δt. The value of Δt which allows construction interference between successive pulses is
View SolutionA string of length L and mass M is lying on a horizontal table. A force F is applied at one of its ends. Tension in the string at a distance x from the end at which force is applied is
View Solution
Knowledge Check
A 1 kg stone at the end of 1 m long string is whirled in a vertical circle at constant speed of 4 m/sec. The tension in the string is 6 N when the stone is at (g=10m/sec2)
Atop of the cirlceBbottom of the circleChalf way downDnone of the above
A string of length 1 m and mass 5 g is fixed at both ends. The tension in the string is 8.0 N. The string is set into vibration using an external vibrator of frequency 100 Hz. The separation between successive nodes on the string is close to :
View SolutionA string of mass per unit length μ=6×10−3 kg/m is fixed at both ends under the tension 540 N. If the string is in resonance with consecutive frequencies 420 Hz and 490 Hz. Then find the length of the string?
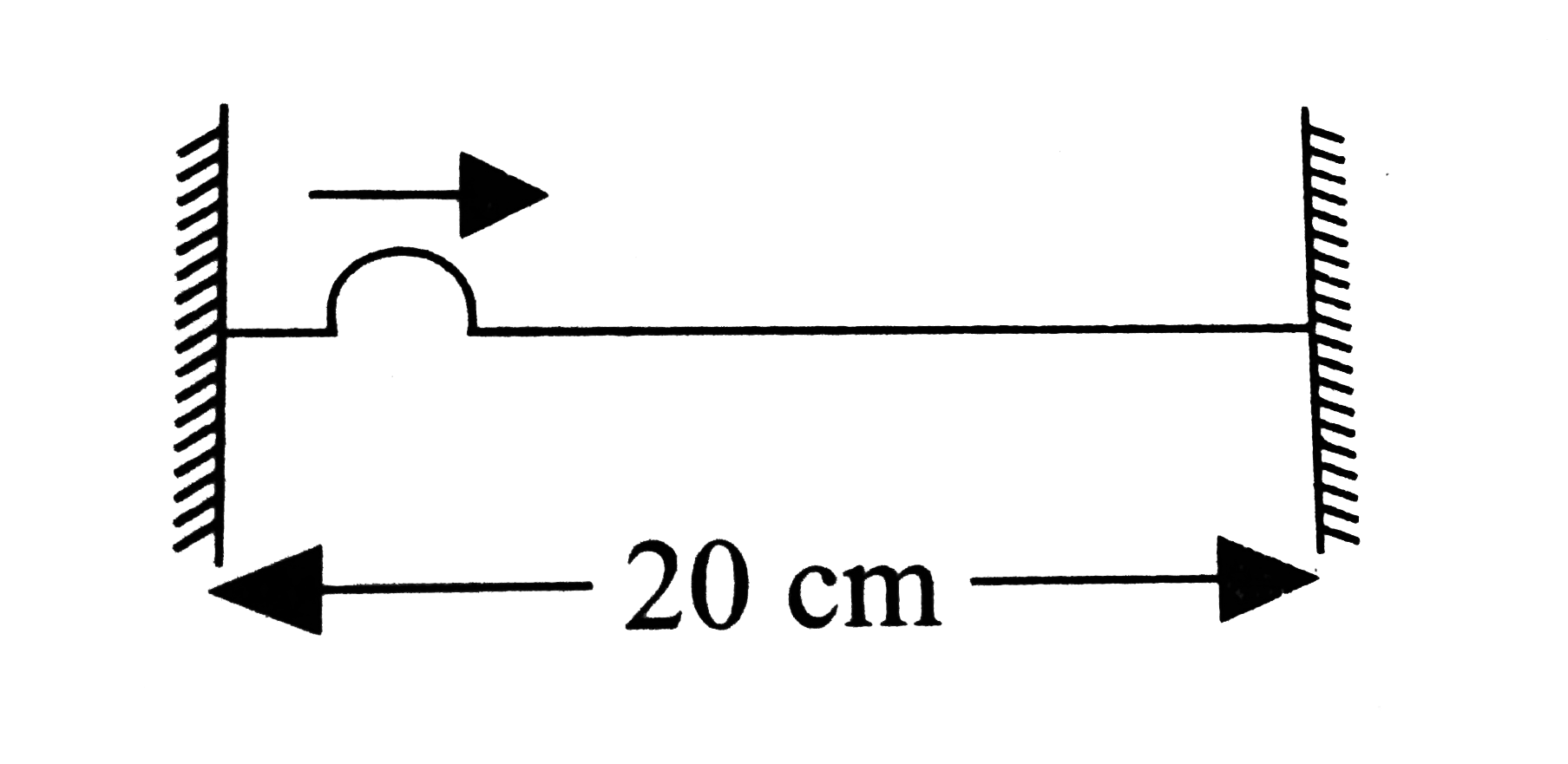
View SolutionA string of length 20cm and linear mass density 0.40g/cm is fixed at both ends and is kept under a tension of 16N.A wave pulse is produced at t=0 nearj an end as shown in figure which travels towards the other end.
when will the string have the shape shown in the figure again? (∈×10−2s)
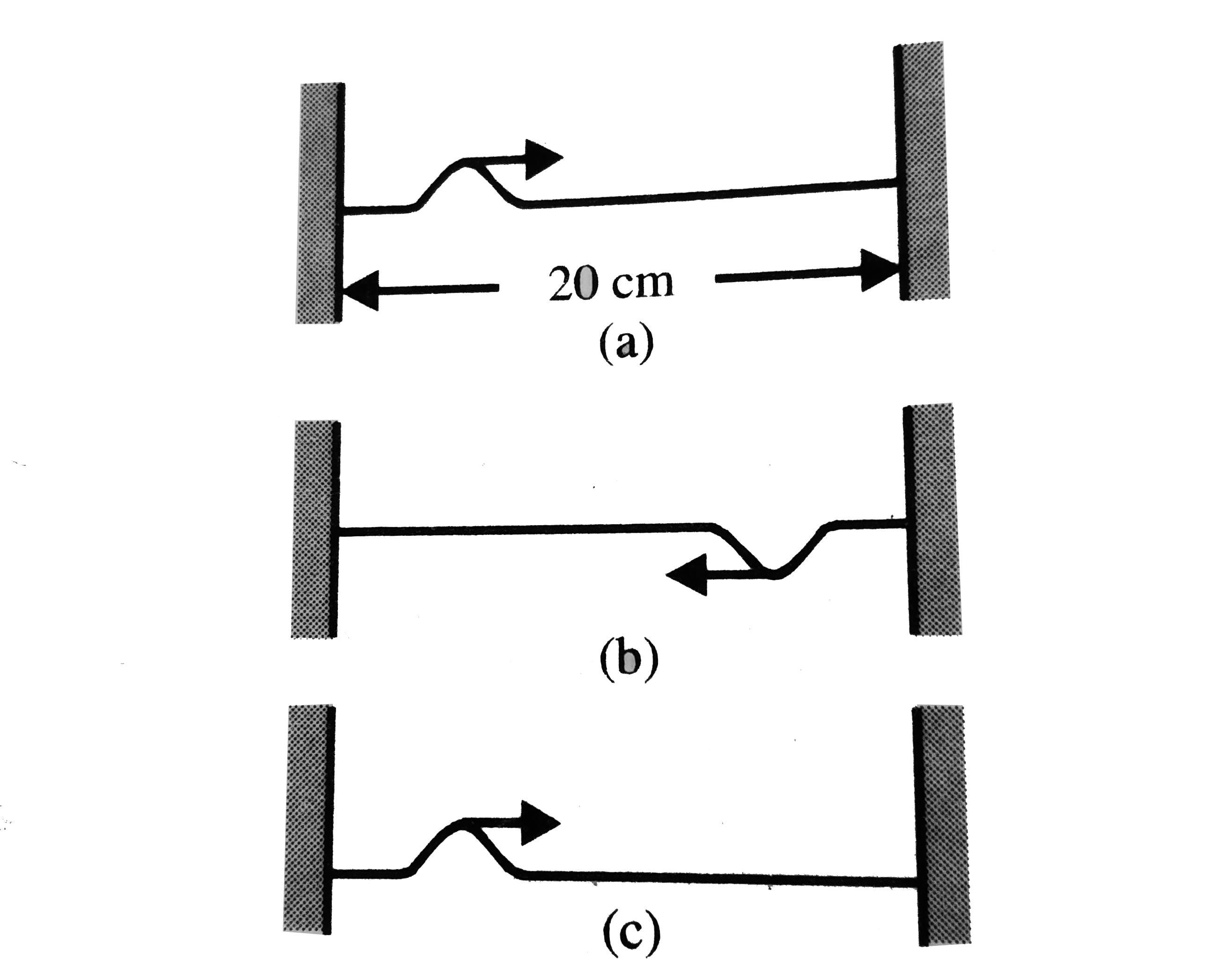
View SolutionA string of length 20cm and linear mass density 0.40g/cm is fixed at both ends and is kept under a tension of 16N . A wave pulse is produced at t=0 near an ends as shown in Fig. 7.17 (b) , which travels towards the other end . When will the string have the shape shown in the Fig . ( c).
View SolutionA string of length 50cm and mass 12.5g is fixed at both ends. A pipe closed at one end has a length 85cm. When the string vibrates in its second overtone and the air column in the pipe in its first. Overtone, they produce a beats frquency of 6Hz. It is also observed that decreasing teh tension in the string decreaes beats frequency. Neglect the end correction in the pipe and velocity of sound in air is 340m/s. then the tension in the string is
View SolutionA uniform rod of length 1m and mass 2kg is suspended. Calculate tension T (in N) in the string at the instant when the right string snaps (g=10m/s2).

View SolutionA string of length 40 cm and weighing 10 g is attached to a spring at one end and to a fixed wall at the other end. The spring has a spring constant of 160Nm−1 and is stretched by 1.0 cm. If a wave pulse is produced on the string near the wall, how much time will it take to reach the spring ?
View Solution
