The plane wave represented by an eqution of the form y = f(x-vt) implies the propagation along the positive x-axis without chang of shape with constant velocity v. Then
∂y∂t=−v(∂y∂x)
∂y∂t=−v(∂2y∂x2)
∂2y∂t2=−v2(∂2y∂x2)
∂2y∂t2=v2(∂2y∂x2)
The correct Answer is:A, C
To solve the problem regarding the plane wave represented by the equation y=f(x−vt), we will analyze the wave properties and derive the relationships between the derivatives of the wave function.
1. Understanding the Wave Equation:
The equation y=f(x−vt) represents a wave traveling in the positive x-direction with a constant velocity v. The function f describes the shape of the wave, which does not change as it propagates.
2. Consider a Specific Wave Function:
Let's take a specific form of the wave function, such as:
y=Asin(ωt−kx)
where A is the amplitude, ω is the angular frequency, and k is the wave number.
3. Calculate the First Derivative with Respect to Time:
We need to find dydt:
dydt=Aωcos(ωt−kx)
4. Calculate the First Derivative with Respect to Position:
Next, we calculate dydx:
dydx=−Akcos(ωt−kx)
5. Relate the Derivatives:
Now, we can relate dydt to dydx:
dydt=−ωkdydx
We know that v=ωk, so we can rewrite this as:
dydt=−vdydx
6. Calculate the Second Derivative with Respect to Time:
We find d2ydt2:
d2ydt2=−Aω2sin(ωt−kx)
This can be expressed in terms of y:
d2ydt2=−ω2y
7. Calculate the Second Derivative with Respect to Position:
Now, we calculate d2ydx2:
d2ydx2=−Ak2sin(ωt−kx)
This can also be expressed in terms of y:
d2ydx2=−k2y
8. Relate the Second Derivatives:
We can relate the second derivative with respect to time to the second derivative with respect to position:
d2ydt2=−ω2k2d2ydx2
Since v2=ω2k2, we have:
d2ydt2=−v2d2ydx2
Conclusion:
From the analysis, we can conclude that:
- Option A is correct: dydt=−vdydx
- Option C is correct: d2ydt2=−v2d2ydx2
The equation y=f(x−vt) represents a wave traveling in the positive x-direction with a constant velocity v. The function f describes the shape of the wave, which does not change as it propagates.
Let's take a specific form of the wave function, such as:
y=Asin(ωt−kx)
where A is the amplitude, ω is the angular frequency, and k is the wave number.
We need to find dydt:
dydt=Aωcos(ωt−kx)
Next, we calculate dydx:
dydx=−Akcos(ωt−kx)
Now, we can relate dydt to dydx:
dydt=−ωkdydx
We know that v=ωk, so we can rewrite this as:
dydt=−vdydx
We find d2ydt2:
d2ydt2=−Aω2sin(ωt−kx)
This can be expressed in terms of y:
d2ydt2=−ω2y
Now, we calculate d2ydx2:
d2ydx2=−Ak2sin(ωt−kx)
This can also be expressed in terms of y:
d2ydx2=−k2y
We can relate the second derivative with respect to time to the second derivative with respect to position:
d2ydt2=−ω2k2d2ydx2
Since v2=ω2k2, we have:
d2ydt2=−v2d2ydx2
From the analysis, we can conclude that:
- Option A is correct: dydt=−vdydx
- Option C is correct: d2ydt2=−v2d2ydx2
Topper's Solved these Questions
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Comprehion Type Questions
20 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Matrix Matching
16 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - ONLY ONE OPTION IS CORRECT
62 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
Similar Questions
Knowledge Check
A transverse wave is represented by the equation
y=y0sin(2π)λ(vt−x)
For what value of λ is the particle velocity equal to two time the wave velocity ?Aλ=2πy0Bλ=2πy0/3Cλ=πy0/2Dλ=πy0
A wave is represented by the equation y=0.5sin(10t−x)m. It is a travelling wave propagating along the + x direction with velocity
View SolutionA wavefront is represented by the plane y=3−x. The propagation of wave takes place at
View SolutionAn ant travels along a long rod with a constant velocity ii relative to the rod starting from the origin. The rod is kept initially along the positive x-axis. At t = 0, the rod also starts rotating with an angular velocity w (anticlockwise) in x-y plane about origin. Then
View SolutionA wave front is represented by the plane y=5−x Find the direction of propagation of wave.
View SolutionA force F=−K(yˆi+xˆj) (where K is a positive constant) acts on a particle moving in the x-y plane. Starting from the origin, the particle is taken along the positive x-axis to the point (a,0), and then parallel to the y-axis to the point (a,a). The total work done by the force F on the particle is
View SolutionA wave is represented by an eqution y=c1sin(c2x+c3t). In which direction is the wave going? Assume that c1,c2andc3 are all positive.
View SolutionA disc of radius R rolls without slipping at speed v along positive x-axis. Velocity of point P at the instant shown in Fig. is
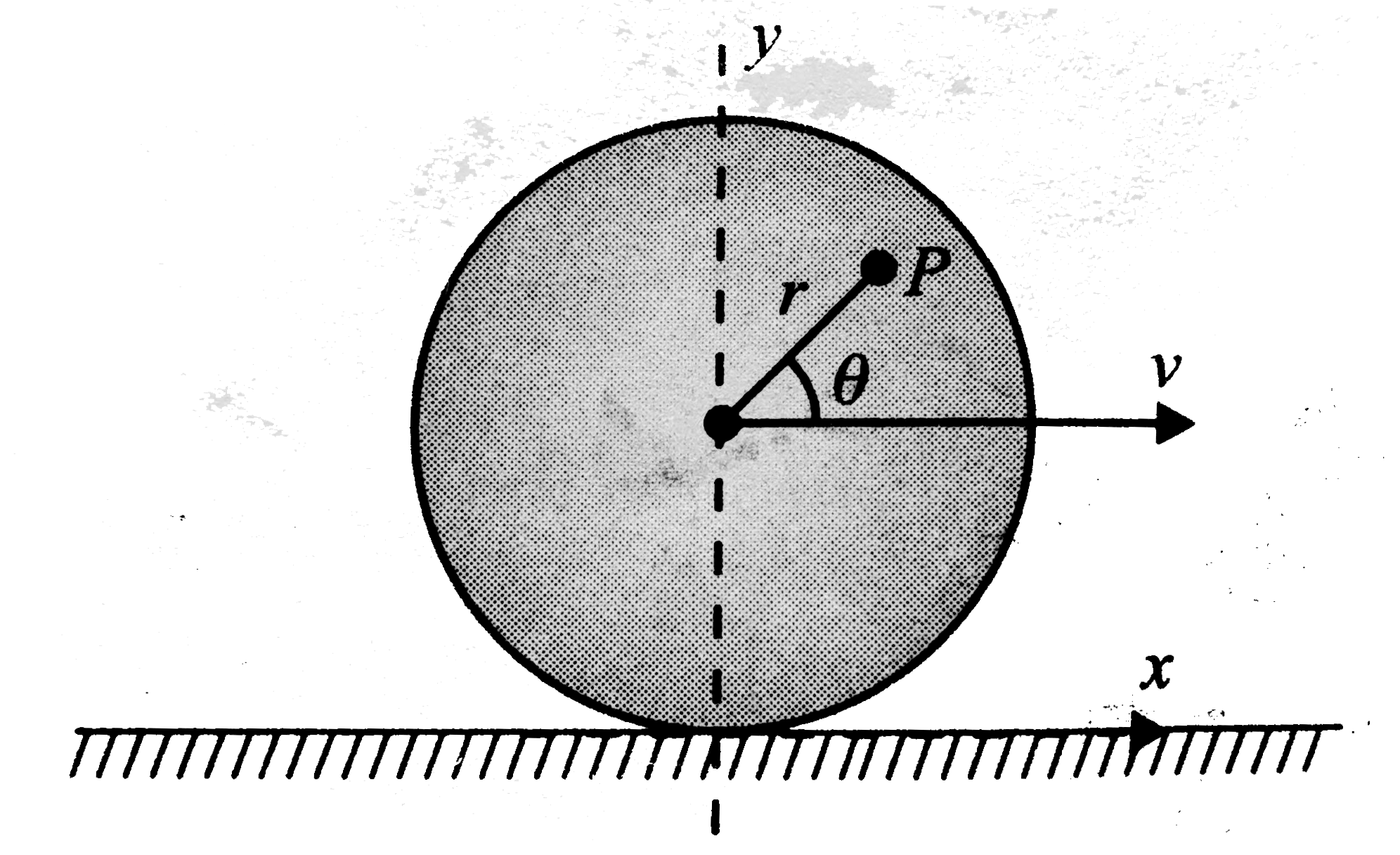
View Solution
