Focal length of a convex mirror is 10 cm
image of an object placed at 20 cm is also at 20 cm
image of an object placed at 10 cm is at infinity
both (a) and (b) are wrong
both (a) and (b) are correct
The correct Answer is:C
To solve the problem regarding the focal length of a convex mirror, we will analyze the given options step by step using the mirror formula.
Given:
- Focal length (F) of the convex mirror = +10 cm (positive because it is a convex mirror)
Mirror Formula:
The mirror formula is given by:
1f=1v+1u
Where:
- f = focal length of the mirror
- v = image distance (positive for virtual images in convex mirrors)
- u = object distance (negative for real objects in front of the mirror)
Analyzing the Options:
Option 1: Image of an object placed at 20 cm is also at 20 cm.
1. Object Distance (u): Since the object is placed at 20 cm, we take u=−20 cm.
2. Using the mirror formula:
1f=1v+1u
Substituting the values:
110=1v+1−20
Rearranging gives:
1v=110+120
Finding a common denominator (20):
1v=220+120=320
Therefore:
v=203 cm
This means the image distance is 203 cm, not 20 cm. Thus, Option 1 is incorrect.
Option 2: Image of an object placed at 10 cm is at infinity.
1. Object Distance (u): For an object placed at 10 cm, we take u=−10 cm.
2. Using the mirror formula:
1f=1v+1u
Substituting the values:
110=1v+1−10
Rearranging gives:
1v=110+110=210=15
Therefore:
v=5 cm
This means the image distance is 5 cm, not at infinity. Thus, Option 2 is also incorrect.
Conclusion:
Since both options A and B are incorrect, the correct answer is:
Option 3: Both A and B are wrong.
- Focal length (F) of the convex mirror = +10 cm (positive because it is a convex mirror)
The mirror formula is given by:
1f=1v+1u
Where:
- f = focal length of the mirror
- v = image distance (positive for virtual images in convex mirrors)
- u = object distance (negative for real objects in front of the mirror)
1. Object Distance (u): Since the object is placed at 20 cm, we take u=−20 cm.
2. Using the mirror formula:
1f=1v+1u
Substituting the values:
110=1v+1−20
Rearranging gives:
1v=110+120
Finding a common denominator (20):
1v=220+120=320
Therefore:
v=203 cm
This means the image distance is 203 cm, not 20 cm. Thus, Option 1 is incorrect.
1. Object Distance (u): For an object placed at 10 cm, we take u=−10 cm.
2. Using the mirror formula:
1f=1v+1u
Substituting the values:
110=1v+1−10
Rearranging gives:
1v=110+110=210=15
Therefore:
v=5 cm
This means the image distance is 5 cm, not at infinity. Thus, Option 2 is also incorrect.
Since both options A and B are incorrect, the correct answer is:
Option 3: Both A and B are wrong.
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - A. Only one option is correct (JEE Advance)
73 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - B. More than one option is correct
20 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
Similar Questions
The focal length of a convex mirror is 20 cm its radius of curvature will be
View SolutionThe focal length of a convex mirror is 40 cm. A point source of light is kept at distance 40 cm from the mirror. Find the distance of image from the mirror.
View SolutionThe focal length of a concave mirror is 10 cm. Find its radius of curvature.
View SolutionFocal length of convex mirror M1 is 20 cm and that of concave mirror M2 is 30 cm .Find position of second image I2.Take first reflection from M1.
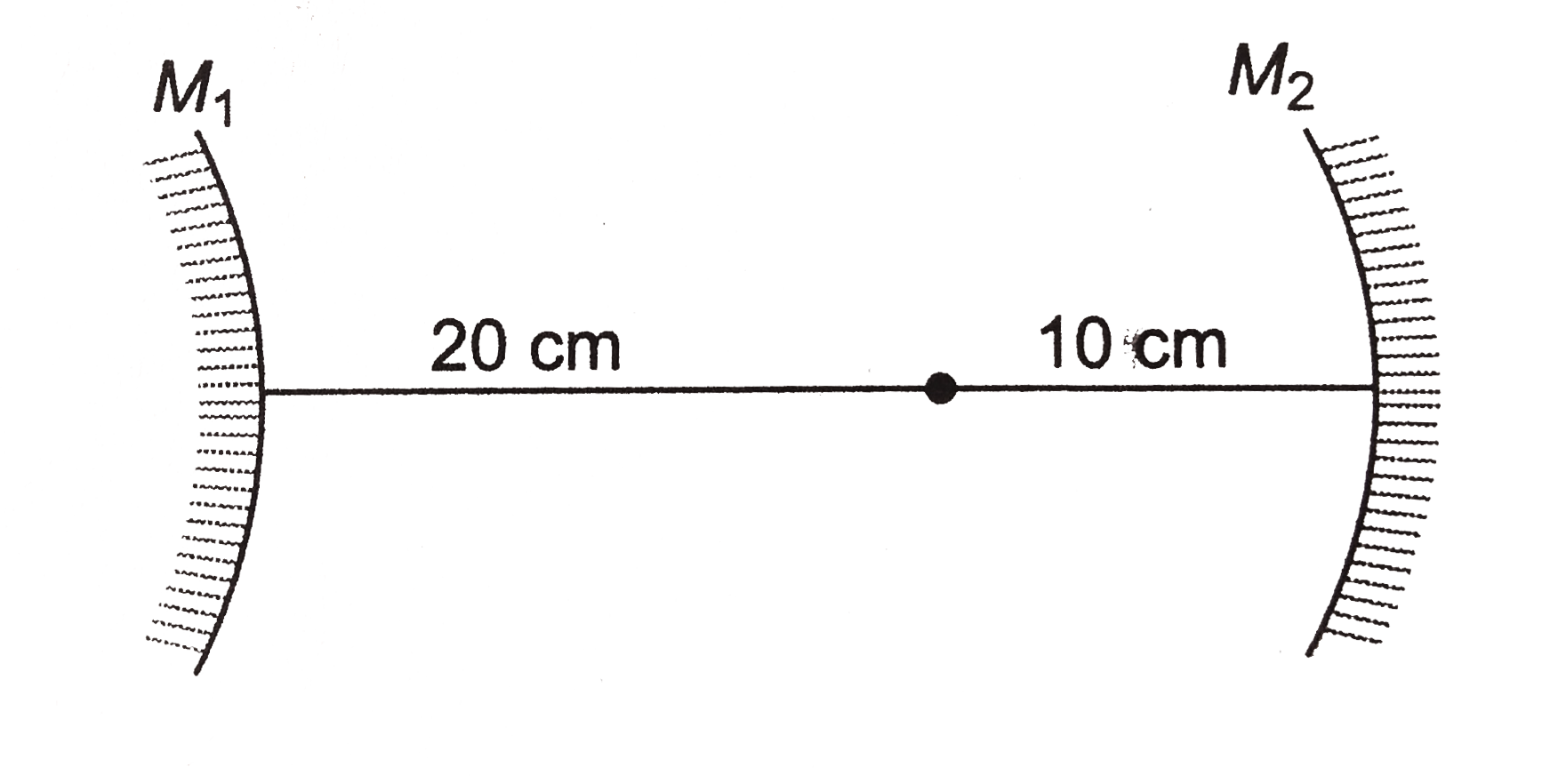
View SolutionThe focal length of a convex mirror is obtained by using a convex lens The following observations are recorded during theexperiment .
objectopsition=5cmlens=35.4cmImage=93.8cmMirror=63.3cmBencherror=−0.1cm
then the focal length of mirror will be .
View SolutionTo find the focal length of a convex mirror , a student records the following data:
Object PinConvex LensConvex mirrorImage Pin22.2cm32.2cm45.8cm71.2cm
The focal length of the convex lens is f1 and that of mirror is f2 . Then taking index correction to be negligibly small , f1 and f2 are close to :
View Solution(a) What is focal length of a convex mirror of radius of curvature 20cm?
(b) What is radius of curvature of a mirror of focal length −50cm ?
View SolutionA point object is placed at a distance of 12cm from a convex lens of focal length 10cm. On the other side of the lens, a convex mirror is placed at a distance of 10cm from the lens such that the image formed by the combination coincides with the object itself. The focal length of the convex mirror is
View SolutionAn object is placed at a distance of 15cm from a convex lenx of focal length 10cm. On the other side of the lens, a convex mirror is placed at its focus such that the image formed by the combination coincides with the object itself. The focal length of the convex mirror is
View SolutionIn an experiment for the determination of focal length of the convex mirror a convex lens of focal length 20cm is placed on the optical bench and an object pin is placed at a distance 30cm from the lens. When a convex mirror is introduced in between the lens and the real and inverted image of the object, the final image of object O is formed at O itself. If the distance between the lens and the mirror is 10cm, then the focal length of the mirror is
View Solution
