One of the refracting surfaces of a prism of angle of 30∘ is silvered. A ray of light incident at an angle of 60∘ retraces its path. The refractive index of the material of prism is
√2
√3
3/2
2
The correct Answer is:B
To find the refractive index of the prism, we can follow these steps:
Step 1: Understand the Geometry of the Prism
We have a prism with an apex angle A=30∘. One of its refracting surfaces is silvered, which means it acts as a mirror. A ray of light is incident on the prism at an angle of 60∘.
Step 2: Analyze the Path of the Ray
Since the ray retraces its path after reflecting off the silvered surface, we can conclude that the angle of incidence at the silvered surface must be such that the angle of refraction is 90∘. This means that the angle of incidence at the silvered surface must be equal to the angle of refraction.
Step 3: Determine the Angles
Let's denote:
- The angle of incidence at the first surface of the prism as i=60∘.
- The angle of refraction at the first surface as r.
- The angle of incidence at the silvered surface as r′.
Since the prism has an apex angle of 30∘, we can use the relationship:
r+r′=A=30∘
From the geometry, we know that if the ray retraces its path, then:
r′=90∘
Thus, we can find r:
r+90∘=30∘⟹r=30∘−90∘=−60∘
This indicates that the ray is actually refracted back at the silvered surface.
Step 4: Apply Snell's Law
Using Snell's law at the first surface of the prism:
sin(i)=μ⋅sin(r)
Substituting the known values:
sin(60∘)=μ⋅sin(30∘)
Using the values:
sin(60∘)=√32,sin(30∘)=12
We can substitute these into the equation:
√32=μ⋅12
Step 5: Solve for the Refractive Index μ
To find μ:
μ=√3/21/2=√3
Conclusion
The refractive index of the material of the prism is √3.
---
We have a prism with an apex angle A=30∘. One of its refracting surfaces is silvered, which means it acts as a mirror. A ray of light is incident on the prism at an angle of 60∘.
Since the ray retraces its path after reflecting off the silvered surface, we can conclude that the angle of incidence at the silvered surface must be such that the angle of refraction is 90∘. This means that the angle of incidence at the silvered surface must be equal to the angle of refraction.
Let's denote:
- The angle of incidence at the first surface of the prism as i=60∘.
- The angle of refraction at the first surface as r.
- The angle of incidence at the silvered surface as r′.
r+r′=A=30∘
From the geometry, we know that if the ray retraces its path, then:
r′=90∘
Thus, we can find r:
r+90∘=30∘⟹r=30∘−90∘=−60∘
This indicates that the ray is actually refracted back at the silvered surface.
Using Snell's law at the first surface of the prism:
sin(i)=μ⋅sin(r)
Substituting the known values:
sin(60∘)=μ⋅sin(30∘)
Using the values:
sin(60∘)=√32,sin(30∘)=12
We can substitute these into the equation:
√32=μ⋅12
Step 5: Solve for the Refractive Index μ
To find μ:
μ=√3/21/2=√3
The refractive index of the material of the prism is √3.
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - A. Only one option is correct (JEE Advance)
73 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - B. More than one option is correct
20 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
Similar Questions
A ray of light is incident on the face AB of a glass prism ABC having vertex anlge A equal to 30∘. The face AC is silvered and a ray of light incident on the face AB retraces its path. If the refractive index of the material of prism is √3 find the angle of incidence on the face AB.
View SolutionA ray of monochromatic light is incident on one refracting face of a prism of angle 75∘. It passes thorugh the prism and is incident on the other face at the critical angle. If the refractive index of the material of the prism is √2, the angle of incidence on the first face of the prism is
View SolutionIf the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
View SolutionA ray of light is incident at an angle of 60∘ on one face of a prism which has refracting angle of 30∘ . The ray emerging out of the prism makes an angle of 30∘ with the incident ray. If the refractive index of the material of the prism is μ=√a , find the value of a .
View SolutionFor a ray of light refracted through a prism of angle 60∘, the angle of incidence is equal to the angle of emergence, each equal to 45∘. Find the refractive index of the material of the prism.
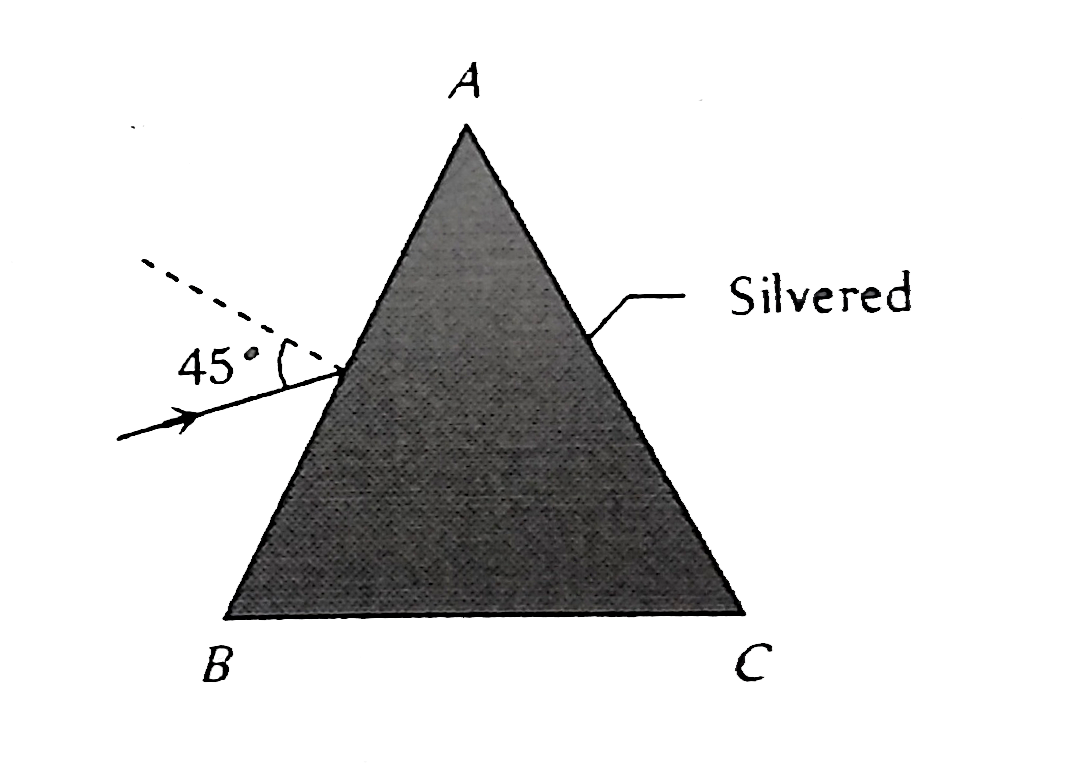
View SolutionA prism ABC of angle 30∘ has its face AC silvered. A ray of light incident at an angle of 45∘ at the face AB retraces its path after refraction at face AB and reflection at face AC . The refractive index of the material of the prism is
View SolutionA glass prism has refractive index √2 and refracting angle 30∘ . One of the refracting surface of the prism is silvered. A beam of monchromatic light will retrace it path it its angle of incidence on the unsilvered refracting surface of the prism is
View SolutionWhen a ray of light is incident normally on one refracting surface of an equilateral prism (Refractive index of the material of the prism =1.5)
View SolutionWhen a ray of light is incident normally on one refracting surface of an equilateral prism (Refractive index of the material of the prism =1.5
View SolutionA ray of light incident at an angle 90° with the face of an equilateral prism passes symmetrically. Calculate the refractive index of the material of the prism
View Solution
