A ray of light is incident on the face AB of a glass prism ABC having vertex anlge A equal to 30∘. The face AC is silvered and a ray of light incident on the face AB retraces its path. If the refractive index of the material of prism is √3 find the angle of incidence on the face AB.
60∘
30∘
45∘
25∘
The correct Answer is:C
To solve the problem, we need to find the angle of incidence on the face AB of a glass prism with a vertex angle A of 30 degrees, where the face AC is silvered, and the ray retraces its path. The refractive index of the prism is given as √3.
1. Understand the Geometry of the Prism:
- The prism has a vertex angle A = 30°.
- The ray of light is incident on face AB and retraces its path after reflecting off the silvered face AC.
2. Identify the Angles:
- Let the angle of incidence on face AB be denoted as I.
- When the ray refracts at face AB, it will have an angle of refraction R.
- Since the face AC is silvered, the ray will reflect off this surface and retrace its path.
3. Use the Geometry of Reflection:
- When the ray retraces its path after reflecting off the silvered face, the angle of incidence at AC will be equal to the angle of reflection.
- The angle of incidence at AC can be calculated as follows:
- The total angle at point A is 180°.
- The angle at point A is divided into two parts: angle R (the angle of refraction at AB) and the angle of incidence at AC.
- Therefore, the angle at A can be expressed as:
180°=R+90°+(30°−R)
This simplifies to:
R+90°+30°−R=180°⟹90°+30°=180°
This confirms the angles are correct.
4. Apply Snell's Law:
- According to Snell's law:
n=sinIsinR
- Here, the refractive index (n) of the prism is given as √3, and R is the angle of refraction at face AB.
- Since the angle A is 30°, we can find R:
R=30°(because the ray retraces its path)
5. Substituting Values into Snell's Law:
- Substitute the known values into Snell's law:
√3=sinIsin30°
- Since sin30°=12, we have:
√3=sinI12⟹sinI=√3⋅12=√32
6. Finding the Angle of Incidence I:
- The angle I can be found using the inverse sine function:
I=sin−1(√32)
- This gives us:
I=60°
Final Answer:
The angle of incidence on the face AB is 60 degrees.
- The prism has a vertex angle A = 30°.
- The ray of light is incident on face AB and retraces its path after reflecting off the silvered face AC.
- Let the angle of incidence on face AB be denoted as I.
- When the ray refracts at face AB, it will have an angle of refraction R.
- Since the face AC is silvered, the ray will reflect off this surface and retrace its path.
- When the ray retraces its path after reflecting off the silvered face, the angle of incidence at AC will be equal to the angle of reflection.
- The angle of incidence at AC can be calculated as follows:
- The total angle at point A is 180°.
- The angle at point A is divided into two parts: angle R (the angle of refraction at AB) and the angle of incidence at AC.
- Therefore, the angle at A can be expressed as:
180°=R+90°+(30°−R)
This simplifies to:
R+90°+30°−R=180°⟹90°+30°=180°
This confirms the angles are correct.
- According to Snell's law:
n=sinIsinR
- Here, the refractive index (n) of the prism is given as √3, and R is the angle of refraction at face AB.
- Since the angle A is 30°, we can find R:
R=30°(because the ray retraces its path)
- Substitute the known values into Snell's law:
√3=sinIsin30°
- Since sin30°=12, we have:
√3=sinI12⟹sinI=√3⋅12=√32
- The angle I can be found using the inverse sine function:
I=sin−1(√32)
- This gives us:
I=60°
The angle of incidence on the face AB is 60 degrees.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - PRACTICE SHEET (ADVANCED) (MORE THAN ONE CORRECT TYPE QUESTIOS)
4 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - PRACTICE SHEET (ADVANCED) (LINKED COMPREHENSION CORRECT TYPE QUESTIOS)
7 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE -I (LEVEL-II LECTURE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
4 Videos
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
10 Videos
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE
106 Videos
Similar Questions
One of the refracting surfaces of a prism of angle of 30∘ is silvered. A ray of light incident at an angle of 60∘ retraces its path. The refractive index of the material of prism is
View SolutionA ray of monochromatic light is incident on one refracting face of a prism of angle 75∘. It passes thorugh the prism and is incident on the other face at the critical angle. If the refractive index of the material of the prism is √2, the angle of incidence on the first face of the prism is
View SolutionA ray of light is incident at an angle of 60∘ on one face of a prism which has refracting angle of 30∘ . The ray emerging out of the prism makes an angle of 30∘ with the incident ray. If the refractive index of the material of the prism is μ=√a , find the value of a .
View SolutionA ray of monochromatic light is incident on the refracting face of a prism (angle 75∘0 . It passes through the prism and is incident on the other face at the critical angle. If the refractive index of the prism is √2 , then the angle of incidence on the first of the prism is
View SolutionWhen a ray of light is incident normally on one refracting surface of an equilateral prism (Refractive index of the material of the prism =1.5
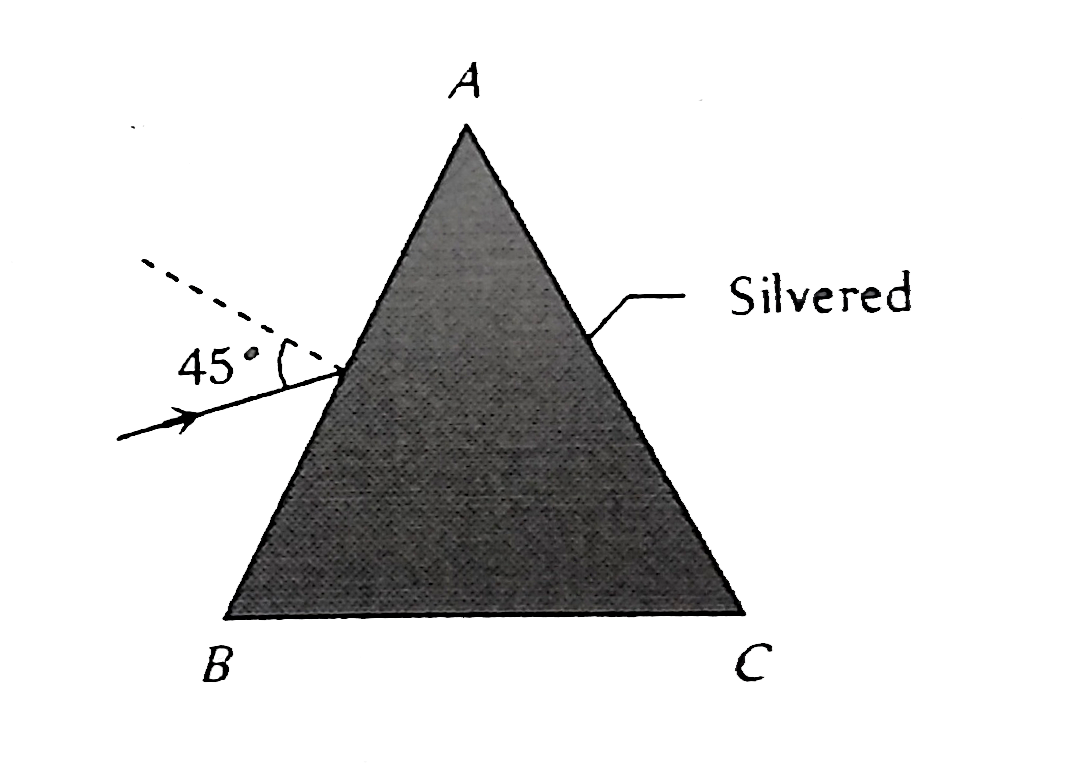
View SolutionA prism ABC of angle 30∘ has its face AC silvered. A ray of light incident at an angle of 45∘ at the face AB retraces its path after refraction at face AB and reflection at face AC . The refractive index of the material of the prism is
View SolutionA ray of light is normally incident on one face of an equilateral glass prism. Answer the following :
(a) What is the angle of incidence on the first face of the prism?
(b) What is the angle of refraction from the first face of the prism ?
(c) What will be the angle of incidence at the second face of the prism ?
(d) Will the light ray suffer minimum deviation by the prism ?
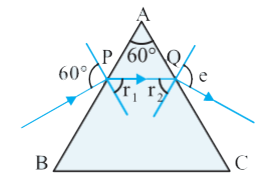
View SolutionA ray of light is incident on one face of a prism (m=√3) at an angle of 60∘. The refracting angle of the prism is also 60∘. Find the angle of emergence .
View SolutionA ray of light is incident on one face of a transparent slab of thickness 15 cm. The angle of incidence is 60∘. If the lateral displacement of the ray on emerging from the parallel plane is 5√3 cm, the refractive index of the material of the slab is
View SolutionA ray OP of monochromatic light is incident on the face AB of prism ABCD mear vertex B at an incident angle of 60degree (see figure). If the refractive index of the material of the prism is √3, which of the following is (are) are correct?

View Solution
