A capacitor is filled with an insulator and a certain potential difference is applied to its pltaes. The energy stored in the capacitor is . Now the capacitor is disconnected from the source and the insulator is pulled out of the capacitor. The work performed against the forces of electric field in pulling out the insulator is . Then dielectric constant of the insulator is.
4
8
5
3
The correct Answer is:C
To solve the problem, we will follow these steps:
Step 1: Understand the initial conditions
When a capacitor is filled with a dielectric material, the capacitance increases. The capacitance C of a capacitor filled with a dielectric is given by:
C=K⋅C0
where K is the dielectric constant and C0 is the capacitance without the dielectric.
Step 2: Write the expression for energy stored in the capacitor
The energy U stored in the capacitor is given by:
U=12CV2
Substituting for C:
U=12(K⋅C0)V2
Step 3: Disconnect the capacitor from the source
When the capacitor is disconnected from the voltage source, the charge Q on the capacitor remains constant. The charge Q can be expressed as:
Q=C⋅V=K⋅C0⋅V
Step 4: Determine the final potential energy after removing the dielectric
When the dielectric is removed, the capacitance of the capacitor becomes C0. The final potential energy Uf can be expressed as:
Uf=Q22C0
Substituting Q:
Uf=(K⋅C0⋅V)22C0=K2⋅C20⋅V22C0=K2⋅C0⋅V22
Step 5: Relate the initial and final potential energies
From the initial energy expression, we have:
U=12KC0V2
Thus, we can relate the final potential energy to the initial potential energy:
Uf=K⋅U
Step 6: Calculate the work done in removing the dielectric
The work done W against the electric field when the dielectric is removed is given as:
W=Uf−U
Substituting for Uf:
W=K⋅U−U=(K−1)⋅U
According to the problem, the work done is 4U:
(K−1)⋅U=4U
Step 7: Solve for the dielectric constant K
Dividing both sides by U (assuming U≠0):
K−1=4
Thus,
K=5
Conclusion
The dielectric constant of the insulator is K=5.
When a capacitor is filled with a dielectric material, the capacitance increases. The capacitance C of a capacitor filled with a dielectric is given by:
C=K⋅C0
where K is the dielectric constant and C0 is the capacitance without the dielectric.
The energy U stored in the capacitor is given by:
U=12CV2
Substituting for C:
U=12(K⋅C0)V2
When the capacitor is disconnected from the voltage source, the charge Q on the capacitor remains constant. The charge Q can be expressed as:
Q=C⋅V=K⋅C0⋅V
When the dielectric is removed, the capacitance of the capacitor becomes C0. The final potential energy Uf can be expressed as:
Uf=Q22C0
Substituting Q:
Uf=(K⋅C0⋅V)22C0=K2⋅C20⋅V22C0=K2⋅C0⋅V22
From the initial energy expression, we have:
U=12KC0V2
Thus, we can relate the final potential energy to the initial potential energy:
Uf=K⋅U
The work done W against the electric field when the dielectric is removed is given as:
W=Uf−U
Substituting for Uf:
W=K⋅U−U=(K−1)⋅U
According to the problem, the work done is 4U:
(K−1)⋅U=4U
Dividing both sides by U (assuming U≠0):
K−1=4
Thus,
K=5
The dielectric constant of the insulator is K=5.
Topper's Solved these Questions
- ELECTROSTATICSBook:DC PANDEY ENGLISHChapter:ELECTROSTATICSExercise:Comprehension
Explore 36 Videos
- ELECTROSTATICSBook:DC PANDEY ENGLISHChapter:ELECTROSTATICSExercise:Matrix Matching
Explore 2 Videos
- ELECTROSTATICSBook:DC PANDEY ENGLISHChapter:ELECTROSTATICSExercise:Integer
Explore 17 Videos
- ELASTICITYBook:DC PANDEY ENGLISHChapter:ELASTICITYExercise:Medical entrances s gallery
Explore 21 Videos
- EXPERIMENTSBook:DC PANDEY ENGLISHChapter:EXPERIMENTSExercise:Subjective
Explore 15 Videos
Similar Questions
Figue shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
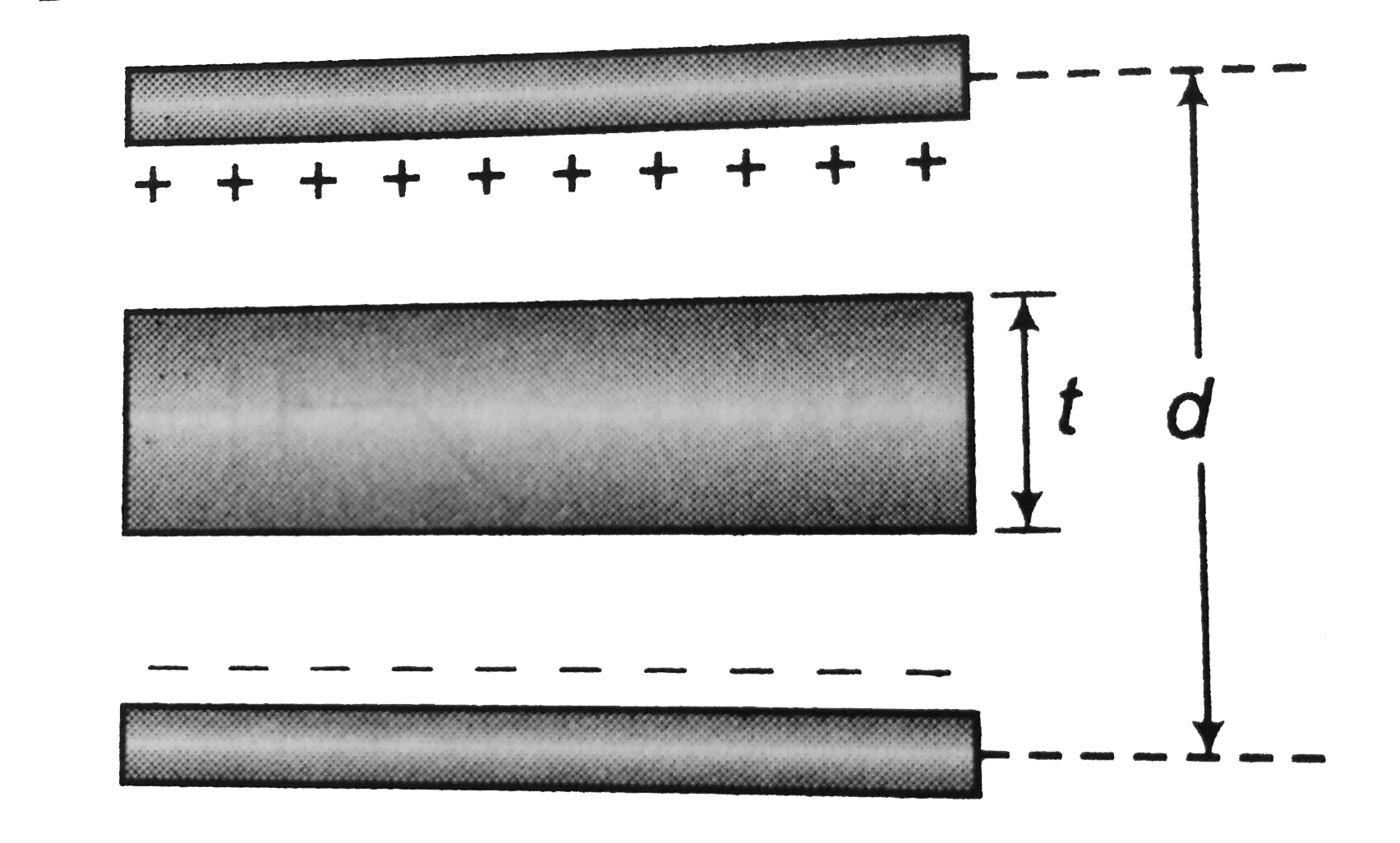
The electric field in the dielectric slab is
View SolutionFigure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
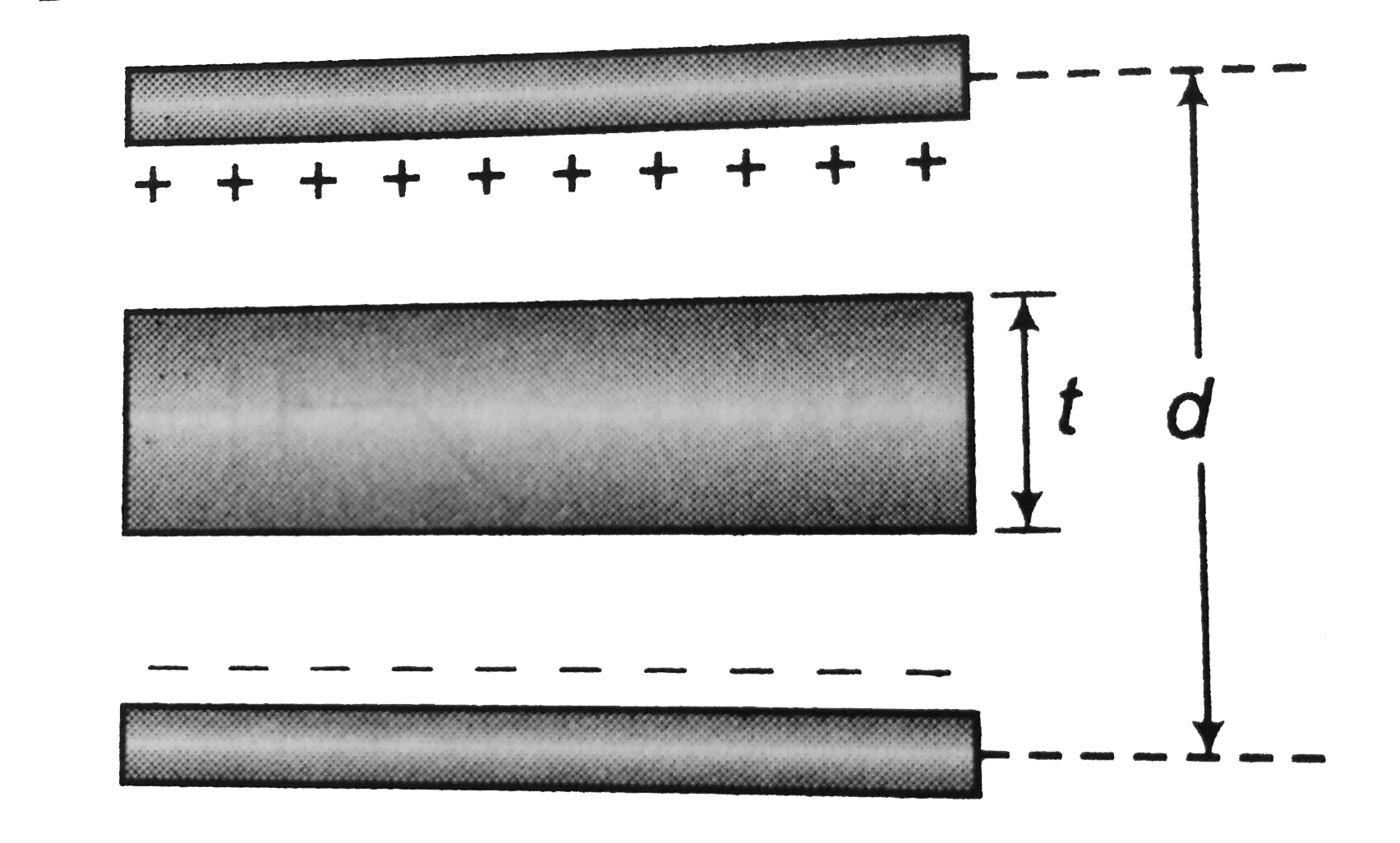
The electric field in the gaps between the plates and the electric slab will be
View Solution
Knowledge Check
A capacitor has some dielectric between its plates, and the capacitor is connected to a DC source. The battery is now disconnected and then the dielectric is removed. State whether the capacitance, the energy stored in it, electric field, charge stored and the voltage will increase ro remain constant.
Acapacitance will increase.Benergy stored will decrease.Celectric field will increase.Dvoltage will decrease.
The terminals of a battery of emf V are connected to the two plates of a parallel plate capacitor. If the space between the plates of the capacitor is filled with an insulator of dielectric constant K, then :
View SolutionThree capacitors having capacitances of 8.4μF,8.4μF and 4.2μF are connected in series across a 36 potential difference.
(a) What is the charge on 4.2μF capacitor?
(b) What is the total energy stored in all three capacitors?
(c) The capacitors are disconnected from the potential difference without allowing them to discharge. They are then reconnected in parallel with each other, with the positively charged plates connected together. What is the voltage across each capacitor in the parallel combination?
(d) What is the total energy now stored in the capacitors?
View SolutionA parallel plate capacitor is charged to a potential difference V by a dc source. The capacitor is then disconnected from the source. If the distance between the plates in doubled, state with reason how the following will change,
(i) electric field between the plates,
(ii) capacitance and
(iii) energy stored in the capacitor.
View SolutionA capacitor of capacitance C1 is charged to a potential V1 while another capacitor of capacitance C2 is charged to a potential difference V2 . The capacitors are now disconnected from their respective charging batteries and connected in parallel to each other .
(a) Find the total energy stored in the two capacitors before they are connected.
(b) Find the total energy stored in the parallel combination of the two capacitors.
(c ) Explain the reason for the difference of energy in parallel combination in comparison to the total energy before they are connected.
View SolutionA parallel-plate capacitor is filled with a dielectric up to one-half of the distance between the plates.The manner in which the potential between the plates varies is illustrated in the figure. Which half (1or2) of the space between the plates is filled with the dielectric and what will be the distribution of the potential after the dielectric is taken out of the capacitor provided that (a) the charges on the plates are conserved or (b) the potential difference across the capacitor is conserved?
View SolutionA parallel plate capacitor having capacitance 12pF is charged bya battery to a potential difference of 10V between its plates. The charging battery is now disonnected and a porcelain slab of dielectric constant 6.5 is slipped between the plates. The work done by the capacitor on the slab is
View SolutionA capacitor has some dielectric between its plates, and the capacitor is connected to a DC source. The battery is now disconnected and then the dielectric is removed. State whether the capacitance, the energy stored in it, electric field, charge stored and the voltage will increase ro remain constant.
View Solution