A horizontal wid is blowing with a velocity v towards north-east. A man starts running towards north with acceleration a. The after which man will feel the wind blowing towards east is
va
√2Va
v√2a
2va
The correct Answer is:C
To solve the problem, we need to determine the time after which the man running towards the north will feel the wind blowing only towards the east. This occurs when the northward component of the wind's velocity equals the man's velocity in the northward direction.
1. Understand the Wind's Velocity:
The wind is blowing towards the north-east with a velocity v. We can break this velocity into its components:
- The northward component (y-direction) of the wind's velocity is:
\(
v_y = \frac{v}{\sqrt{2}}
\)
- The eastward component (x-direction) of the wind's velocity is:
\(
v_x = \frac{v}{\sqrt{2}}
\)
2. Man's Motion:
The man starts running towards the north with an acceleration a. Since he starts from rest, his velocity at time t can be expressed using the equation of motion:
\(
v_m = a t
\)
3. Condition for Feeling Wind Only Towards East:
The man will feel the wind blowing only towards the east when the northward component of the wind's velocity equals the man's velocity. Therefore, we set the northward component of the wind's velocity equal to the man's velocity:
\(
v_y = v_m
\)
Substituting the expressions we have:
\(
\frac{v}{\sqrt{2}} = a t
\)
4. Solve for Time t:
Rearranging the equation to solve for t gives:
\(
t = \frac{v}{\sqrt{2} a}
\)
Final Answer:
The time after which the man will feel the wind blowing only towards the east is:
\(
t = \frac{v}{\sqrt{2} a}
\)
The wind is blowing towards the north-east with a velocity v. We can break this velocity into its components:
- The northward component (y-direction) of the wind's velocity is:
\(
v_y = \frac{v}{\sqrt{2}}
\)
- The eastward component (x-direction) of the wind's velocity is:
\(
v_x = \frac{v}{\sqrt{2}}
\)
The man starts running towards the north with an acceleration a. Since he starts from rest, his velocity at time t can be expressed using the equation of motion:
\(
v_m = a t
\)
The man will feel the wind blowing only towards the east when the northward component of the wind's velocity equals the man's velocity. Therefore, we set the northward component of the wind's velocity equal to the man's velocity:
\(
v_y = v_m
\)
Substituting the expressions we have:
\(
\frac{v}{\sqrt{2}} = a t
\)
Rearranging the equation to solve for t gives:
\(
t = \frac{v}{\sqrt{2} a}
\)
The time after which the man will feel the wind blowing only towards the east is:
\(
t = \frac{v}{\sqrt{2} a}
\)
Topper's Solved these Questions
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - MCQ_TYPE
27 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - COMPREHENSION_TYPE
19 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
Similar Questions
A particle is moing with a velocity of 10m/s towards east. After 10s its velocity changes to 10m/s towards north. Its average acceleration is:-
View SolutionA body is moving with velocity 30m/s towards east. After 10s its velocity becomes 40m/s towards north. The average acceleration of the body is.
View Solution
Knowledge Check
A particle is moving at 5ms−1 towards east In one second its velocity changes to 5ms−1 towards north. Assuming the acceleration to be uniform, the change in velocity will be directed at
A135∘ to eastB135∘ to northC45∘ to eastD90∘ to east
A body is moving with velocity 30m/s towards east. After 10s its velocity becomes 40m/s towards north. The average acceleration of the body is.
View SolutionA boat is moving towards east velocity 4 m/s w.r.t still water and river is flowing towards north with velocity 2 m/s and the wind blowing towards north with velocity 6 m/s. the direction of the flag blown over by the wind hpisted on the boat is
View SolutionA car is moving with velocity →vc towards north and a train is moving towards east with velocity →vt . Find the direction of relative velocity of car with respect to train.
View SolutionA bota has a velocity 4m/s towards east with respect to river is flowing north with velocity 2m/s Wind is blowing towards north with velocity 6m/s. The direction of the flag blown over by the wind hoisted on the boat is :
View SolutionThree ships A, B and C are in motion. The motion of A as seen by B is with speed v towards north-east . The motion. Of B as seen by C is with speed v towards the north-west. Then as seen by A, C will be moving towards
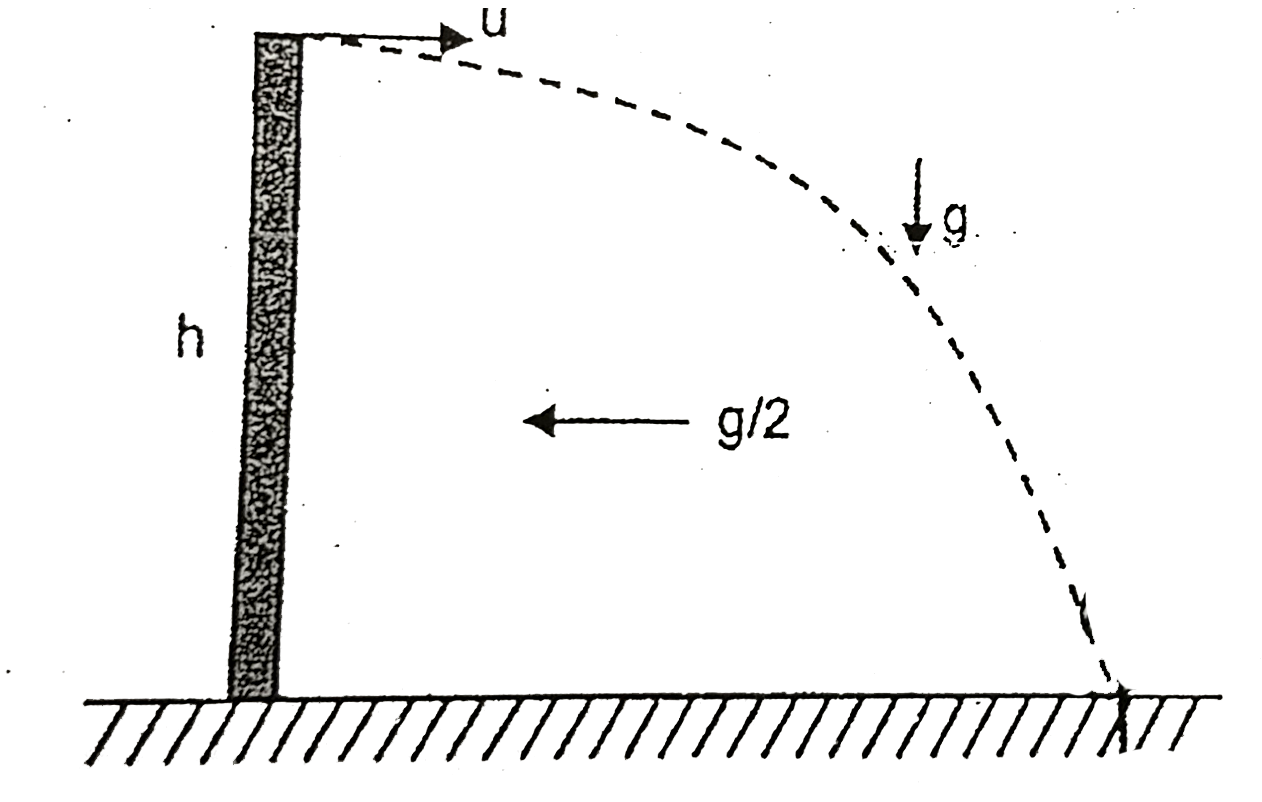
View SolutionA particle is projected horizontally from a tower with velocity u towards east. Wind provides constant horizontal acceleration g2 towards west. If particle strikes the ground vertically the then :
View SolutionA train is moving due east and a car is moving due north with equal speeds. A passenger in the train finds that the car is moving towards
View Solution