The displacement of two identical particles executing SHM are represented by equations.
x1=4sin(10t+π6) and x2=5cosεt
For what value of epsilon energy of both the particles is same?
16 unit
6 unit
4 unit
8 unit
The correct Answer is:D
To find the value of epsilon for which the energy of both particles executing Simple Harmonic Motion (SHM) is the same, we can follow these steps:
Step 1: Understand the energy of SHM
The total energy (E) of a particle in SHM is given by the formula:
E=12kA2
where k is the force constant and A is the amplitude of the motion.
Step 2: Relate energy to angular frequency
The force constant k can also be expressed in terms of mass m and angular frequency ω:
k=mω2
Thus, the energy can be rewritten as:
E=12mω2A2
Step 3: Set up the equations for both particles
For the first particle:
- Displacement: x1=4sin(10t+π6)
- Amplitude A1=4
- Angular frequency ω1=10
The energy of the first particle is:
E1=12m(102)(42)
For the second particle:
- Displacement: x2=5cos(ϵt)
- Amplitude A2=5
- Angular frequency ω2=ϵ
The energy of the second particle is:
E2=12m(ϵ2)(52)
Step 4: Set the energies equal
Since the energies of both particles are the same:
E1=E2
This gives us:
12m(102)(42)=12m(ϵ2)(52)
Step 5: Simplify the equation
We can cancel 12m from both sides (assuming m≠0):
(102)(42)=ϵ2(52)
Step 6: Substitute and solve for epsilon
Calculating the left side:
100×16=1600
Calculating the right side:
ϵ2×25
Setting them equal gives:
1600=25ϵ2
Now, solving for ϵ2:
ϵ2=160025=64
Taking the square root:
ϵ=8
Final Answer
The value of epsilon for which the energy of both particles is the same is:
ϵ=8
---
The total energy (E) of a particle in SHM is given by the formula:
E=12kA2
where k is the force constant and A is the amplitude of the motion.
The force constant k can also be expressed in terms of mass m and angular frequency ω:
k=mω2
Thus, the energy can be rewritten as:
E=12mω2A2
For the first particle:
- Displacement: x1=4sin(10t+π6)
- Amplitude A1=4
- Angular frequency ω1=10
E1=12m(102)(42)
- Displacement: x2=5cos(ϵt)
- Amplitude A2=5
- Angular frequency ω2=ϵ
E2=12m(ϵ2)(52)
Since the energies of both particles are the same:
E1=E2
This gives us:
12m(102)(42)=12m(ϵ2)(52)
We can cancel 12m from both sides (assuming m≠0):
(102)(42)=ϵ2(52)
Calculating the left side:
100×16=1600
Calculating the right side:
ϵ2×25
Setting them equal gives:
1600=25ϵ2
ϵ2=160025=64
Taking the square root:
ϵ=8
The value of epsilon for which the energy of both particles is the same is:
ϵ=8
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - JEE Advanced
34 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - More than one option is correct
50 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise 14.4
4 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
The displacement of two identical particles executing SHM are represented by equations x1=4sin(10t+π6)&x2=5cos(ωt) For what value of ∙, energy of both the particles is same.
View SolutionTwo SHM are represented by equations x1=4sin(ωt+37°) and x2=5cos(ωt). The phase difference between them is
View Solution
Knowledge Check
The displacement of a particle is represented by the equation y=3cos(π4−2ωt).
The motion of the particle isAsimple harmonic with period 2πωBsimple harmonic with period πωCperiodic but not simplee harmonicDnon-periodic.
If two SHMs are represented by equations y1=5sin(2πt+π/6)andy2=5[sin(3π)+√3cos(3πt)]. Find the ratio of their amplitudes.
View SolutionThe displacement of a particle is represented by the equation y=3cos(π4−2ωt).
The motion of the particle is
View SolutionThe displacement of a particle is represented by the equation y=3cos(π4−2ωt).
The motion of the particle is
View SolutionThe displacement (x) is plotted with time (t) for a particle executing SHM as shown below. The correct equation of SHM is
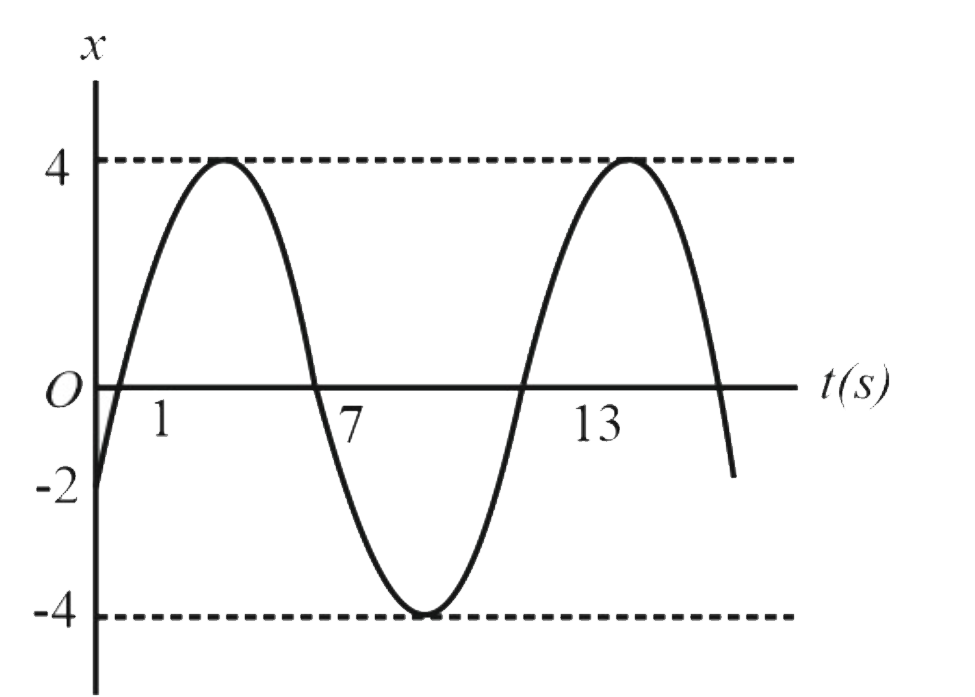
View SolutionThe displacement of a particle executing SHM is given by
y=0.5 sin100t cm . The maximum speed of the particle is
View SolutionIf two SHMs are represented by equations y1= 4 sin[3πt + (π/3)], y2= 4 [sin(3πt) +√3 cos (3πt)], then the ratio of their amplitudes is
View SolutionThe displacement of a particle in SHM is indicated by equation y=10sin(20t+π/3) where, y is in metres. The value of time period of vibration will be (in second)
View Solution
