Maximum velocity ini SHM is vm. The average velocity during motion from one extreme point to the other extreme point will be
π2vm
(2π)vm
(4π)vm
(π4)vm
The correct Answer is:B
To find the average velocity during motion from one extreme point to the other extreme point in Simple Harmonic Motion (SHM), we can follow these steps:
1. Understand the Maximum Velocity in SHM:
The maximum velocity vm in SHM is given by the formula:
vm=ωA
where ω is the angular frequency and A is the amplitude.
2. Identify the Motion from One Extreme to Another:
When the particle moves from one extreme position to the other, it covers a total displacement of 2A (from −A to +A).
3. Determine the Time Taken:
The time taken to move from one extreme to the other is half of the time period T of the motion:
Time taken=T2
4. Calculate the Average Velocity:
The average velocity vavg can be calculated using the formula:
vavg=Total DisplacementTotal Time
Substituting the values:
vavg=2AT2=2A⋅2T=4AT
5. Relate Average Velocity to Maximum Velocity:
We know that ω=2πT. Therefore, we can express T in terms of ω:
T=2πω
Substituting this into the average velocity equation:
vavg=4A2πω=4A⋅ω2π=2Aωπ
6. Substitute for Maximum Velocity:
Now, substituting ωA for vm:
vavg=2πvm
Final Result:
Thus, the average velocity during motion from one extreme point to the other extreme point is:
vavg=2πvm
The maximum velocity vm in SHM is given by the formula:
vm=ωA
where ω is the angular frequency and A is the amplitude.
When the particle moves from one extreme position to the other, it covers a total displacement of 2A (from −A to +A).
The time taken to move from one extreme to the other is half of the time period T of the motion:
Time taken=T2
The average velocity vavg can be calculated using the formula:
vavg=Total DisplacementTotal Time
Substituting the values:
vavg=2AT2=2A⋅2T=4AT
We know that ω=2πT. Therefore, we can express T in terms of ω:
T=2πω
Substituting this into the average velocity equation:
vavg=4A2πω=4A⋅ω2π=2Aωπ
Now, substituting ωA for vm:
vavg=2πvm
Thus, the average velocity during motion from one extreme point to the other extreme point is:
vavg=2πvm
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - JEE Advanced
34 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - More than one option is correct
50 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise 14.4
4 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
A particle execute SHM and its position varies with time as x=Asinωt. Its average speed during its motion from mean position to mid-point of mean and extreme position is
View SolutionHow much time does the bob of a second.s pendulum take to move from one extreme to the other extreme of its oscillation?
View SolutionThe equation of a S.H.M. of amplitude A and angular frequency ω in which all distances are measured from one extreme position and time is taken to be zero at the other extreme position is
View SolutionA particle of mass m executes SHM with amplitude 'a' and frequency 'v'. The average kinetic energy during motion from the position of equilibrium to the end is:
View SolutionThe velocity-time graph of a particle moving along a straight line is shown is Fig. The rate of acceleration and deceleration is constant and it is equal to 5ms−2. If the a average velocity during the motion is 20ms−1, Then
 .
.
The maximum velocity of the particle is .
View SolutionThe velocity-time graph of a particle moving along a straight line is shown is Fig. The rate of acceleration and deceleration is constant and it is equal to 5ms−2. If the a average velocity during the motion is 20ms−1, Then
 .
.
The maximum velocity of the particle is .
View SolutionA projectile is thrown at an angle of 30∘ with a velocity of 10m/s. the change in velocity during the time interval in which it reaches the highest point is
View Solution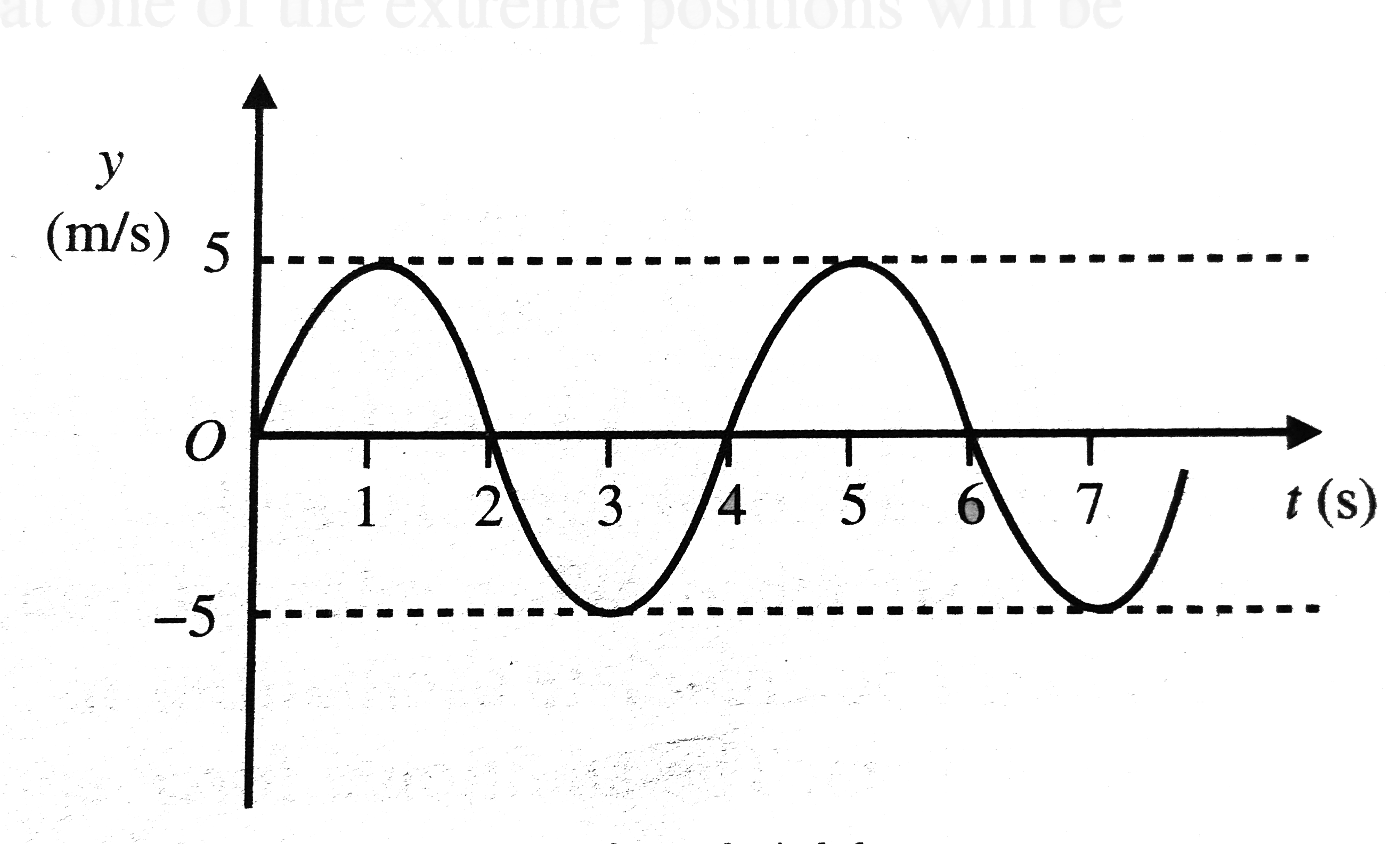
The variation of velocity of a particle executing SHM with time is shown is fig. The velocity of the particle when a phase change of π6 takes place from the instant it is at one of the extreme positions will be
View SolutionA train is moving with uniform acceleration. The two ends of the train pass through a point on the track with velocity v1 and v2. With what velocity the middle point of the train would pass through the same point ?
View SolutionIf velocity of a particle is given by v=(2t+3)m/s. Then average velocity in interval 0≤t≤1 s is :
View Solution
