A horizontal platform with an object placed on it is executing SHM in the vertical direction. The amplitude of oscillation is 4×10−3m. The least period of these oscillations, so that the object is not detached from the platform is π5x second. Find value of x. Take g=10m/s2
The correct Answer is:5
To solve the problem, we need to determine the value of x given the conditions of the simple harmonic motion (SHM) of an object on a platform.
1. Understanding the Problem:
- The platform is executing SHM in the vertical direction with an amplitude A=4×10−3 m.
- The least period of oscillation such that the object does not detach from the platform is given as T=π5x seconds.
- We need to find the value of x.
2. Condition for Detachment:
- The object will not detach from the platform if the maximum acceleration of the object is equal to or less than the acceleration due to gravity g.
- The maximum acceleration amax in SHM is given by:
amax=ω2A
- Here, ω is the angular frequency, and A is the amplitude.
3. Relating Angular Frequency and Period:
- The angular frequency ω is related to the period T by:
ω=2πT
- Substituting this into the equation for maximum acceleration gives:
amax=(2πT)2A
4. Setting Up the Equation:
- For the object to remain on the platform, we need:
amax≤g
- Therefore:
(2πT)2A≤g
5. Substituting Values:
- Substituting A=4×10−3 m and g=10m/s2:
(2πT)2(4×10−3)≤10
6. Solving for Period T:
- Rearranging gives:
(2πT)2≤104×10−3=2500
- Taking the square root:
2πT≤50
- Thus:
T≥2π50=π25
7. Equating to Given Period:
- We know T=π5x, so we set:
π5x=π25
8. Solving for x:
- Cancelling π from both sides:
15x=125
- Cross-multiplying gives:
25=5x⟹x=5
Final Answer:
The value of x is 5.
- The platform is executing SHM in the vertical direction with an amplitude A=4×10−3 m.
- The least period of oscillation such that the object does not detach from the platform is given as T=π5x seconds.
- We need to find the value of x.
- The object will not detach from the platform if the maximum acceleration of the object is equal to or less than the acceleration due to gravity g.
- The maximum acceleration amax in SHM is given by:
amax=ω2A
- Here, ω is the angular frequency, and A is the amplitude.
- The angular frequency ω is related to the period T by:
ω=2πT
- Substituting this into the equation for maximum acceleration gives:
amax=(2πT)2A
- For the object to remain on the platform, we need:
amax≤g
- Therefore:
(2πT)2A≤g
- Substituting A=4×10−3 m and g=10m/s2:
(2πT)2(4×10−3)≤10
- Rearranging gives:
(2πT)2≤104×10−3=2500
- Taking the square root:
2πT≤50
- Thus:
T≥2π50=π25
- We know T=π5x, so we set:
π5x=π25
- Cancelling π from both sides:
15x=125
- Cross-multiplying gives:
25=5x⟹x=5
The value of x is 5.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Matrix matching type questions
13 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
A horizontal platform with an object placed on it is executing SHM in the vertical direction . The amplitude of oscillation is 2.5 cm what must be the least period of these oscillations so that the object is not detached ?
View SolutionA horizontal platform with an object placed on it is executing S.H.M. in the vertical direction. The amplitude of oscillation is 3.92×10−3 m. what must ve the least period of these oscillations. So that the object is not detached from the platform
View Solution
Knowledge Check
The kinetic energy of a particle, executing S.H.M. is 16 J when it is in its mean position. IF the amplitude of oscillation is 25 cm and the mass of the particle is 5.12 Kg, the time period of its oscillations is
Aπ/5 secB2πsecC20πsecD5π sec
A horizontal platform with an object placed on it is executing S.H.M. in the vertical direction. The amplitude of oscillation is 3.92×10−3m. What must be the least period of these oscillation, so that the object is not detached from the platform
View Solution
A horizontal platform with a mass m placed on it is executing SHM slong y-axis. If the amplitude of oscillation is 2.5 cm, the minimum period of the motion for the mass not to be detached from the platform (g=10ms2)
View Solution
A horizontal platform with a mass m placed on it is executing SHM slong y-axis. If the amplitude of oscillation is 2.5 cm, the minimum period of the motion for the mass not to be detached from the platform (g=10ms2)
View SolutionThe maximum velocity of a particle, executing SHM with an amplitude 7 mm is 4.4 m/s. the period of oscillation is
View SolutionThe force on a body executing SHM is 4 N when the displacement from mean position is 2 cm. If amplitude of oscillation is 10 cm, then the maximum kinetic energy associated with the SHM will be
View SolutionA particle of mass 0.1 kg executes SHM under a for F=(−10x) N. Speed of particle at mean position 6m/s. Then amplitude of oscillations is
View SolutionA block is kept is kept over a horizontal platform, executing vertical SHM of angular frequency ω. Find maximum amplitude of oscillations, so that the block does not leave contact with the platform.
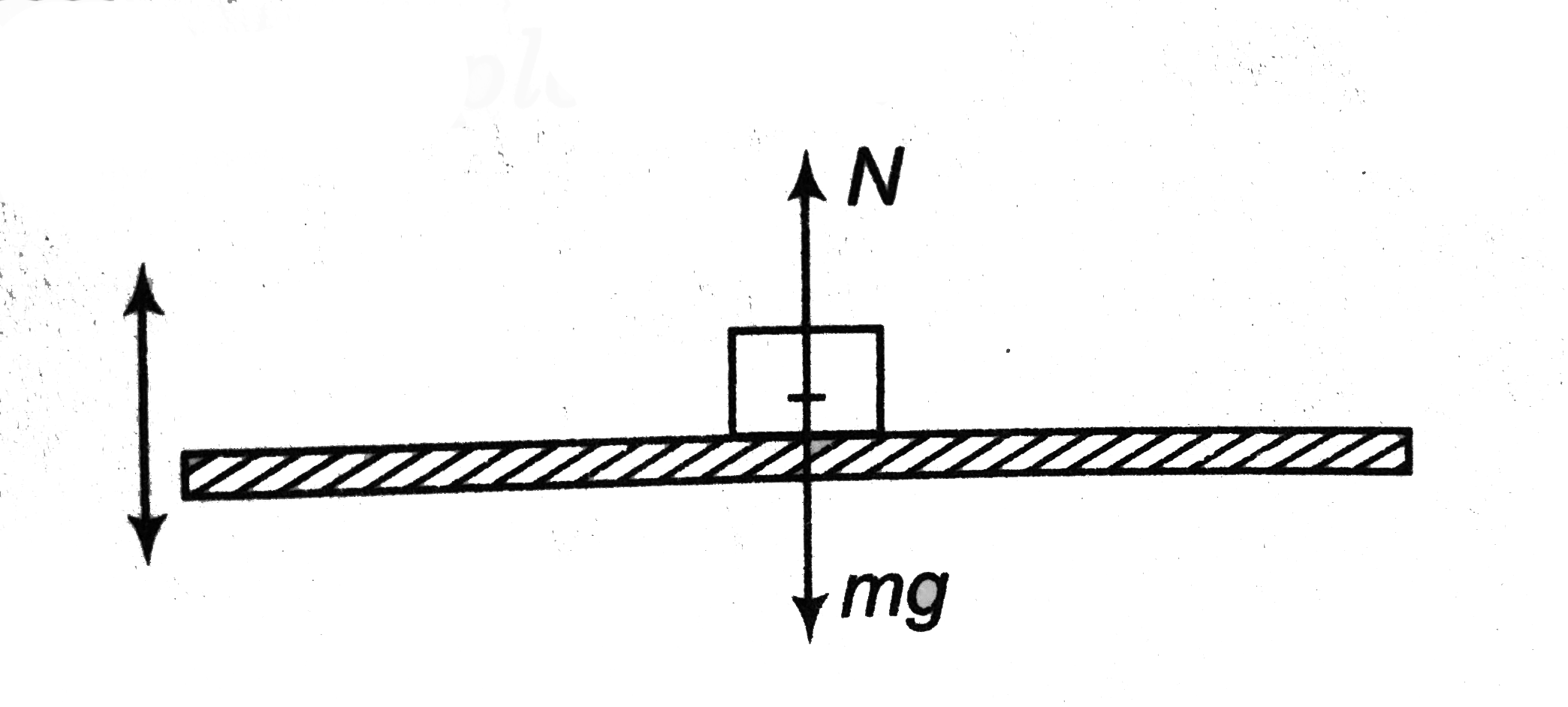
View Solution
