A particle has chrage and a particle has charge with each of them having the same mass . When allowed to fall from rest through the same electric potential difference, the ratio of their speed will become
The correct Answer is:B
To solve the problem, we need to find the ratio of the speeds of two charged particles, A and B, after they fall through the same electric potential difference.
1. Understanding the Problem:
- Particle A has charge +q and mass m.
- Particle B has charge +4q and mass m.
- Both particles fall through the same electric potential difference V.
2. Using Energy Conservation:
- The change in kinetic energy (ΔKE) of each particle is equal to the change in potential energy (ΔPE).
- The equation for kinetic energy is given by:
ΔKE=12mv2
- The potential energy change when a charge q moves through a potential difference V is:
ΔPE=qV
3. Setting Up the Equations:
- For particle A:
12mv2A=qV(1)
- For particle B:
12mv2B=4qV(2)
4. Dividing the Equations:
- Divide equation (1) by equation (2):
12mv2A12mv2B=qV4qV
- Simplifying this gives:
v2Av2B=14
5. Finding the Ratio of Speeds:
- Taking the square root of both sides:
vAvB=12
6. Conclusion:
- The ratio of the speeds of particles A and B is:
vAvB=12
Final Answer:
The ratio of their speeds vAvB is 12.
---
- Particle A has charge +q and mass m.
- Particle B has charge +4q and mass m.
- Both particles fall through the same electric potential difference V.
- The change in kinetic energy (ΔKE) of each particle is equal to the change in potential energy (ΔPE).
- The equation for kinetic energy is given by:
ΔKE=12mv2
- The potential energy change when a charge q moves through a potential difference V is:
ΔPE=qV
- For particle A:
12mv2A=qV(1)
- For particle B:
12mv2B=4qV(2)
- Divide equation (1) by equation (2):
12mv2A12mv2B=qV4qV
- Simplifying this gives:
v2Av2B=14
- Taking the square root of both sides:
vAvB=12
- The ratio of the speeds of particles A and B is:
vAvB=12
The ratio of their speeds vAvB is 12.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.5
20 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
An α-particle and a proton are accelerated from rest through the same potential difference V. Find the ratio of de-Broglie wavelength associated with them.
View SolutionAn electron of mass m and charge e is accelerated from rest through a potential difference V in vacuum. The final speed of the electron will be
View SolutionAn electron of mass m and charge e is accelerated from rest through a potential difference V in vacuum. The final speed of the electron will be
View SolutionThe ratio of the deBroglie wavelengths of proton, deuteron and alpha particle accelerated through the same potential difference 100V is
View SolutionA particle of charges Q and mass m travels through a potential difference V from rest. The final momentum of the particle is
View SolutionIf λ1 and λ2 denote the de-Broglie wavelength of two particles with same masses but charges in the ratio of 1:2 after they are accelerated from rest through the same potential difference, then
View SolutionAn α - particle and a proton are accelerated through same potential difference. Find the ratio (va/vp) of velocities acquired by two particles.
View SolutionFigure shows two rings having charges Q and −√5Q. Find potential difference a and B i.e. (VA−VB).
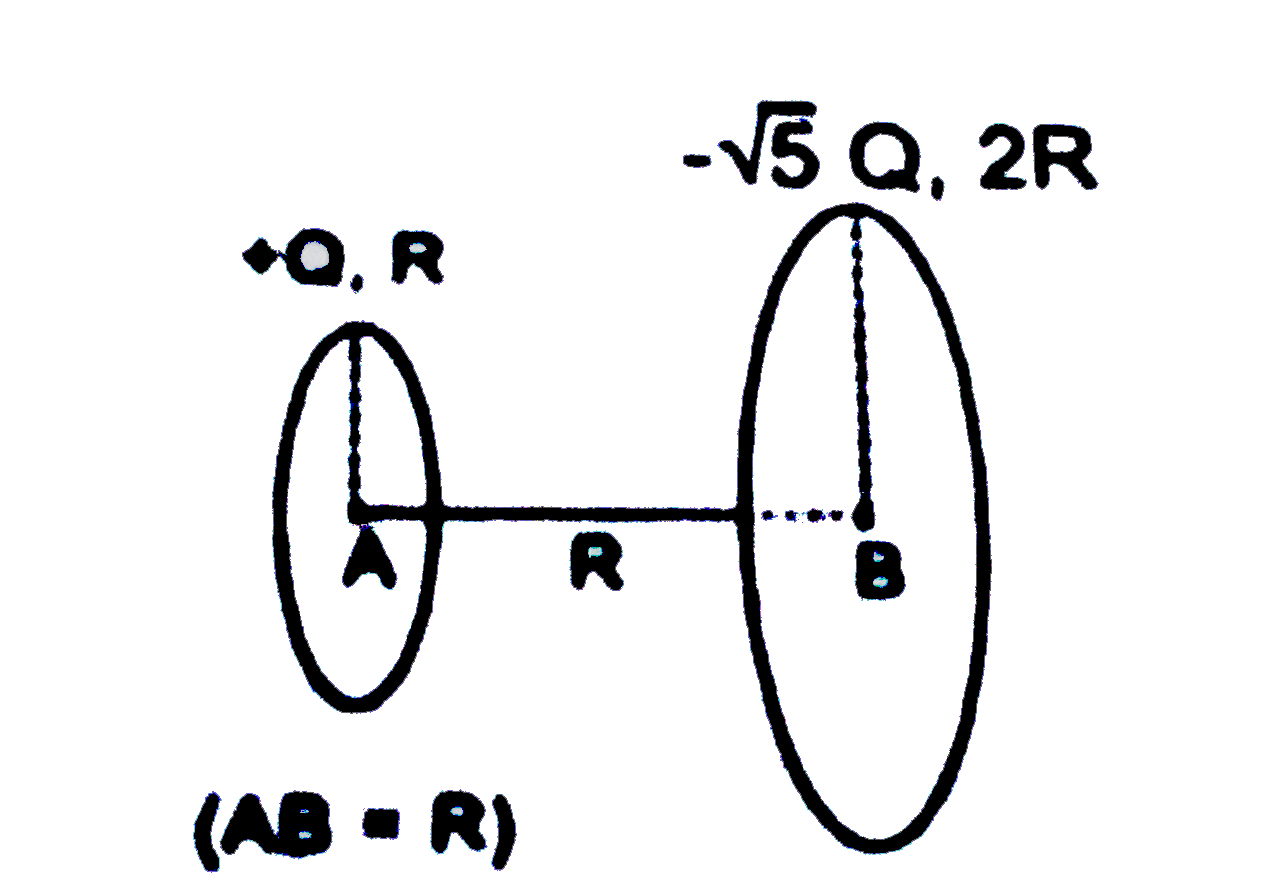
View SolutionMention the significance of Davisson-Germer experiment. An α-particle and a proton are accelerated from rest through the same potential difference V. Find the ratio of de-Broglie wavelengths associated with them.
View SolutionA proton and an α− Particle ( with their masses in the ratio of 1:4 and charges in the ratio of 1:2 ) are accelerated form rest through a potential differeence V, if a uniform magnetic field (B) is set up perpendicular to their velocities , the ratio of the radii rp:rα of the circular paths described by them will be :
View Solution
