Assertion : V = iR is Ohm's law.
Reason : V - I graph is always a straight line passing through origin.
If both Assertion and Reason are true and Reason is the correct explanation of Assertion.
If both Assertion and Reason are true and Reason is not correct explanation of Assertion.
If Assertion is true but Reason is false.
If Assertion is false but Reason is true.
The correct Answer is:C
To solve the assertion and reason question, we need to analyze both statements carefully.
Step 1: Understand the Assertion
The assertion states that "V = iR is Ohm's law."
- Explanation: Ohm's law indeed states that the voltage (V) across a conductor is directly proportional to the current (I) flowing through it, provided the temperature and physical state of the conductor remain constant. The equation V = iR represents this relationship, where R is the constant resistance.
Step 2: Understand the Reason
The reason states that "V - I graph is always a straight line passing through the origin."
- Explanation: The V-I graph (Voltage vs. Current graph) is a straight line passing through the origin only for ohmic conductors, where the resistance remains constant. However, for non-ohmic conductors, the graph can be non-linear, meaning it does not always pass through the origin or remain a straight line.
Step 3: Analyze the Relationship Between Assertion and Reason
- The assertion is true because V = iR is indeed Ohm's law.
- The reason is false because the V-I graph does not always represent a straight line passing through the origin for all types of conductors. It is only true for ohmic conductors.
Conclusion
- Assertion: True
- Reason: False
- Therefore, the correct answer is that the assertion is true, but the reason is false.
Final Answer
The assertion is true, and the reason is false. The correct option is C.
---
The assertion states that "V = iR is Ohm's law."
- Explanation: Ohm's law indeed states that the voltage (V) across a conductor is directly proportional to the current (I) flowing through it, provided the temperature and physical state of the conductor remain constant. The equation V = iR represents this relationship, where R is the constant resistance.
The reason states that "V - I graph is always a straight line passing through the origin."
- Explanation: The V-I graph (Voltage vs. Current graph) is a straight line passing through the origin only for ohmic conductors, where the resistance remains constant. However, for non-ohmic conductors, the graph can be non-linear, meaning it does not always pass through the origin or remain a straight line.
- The assertion is true because V = iR is indeed Ohm's law.
- The reason is false because the V-I graph does not always represent a straight line passing through the origin for all types of conductors. It is only true for ohmic conductors.
- Assertion: True
- Reason: False
- Therefore, the correct answer is that the assertion is true, but the reason is false.
The assertion is true, and the reason is false. The correct option is C.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Match the columns
4 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Taking it together
142 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
Assertion: In isobaric process, (V - T) graph is a straight line passing through origin. Slope of this line is directly proportional to mass of the gas. (V) is taken on (y - axis).
Reason: V=(nRp)T
∴ Slope∝n
or slope∝m.
View SolutionThe p-T graph for the given mass of an ideal gas is shown in figure. What inference can be drawn regarding the change in volume (whether it is constant, increasing or decreasing) ?
How to proceed Definitely, it is not constant. Because when volume of the gas is constant p−T graph is a straight line passing through origin. The given line does not pass through origin, hence volume is not constant. .
.
V=(nR)(TP)
Now, to see volume of the gas we will have to see whether TP is increasing or decreasing.
View SolutionFind the differential equation of family of all straight lines passing through the origin .
View SolutionIf the P-V diagram of a diatomic gas is plotted, it is a straight line passing through the origin. The molar heat capacity of the gas in the process is IR, where I is an integer. Find the value of I.
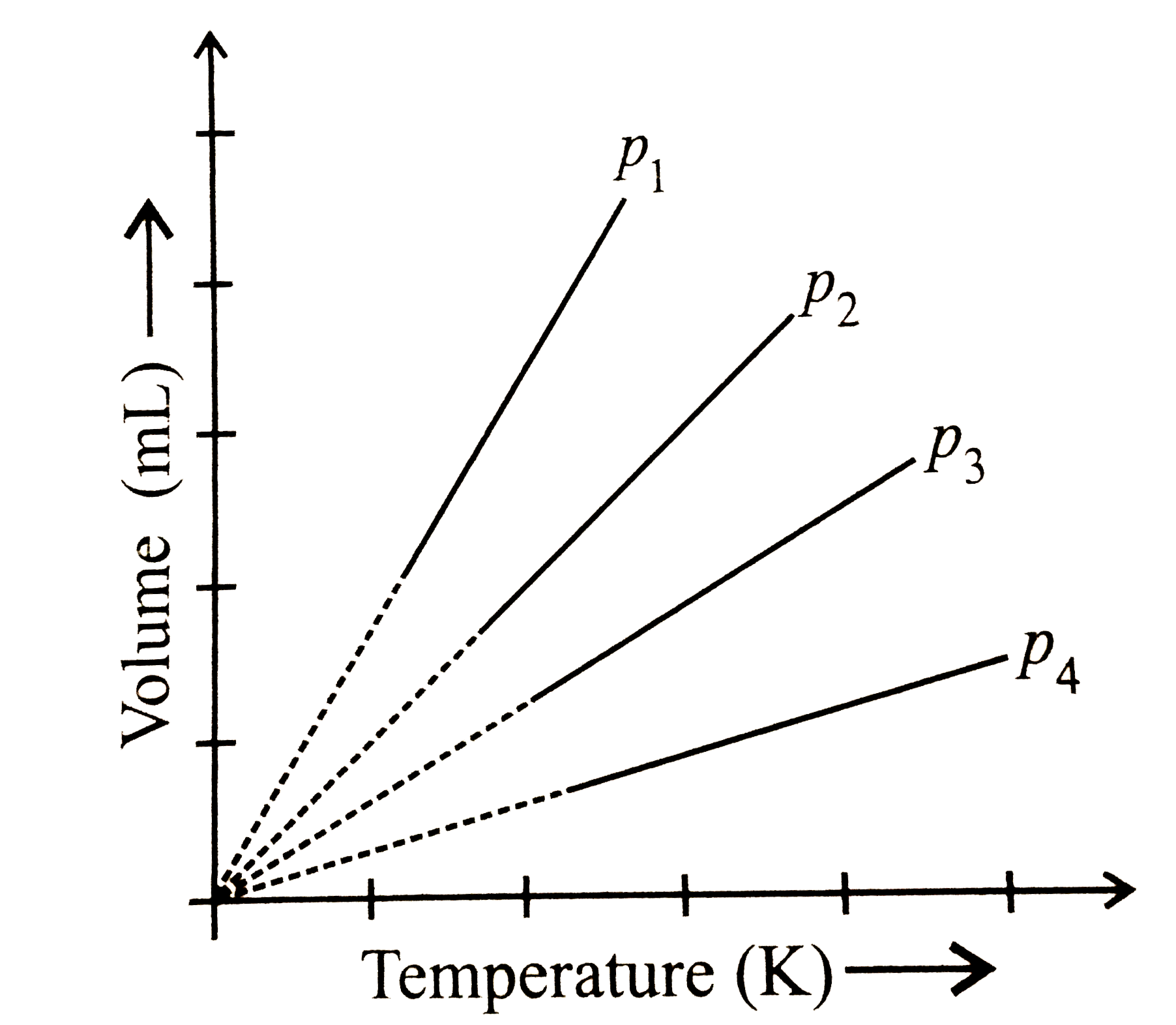
View SolutionA plot of volume (V) versus temperature (T) for a gas at constant pressure is a straight line passing through the origin. The plots at different values of pressure are shown in figure. Which of the following order of pressure is correct for this gas ?
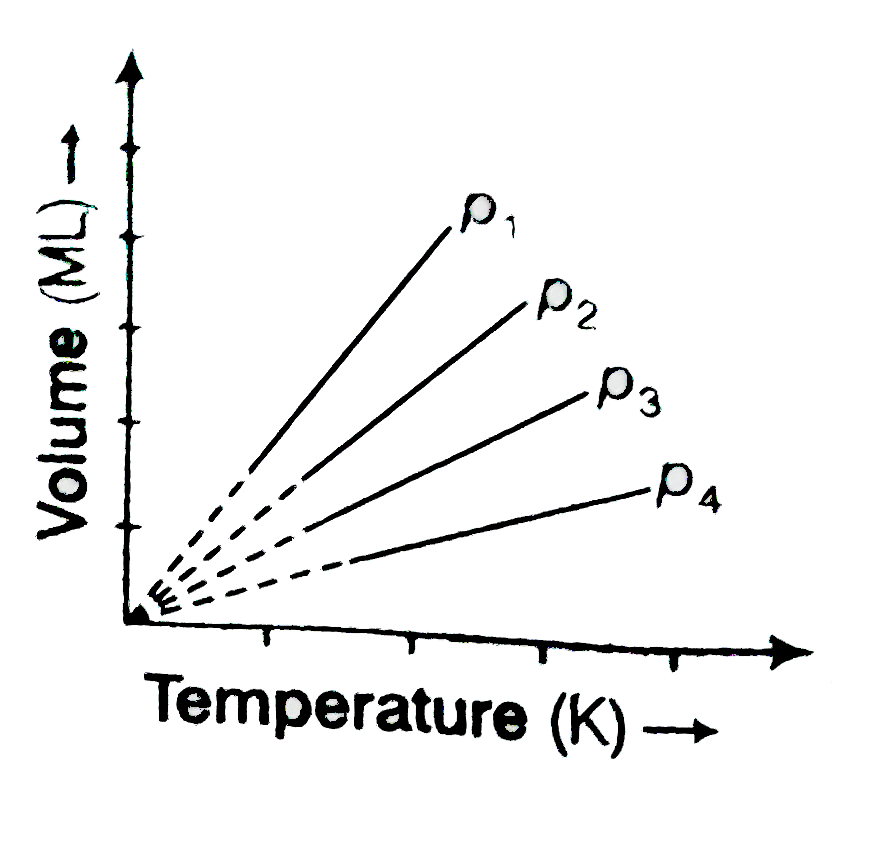
View SolutionA plot of volume (V) versus temperature (T) for a gas at constant pressure is a straight line passing through the origin . The plots at different values of pressure are shown in figure .
Which of the following order of pressure is correct for this gas ?
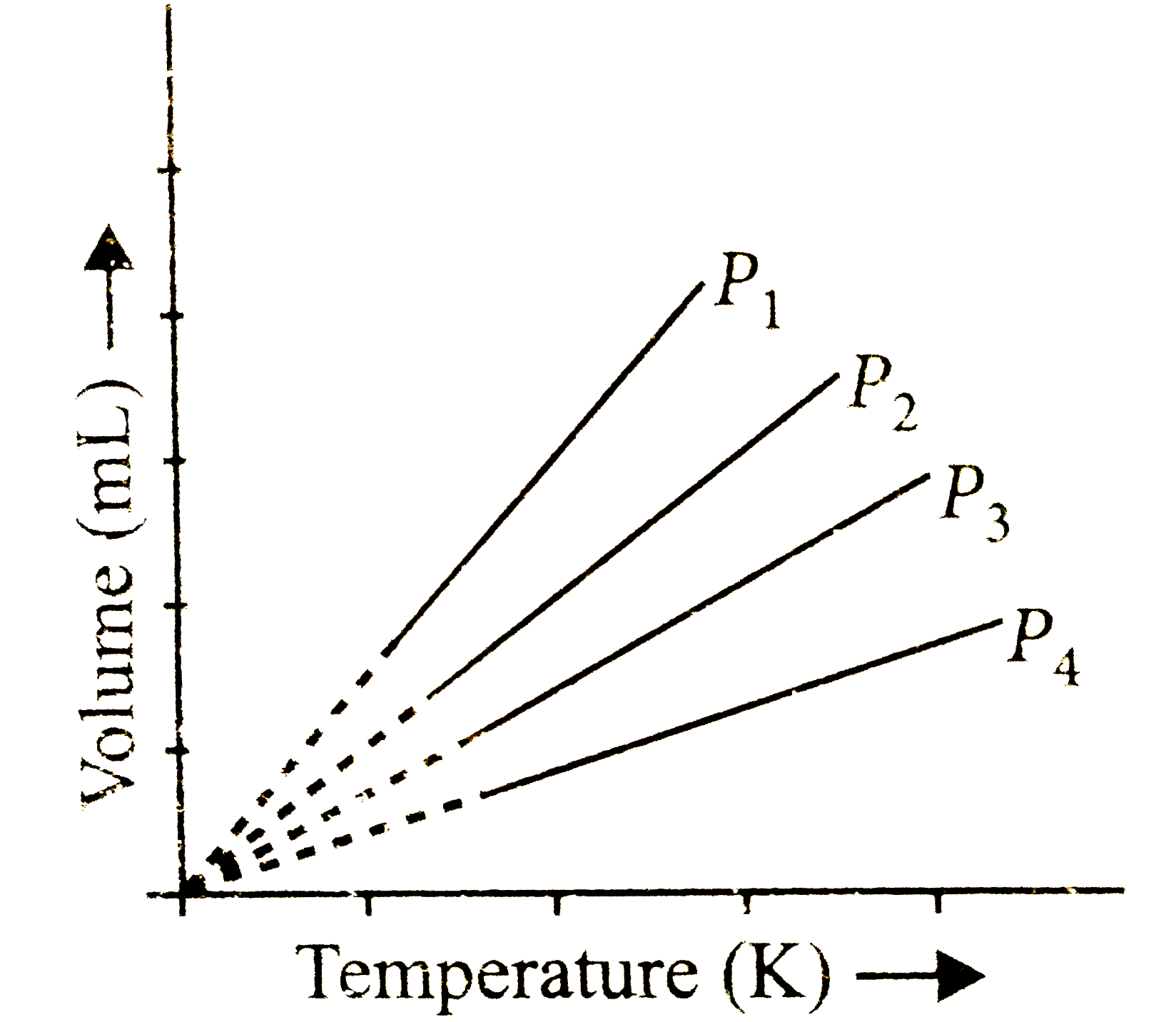
View SolutionA plot of volume (V) versus temperature (T) for a gas at constannt pressure is a straight line passing through the origin. The plots at different values of pressure are shown in figure. Which of the following order of pressure is correct for this gas?
View SolutionAssertion : Path of a particle in SHM is always a straight line.
Reason : All straight line motions are not simple harmonic.
View SolutionWhile stud dying the dependence of potential difference (V) across a resistor on the current
(I) passing through it, in order to determine the resistance of the resistor, a student took
5 readings for different values of current and plotted a graph between V and I. He got a
straight line graph passing through the origin. What does the straight line signify? Write
the method of determining resistance of the resistor using this graph.
View Solution|z−3i|=2|z−3| represents a straight line passing through (0+3) and (1+0) a straight line passing through origin a circle with centre (4, -1) and radius √8 none of these
View Solution
