Currents of 10A,2A are passed through two parallel wires A and B respectively in opposite directions. If the wire A is infinitely long and the length of the wire B is 2 metre, the force on the conductor B, which is situated at 10cm distance from A will be
To solve the problem, we need to calculate the force on wire B due to the magnetic field created by wire A. Here are the steps to arrive at the solution:
Step 1: Identify the Given Values
- Current in wire A, I1=10A
- Current in wire B, I2=2A
- Length of wire B, L=2m
- Distance between the wires, d=10cm=0.1m
Step 2: Calculate the Magnetic Field due to Wire A at the Location of Wire B
The magnetic field B at a distance d from a long straight conductor carrying current I is given by the formula:
B=μ0I12πd
where μ0 (the permeability of free space) is approximately 4π×10−7T m/A.
Substituting the values:
B=4π×10−7×102π×0.1
B=4×10−7×100.2=4×10−60.2=2×10−5T
Step 3: Determine the Direction of the Magnetic Field
Using the right-hand rule, since the currents in wires A and B are in opposite directions, the magnetic field at the location of wire B (due to wire A) will be directed into the plane of the paper.
Step 4: Calculate the Force on Wire B
The force FB on a current-carrying conductor in a magnetic field is given by:
FB=I2LBsinθ
Since the angle θ between the current direction in wire B and the magnetic field is 90∘, we have sin90∘=1:
FB=I2LB
Substituting the values:
FB=2×2×10−5
FB=4×10−5N
Step 5: Final Calculation
The force on wire B is:
FB=4×10−5N
Conclusion
The force on conductor B, which is situated at a distance of 10 cm from A, is 4×10−5N.
---
- Current in wire A, I1=10A
- Current in wire B, I2=2A
- Length of wire B, L=2m
- Distance between the wires, d=10cm=0.1m
The magnetic field B at a distance d from a long straight conductor carrying current I is given by the formula:
B=μ0I12πd
where μ0 (the permeability of free space) is approximately 4π×10−7T m/A.
B=4π×10−7×102π×0.1
B=4×10−7×100.2=4×10−60.2=2×10−5T
Using the right-hand rule, since the currents in wires A and B are in opposite directions, the magnetic field at the location of wire B (due to wire A) will be directed into the plane of the paper.
The force FB on a current-carrying conductor in a magnetic field is given by:
FB=I2LBsinθ
Since the angle θ between the current direction in wire B and the magnetic field is 90∘, we have sin90∘=1:
FB=I2LB
FB=2×2×10−5
FB=4×10−5N
The force on wire B is:
FB=4×10−5N
The force on conductor B, which is situated at a distance of 10 cm from A, is 4×10−5N.
Topper's Solved these Questions
- MAGNETIC FIELD AND FORCESBook:DC PANDEY ENGLISHChapter:MAGNETIC FIELD AND FORCESExercise:Check point
Explore 54 Videos
- MAGNETIC FIELD AND FORCESBook:DC PANDEY ENGLISHChapter:MAGNETIC FIELD AND FORCESExercise:Taking it together
Explore 75 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:INTERFERENCE AND DIFFRACTION OF LIGHTExercise:Level 2 Subjective
Explore 5 Videos
- MAGNETICSBook:DC PANDEY ENGLISHChapter:MAGNETICSExercise:MCQ_TYPE
Explore 1 Video
Similar Questions
Currents of 10 A and 2A are passed through two parallel wires A and B respectuvely, in opposite direction.If the wire A is infinitely long on the wire B is 2 2m then the find the force acting on the conductor B which is situated at 10 cm distance from A
View SolutionIf current in two parallel wires flow in opposite directions, the force between the wires will:
View Solution
Knowledge Check
Two parallel wires 2 m apart carry currents of 2 A and 5 A respectively in same direction, the force per unit length acting between these two wires is
A2×10−6Nm−1B3×10−6Nm−1C1×10−6Nm−1D4×10−6Nm−1
Two long parallel copper wires carry current of 5A each in opposite directions. If the wires are separated by a distance distance of 0.5m, then the force per unit length between the two wires is
View SolutionTwo long parallel wires are 30 cm apart carrying currents 10 A and 15 A respectively in the same direction. The force acting over a length of 5 m of the wires is
View SolutionTwo parallel wires carry currents of 20A and 40A in opposite directions. Another wire carrying a current antiparallel to 20A is placed midway between the two wires. The magnetic force on it will be
View SolutionWhen two parallel wires carrying current l and 2l in same direction are placed at a distance d apart, the ratio of force per unit length acting upon the wire due to other is
View SolutionTwo long parallel straight wires X and Y separated by a distance 5cm in air carry currents of 10A and 5A respectively in opposite directions. Calculate the magnitude and direction of force on a 20cm length of the wire Y. Figure
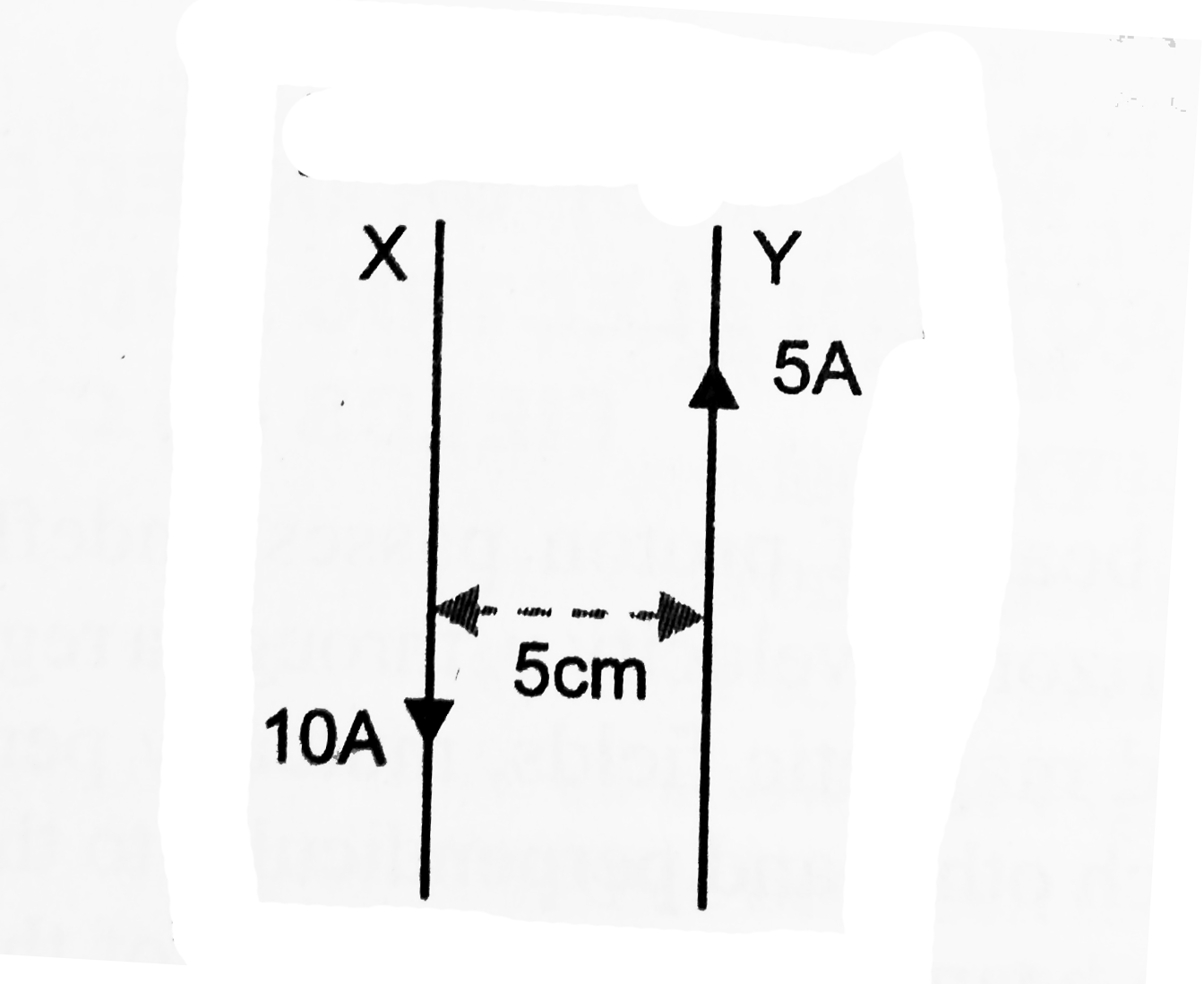
View SolutionTwo parallel conductors A and B of equal lengths carry currents I and 10I, respectively, in the same direction. Then
View SolutionTwo long straight wires, each carrying a current I in opposite directions are separated by a distance R. The magnetic induction at a point mid way between the wire is
View Solution
