Currents of 10A,2A are passed through two parallel wires A and B respectively in opposite directions. If the wire A is infinitely long and the length of the wire B is 2 metre, the force on the conductor B, which is situated at 10cm distance from A will be
8×10−5N
4×10−5N
4×10−7N
8×10−7N
The correct Answer is:A
To solve the problem, we will use the formula for the magnetic force per unit length between two parallel current-carrying wires. The formula is given by:
F/L=μ0I1I22πr
Where:
- F is the force between the wires,
- L is the length of the wire experiencing the force,
- μ0 is the permeability of free space (4π×10−7T m/A),
- I1 and I2 are the currents in the wires,
- r is the distance between the wires.
1. Identify the given values:
- Current in wire A, I1=10A
- Current in wire B, I2=2A
- Distance between the wires, r=10cm=0.1m
- Length of wire B, L=2m
2. Substitute the values into the formula:
- We need to calculate the force per unit length first:
F/L=μ0I1I22πr
Substituting the values:
F/L=(4π×10−7)×(10)×(2)2π×(0.1)
3. Simplify the expression:
- The π in the numerator and denominator cancels out:
F/L=(4×10−7)×(10)×(2)2×(0.1)
- This simplifies to:
F/L=(4×10−7)×200.2
- Further simplifying gives:
F/L=80×10−70.2=400×10−7=4×10−5N/m
4. Calculate the total force on wire B:
- Now, multiply the force per unit length by the length of wire B:
F=(F/L)×L=(4×10−5)×2=8×10−5N
5. Final Result:
- The force on wire B is:
F=8×10−5N
- F is the force between the wires,
- L is the length of the wire experiencing the force,
- μ0 is the permeability of free space (4π×10−7T m/A),
- I1 and I2 are the currents in the wires,
- r is the distance between the wires.
- Current in wire A, I1=10A
- Current in wire B, I2=2A
- Distance between the wires, r=10cm=0.1m
- Length of wire B, L=2m
- We need to calculate the force per unit length first:
F/L=μ0I1I22πr
Substituting the values:
F/L=(4π×10−7)×(10)×(2)2π×(0.1)
- The π in the numerator and denominator cancels out:
F/L=(4×10−7)×(10)×(2)2×(0.1)
- This simplifies to:
F/L=(4×10−7)×200.2
- Further simplifying gives:
F/L=80×10−70.2=400×10−7=4×10−5N/m
- Now, multiply the force per unit length by the length of wire B:
F=(F/L)×L=(4×10−5)×2=8×10−5N
- The force on wire B is:
F=8×10−5N
Topper's Solved these Questions
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Taking it together
75 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Assertion and reason
20 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 2 Subjective
5 Videos
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE
1 Videos
Similar Questions
Currents of 10 A and 2A are passed through two parallel wires A and B respectuvely, in opposite direction.If the wire A is infinitely long on the wire B is 2 2m then the find the force acting on the conductor B which is situated at 10 cm distance from A
View SolutionIf current in two parallel wires flow in opposite directions, the force between the wires will:
View Solution
Knowledge Check
Two parallel wires 2 m apart carry currents of 2 A and 5 A respectively in same direction, the force per unit length acting between these two wires is
A2×10−6Nm−1B3×10−6Nm−1C1×10−6Nm−1D4×10−6Nm−1
Two long parallel copper wires carry current of 5A each in opposite directions. If the wires are separated by a distance distance of 0.5m, then the force per unit length between the two wires is
View SolutionTwo long parallel wires are 30 cm apart carrying currents 10 A and 15 A respectively in the same direction. The force acting over a length of 5 m of the wires is
View SolutionTwo parallel wires carry currents of 20A and 40A in opposite directions. Another wire carrying a current antiparallel to 20A is placed midway between the two wires. The magnetic force on it will be
View SolutionWhen two parallel wires carrying current l and 2l in same direction are placed at a distance d apart, the ratio of force per unit length acting upon the wire due to other is
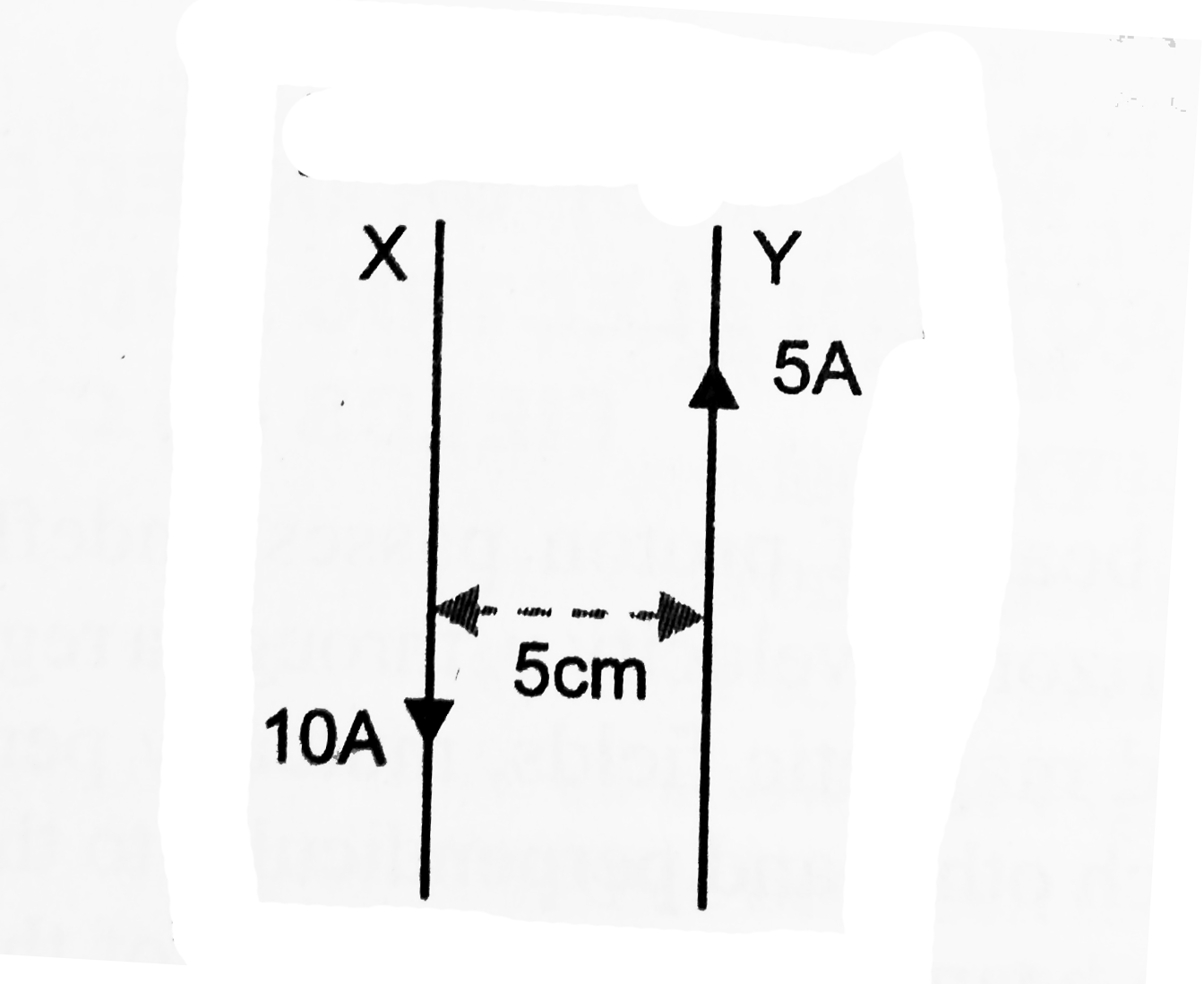
View SolutionTwo long parallel straight wires X and Y separated by a distance 5cm in air carry currents of 10A and 5A respectively in opposite directions. Calculate the magnitude and direction of force on a 20cm length of the wire Y. Figure
View SolutionTwo parallel conductors A and B of equal lengths carry currents I and 10I, respectively, in the same direction. Then
View SolutionTwo long straight wires, each carrying a current I in opposite directions are separated by a distance R. The magnetic induction at a point mid way between the wire is
View Solution
