Two pure inductors each of self-inductance are connected in parallel but are well separted from each other. The total inductance is
2 L
L
The correct Answer is:C
To find the total inductance of two pure inductors, each with self-inductance L, connected in parallel, we can follow these steps:
1. Identify the Inductors: We have two inductors, both with the same self-inductance L.
2. Use the Formula for Inductors in Parallel: The formula for the equivalent inductance Lequiv of inductors in parallel is given by:
1Lequiv=1L1+1L2
Since both inductors have the same inductance L, we can substitute L1=L and L2=L:
1Lequiv=1L+1L
3. Combine the Terms: This simplifies to:
1Lequiv=2L
4. Take the Reciprocal: To find Lequiv, we take the reciprocal of both sides:
Lequiv=L2
5. Conclusion: The total inductance of the two inductors connected in parallel is:
Lequiv=L2
Final Answer:
The total inductance is L2.
---
1Lequiv=1L1+1L2
Since both inductors have the same inductance L, we can substitute L1=L and L2=L:
1Lequiv=1L+1L
1Lequiv=2L
Lequiv=L2
Lequiv=L2
The total inductance is L2.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Taking it together
119 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrance special format questions
17 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Example
71 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
Knowledge Check
Two inductors of inductance L each are connected in series with opposite magnetic fluxes. The resultant inductance is
(Ignore mutual inductance)AzeroBLC2LD3L
Two inductors each of inductance L are connected in parallel. One more inductor of value 5 mH is connected in series of this configuration then the effective inductance is 15 mH . The value of L is ………..mH.
View SolutionShow that if two inductors with equal inductance L are connected in parallel then the equivalent inductance of combination is L/2.The inductors are separated by a large distance.
View SolutionThe self-inductances of two identical coils are 0.1H. They are wound over each other. Mutual inductance will be-
View SolutionAn inductor coil of inductance L is divided into two equal parts and both parts are connected in parallel. The net inductance is :
View SolutionTwo coils of self inductance L1 and L2 are connected in parallel and then connected to a cell of emf ε and internal resistance - R. Find the steady state current in the coils.
View SolutionTwo ends of an inductor of inductance L are connected to two parallel conducting wires. A rod of length l and mass m is gien velocity v0 as shown. The whole sytem is placed in perpendicular magnetic field B. Find the maximum current in the inductor. (Neglect gravity and friction)
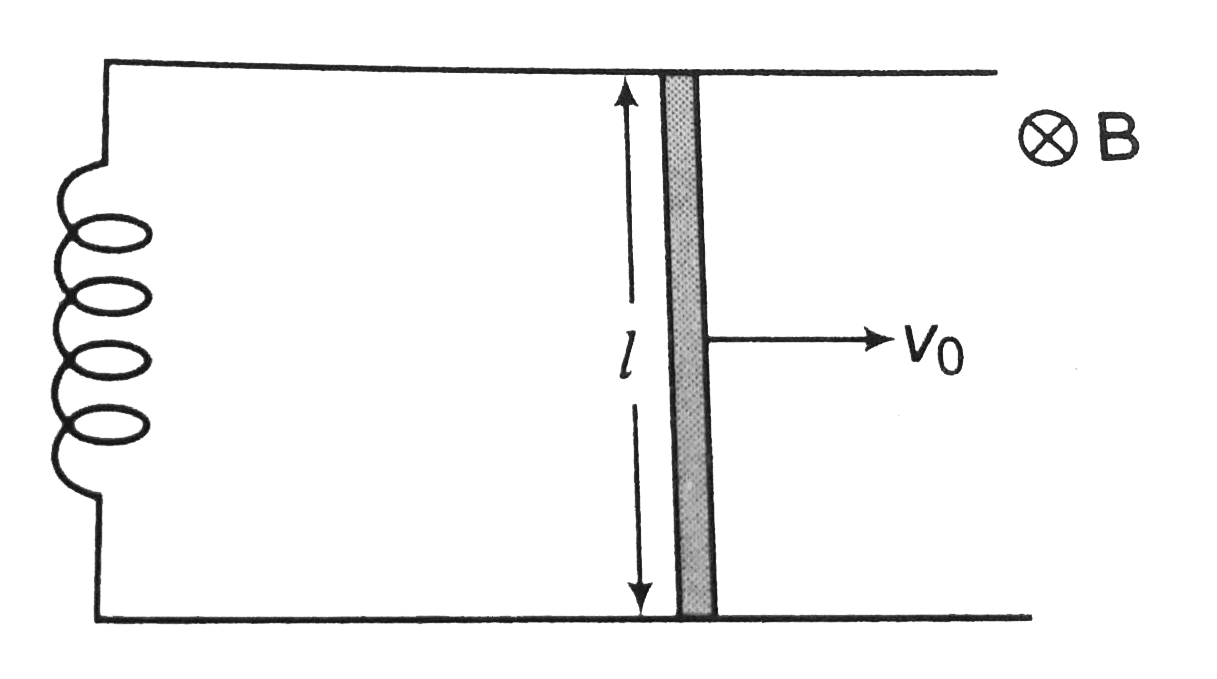
View SolutionA coil of self-inductance L is connected in series with a bulb B and an AC source. Brightness of the bulb decreases when
View Solution
