An L-C resonant circuit contains a 100μF capacitor an a 400μH inductor. It is not set into oscillation coupled to an antenna. Find the frequency of the radiated electromagnetic waves.
To find the frequency of the radiated electromagnetic waves from the given L-C resonant circuit, we can use the formula for the resonant frequency f of an LC circuit, which is given by:
f=12π√LC
where:
- L is the inductance in henries (H)
- C is the capacitance in farads (F)
Step 1: Convert the given values into standard units
- The capacitance C is given as 100μF=100×10−6F
- The inductance L is given as 400μH=400×10−6H
Step 2: Substitute the values into the resonant frequency formula
Now, substituting the values of L and C into the formula:
f=12π√(400×10−6)(100×10−6)
Step 3: Calculate the product LC
First, we calculate the product LC:
LC=(400×10−6)(100×10−6)=40000×10−12=4×10−8
Step 4: Calculate the square root of LC
Next, we find the square root of LC:
√LC=√4×10−8=2×10−4
Step 5: Substitute back into the frequency formula
Now, substituting this back into the frequency formula:
f=12π(2×10−4)
Step 6: Calculate the frequency
Calculating the denominator:
2π(2×10−4)≈1.25664×10−3
Now, taking the reciprocal:
f≈11.25664×10−3≈796.18Hz
Final Answer
Thus, the frequency of the radiated electromagnetic waves is approximately:
f≈796.18Hz
- L is the inductance in henries (H)
- C is the capacitance in farads (F)
- The capacitance C is given as 100μF=100×10−6F
- The inductance L is given as 400μH=400×10−6H
Now, substituting the values of L and C into the formula:
First, we calculate the product LC:
Next, we find the square root of LC:
Now, substituting this back into the frequency formula:
Calculating the denominator:
Thus, the frequency of the radiated electromagnetic waves is approximately:
Topper's Solved these Questions
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Check point 8.1
10 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Check point 8.2
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
Similar Questions
A L-C resonant circuit contains a 200 pF capacitor and a 100μG inductor. It is set into oscillation coupled to an antenna. The wavelength of the radiated electromagnetic waves is
View SolutionAn L−c resonant circuit contains a 200pF capacitor and a 200μH inductor. It is into oscillation coupled to an antenna. The wavelength of the radiated electromagnetic waves is
View SolutionThe carrier frequency generated by a tank circuit containing 1nF capacitor and 10μH inductor is
View SolutionA resonant AC circuit contains a capacitor of capacitance 10−6F and an inductor of 10−4H. The frequency of electrical oscillation will be
View SolutionAn oscillatory circuit is made up of a 100μμF capacitor and a 64μH coil with resistance of 1.0 ohm. Find the natural frequency, the period of oscillations and the Q-factor of the circuit.
View SolutionAn L-C circuit contains a 0.60 H inductor and a 25μF capacitor. What is rate of change of current when the charges on the capacitor is3.0×10−5C?
View SolutionAn L−C circuit consists of a 20.0mH inductor and a 0.5μF capacitor. If the maximum instantaneous current is 0.1A, what is the greatest potential difference across the capacitor?
View SolutionAnswer the following:
(a) What do you understand by 'sharpness of resonance' for a series LCR resonant circuit? How is it related with the quality factor 'Q' of the circuit? Using the graphs given in the diagram, explain the factors which affect it. For which graph is the resistance (R ) minimum?
(b) A 2μF capacitor, 100Ω resistor and 8H inductor are connected in series with an ac source. Find the frequency of the ac source for which the current drawn in the circuit is maximum.
If the peak value of emf of the source is 200 V, calculate the (i) maximum current, and (ii) inductive and capacitive reactance of the circuit at resonance.
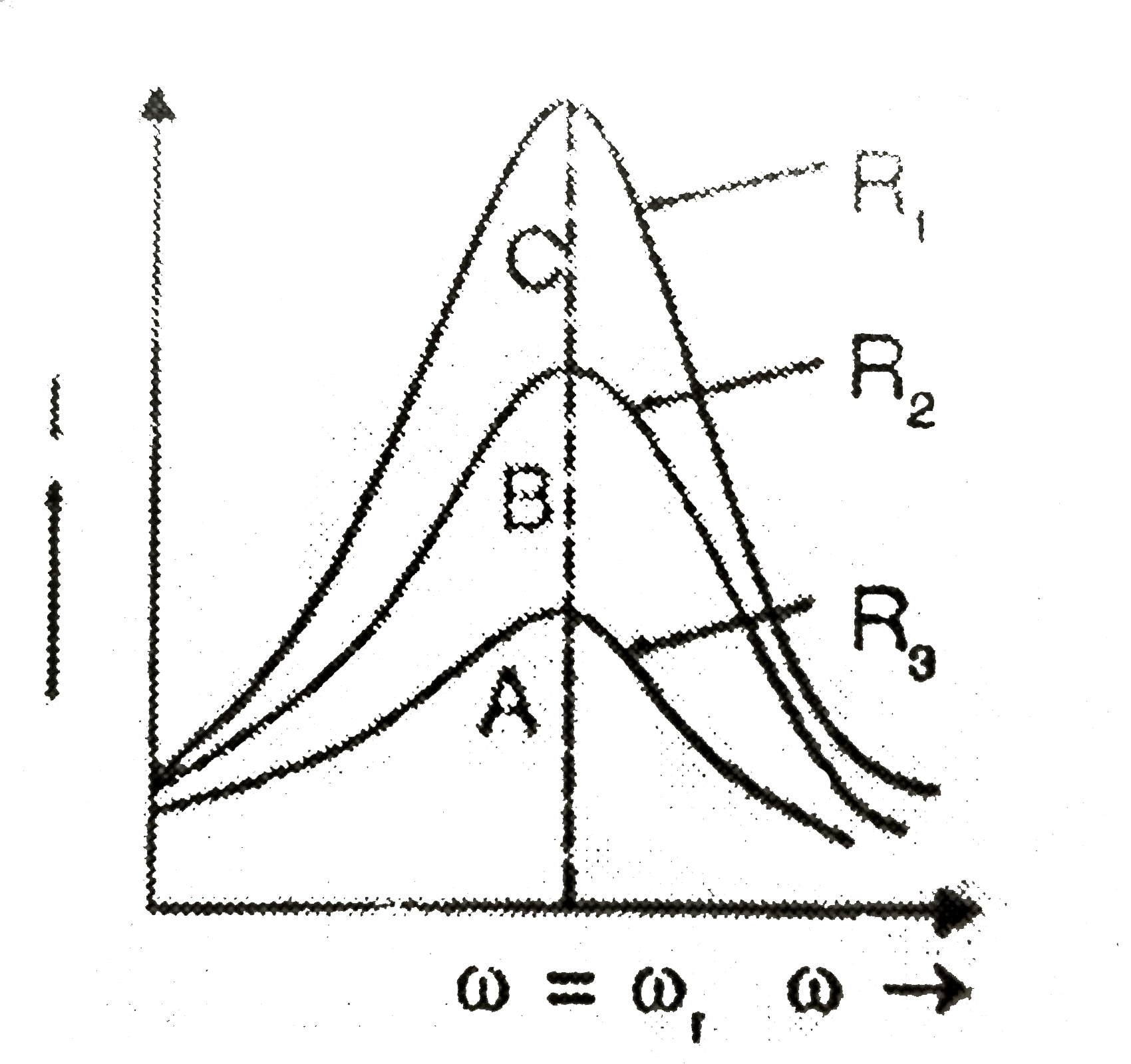
View SolutionA resistor R, an inductor L and a capacitor C are connected in series to an oscillator of frequency n. If the resonant frequency is nr, then the current lags behind voltage, when
View SolutionAn L-C circuit contains 20 mH inductor and a 50μF capacitor with an initial charge of 10 mC. The resistance of the circuit is negligible. Let the instant the circuit is closed be t = 0. what is the total energy stored initially ? At what times is the total energy shared equally between the inductor and the capacitor ?
View Solution
