x≠1 के लिए 1+x+x2+...+xn−1=1−xn1−x हो, तो श्रेणी S=1+2x+3x2+...+(n+1)xn का योगफल ज्ञात कीजिए।
The correct Answer is:(n+1)xn+1−(n+2)xn+1+1(1−x)2
Step by step video, text & image solution for x ne 1 के लिए 1+ x + x ^(2)+...+ x ^(n -1) = (1 - x^n)/( 1 -x) हो, तो श्रेणी S = 1 + 2x + 3x ^(2) + ...+ (n+1) x ^(n) का योगफल ज्ञात कीजिए। by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
फलन f1(x)=⎧⎪⎨⎪⎩1यदिx≤0x2+1यदि0<x<25यदिx≥2,f2(x)=log1/2(x−3),f3(x)=23−x तथा f4(x)=e{x} जहाँ {.} भिन्नात्मक भागफलन को प्रदर्शित करता है, के आरेख निम्न (क्रम में नहीं) है-
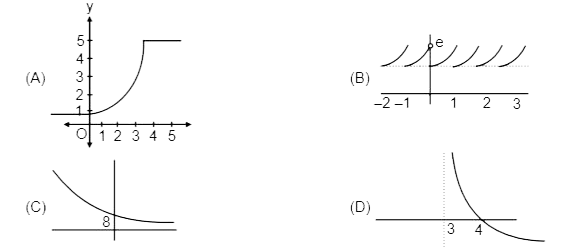
फलन f1(x),f2(x),f3(x) एवं f4(x) के आरेखों का सही क्रम होगा-02:24View Solutionf(x)=cos(sin(ln(x2+ex2+1)))+sin(cos(ln(x2+ex2+1)))
05:38View SolutionIf |x|<1,then1+n(2x1+x)+n(n+1)2!(2x1+x)2+...... is equal to a.(2x1+x)n b. (1+x2x)n c. (1−x1+x)n d. (1+x1−x)n
02:14View SolutionIf |x|<1 then 1+n((2x)/(1+x))+(n(n+1))/(2!)((2x)/(1+x))^(2)+......oo
04:58View SolutionAA n in N, 1 + 2x + 3x ^ (2) ++ ndot x ^ (n-1) = (x in R, x! = 1)
06:54View Solutionit is known for x≠1 that 1+x+x2+…..+xn−1=1−xn1−x, hence find the sum of the series S=1+2x+3x2+…..+(n+1)xn.
06:30View SolutionLet Sn(x)=(xn−1+1xn−1)+2(xn−2+1xn−2)+.....+(n−1)(x+1x)+n, then
04:50View SolutionFrom the relation 1+x+x2+⋅⋅⋅+xn−1=1−xn1−x , find the sum of the series 1+2x+3x2+⋅⋅⋅+(n−1)xn−2 .
03:25View Solutionनिम्न व्यंजक के प्रसार में xn का गुणांक ज्ञात कीजिए|
1−x(1−x)302:27View Solutionदर्शाइए की (1+x1−x)n=1+n(2x1+x)+n(n+1)⌊2(2x1+x)2.............
06:51View Solutionसिद्ध कीजिए कि (1+x1−x)n=1+n(2x1+x)+n(n+1)1.2(2x1+x)2+....
02:24View Solution1+x+x2+.....+xn−1=1−xn1−x সম্বন্ধ থেকে 1+2x+3x2+....+(n−1)xn−2 শ্রেণীটির সমষ্টি নির্ণয় করো।
06:06View SolutionIf |x|<1,then1+n(2x1+x)+n(n+1)2!(2x1+x)2+...... is equal to a.(2x1+x)n b. (1+x2x)n c. (1−x1+x)n d. (1+x1−x)n
04:01View Solution∀n∈,1+2x+3x2+….+n.xn−1=(x∈R,x≠1)
10:41View Solutionit is known for x≠1 that 1+x+x2+…..+xn−1=1−xn1−x, hence find the sum of the series S=1+2x+3x2+…..+(n+1)xn.
09:05View Solution
