If the graph intersects Y-axis below the X-axis then which of the following can be valid?
Roots are real and parabola opens downward
Roots are non-real and parabola opens upward
Roots are real only
If the roots are real then parabola opens upward and if the roots are non-real then parabola opens downward
The correct Answer is:D
To solve the question, we need to analyze the conditions under which a graph intersects the Y-axis below the X-axis. This typically indicates that the value of the function at x=0 (i.e., f(0)) is negative.
Let's break down the options provided:
1. Option A: Roots are real and parabola opens downward.
- If the parabola opens downward and has real roots, it means it will intersect the X-axis at two points and will be above the X-axis between these roots. Therefore, it cannot intersect the Y-axis below the X-axis. This option is not valid.
2. Option B: Roots are non-real and parabola opens downward.
- If the roots are non-real, the parabola does not intersect the X-axis at all. A downward-opening parabola that does not intersect the X-axis will be entirely below the X-axis. Therefore, it will intersect the Y-axis below the X-axis. This option is valid.
3. Option C: Roots are real only.
- This option is incomplete as it does not specify the direction of the parabola. However, if the roots are real, the parabola will intersect the X-axis, which means it cannot be entirely below the X-axis. Thus, this option is not valid.
4. Option D: If roots are real, then the parabola opens upward; if roots are non-real, then the parabola opens downward.
- This statement is true. If the roots are real, the parabola opens upward and intersects the X-axis. If the roots are non-real, the parabola opens downward and does not intersect the X-axis, which means it can intersect the Y-axis below the X-axis. This option is valid.
Now, summarizing the analysis:
- The valid options are B and D.
2. Analyze each option based on the behavior of the parabola.
- Option A: Real roots and downward opening - Not valid.
- Option B: Non-real roots and downward opening - Valid.
- Option C: Real roots only - Not valid.
- Option D: Real roots (upward) or non-real roots (downward) - Valid.
3. Conclude which options are valid.
- The valid options are B and D.
- If the parabola opens downward and has real roots, it means it will intersect the X-axis at two points and will be above the X-axis between these roots. Therefore, it cannot intersect the Y-axis below the X-axis. This option is not valid.
- If the roots are non-real, the parabola does not intersect the X-axis at all. A downward-opening parabola that does not intersect the X-axis will be entirely below the X-axis. Therefore, it will intersect the Y-axis below the X-axis. This option is valid.
- This option is incomplete as it does not specify the direction of the parabola. However, if the roots are real, the parabola will intersect the X-axis, which means it cannot be entirely below the X-axis. Thus, this option is not valid.
- This statement is true. If the roots are real, the parabola opens upward and intersects the X-axis. If the roots are non-real, the parabola opens downward and does not intersect the X-axis, which means it can intersect the Y-axis below the X-axis. This option is valid.
- The valid options are B and D.
- Option A: Real roots and downward opening - Not valid.
- Option B: Non-real roots and downward opening - Valid.
- Option C: Real roots only - Not valid.
- Option D: Real roots (upward) or non-real roots (downward) - Valid.
- The valid options are B and D.
Topper's Solved these Questions
Similar Questions
The graph of (y−x) against (y+x) is shown below.
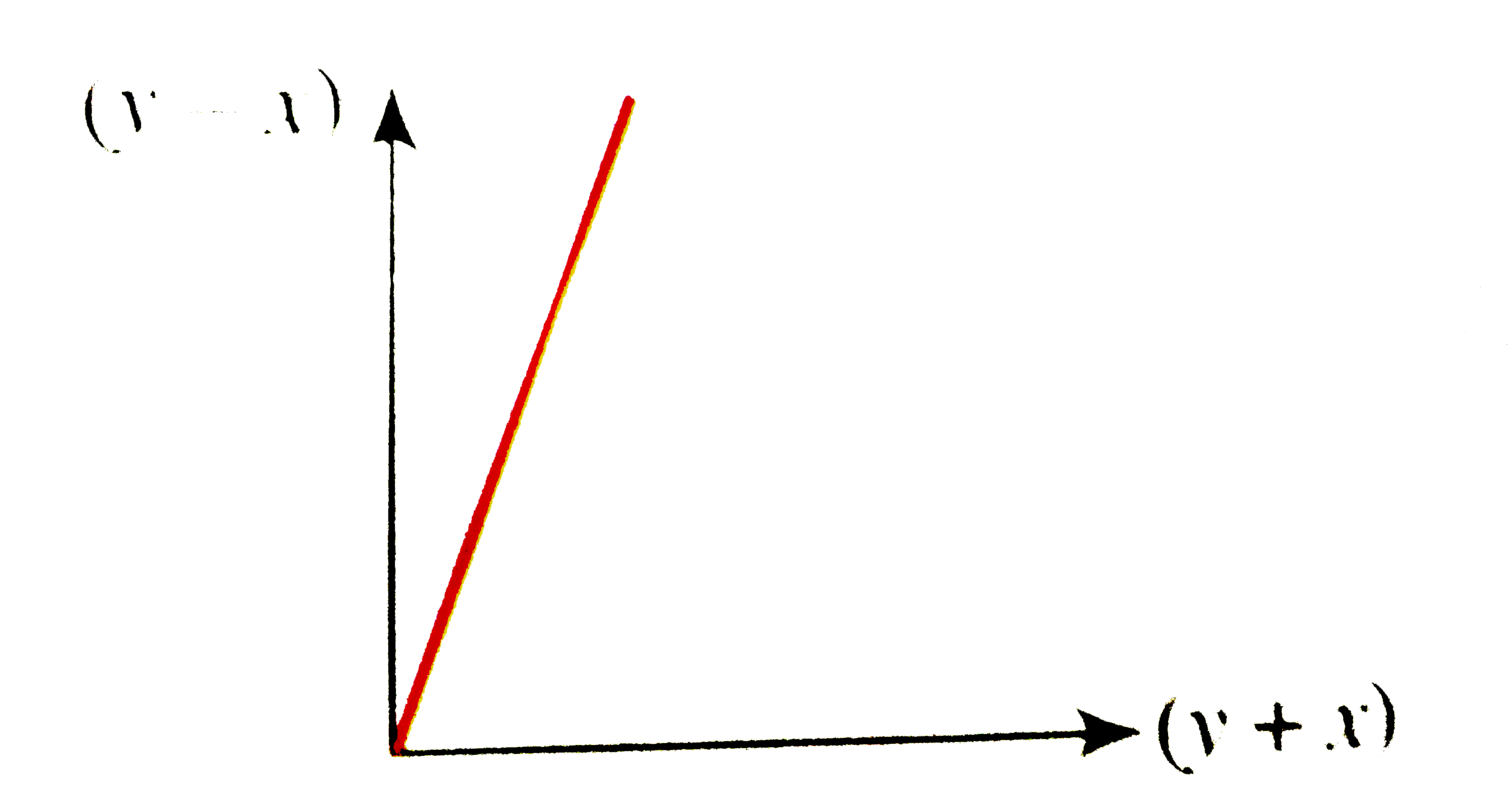
Which one of the following shows the graph of y against x ?
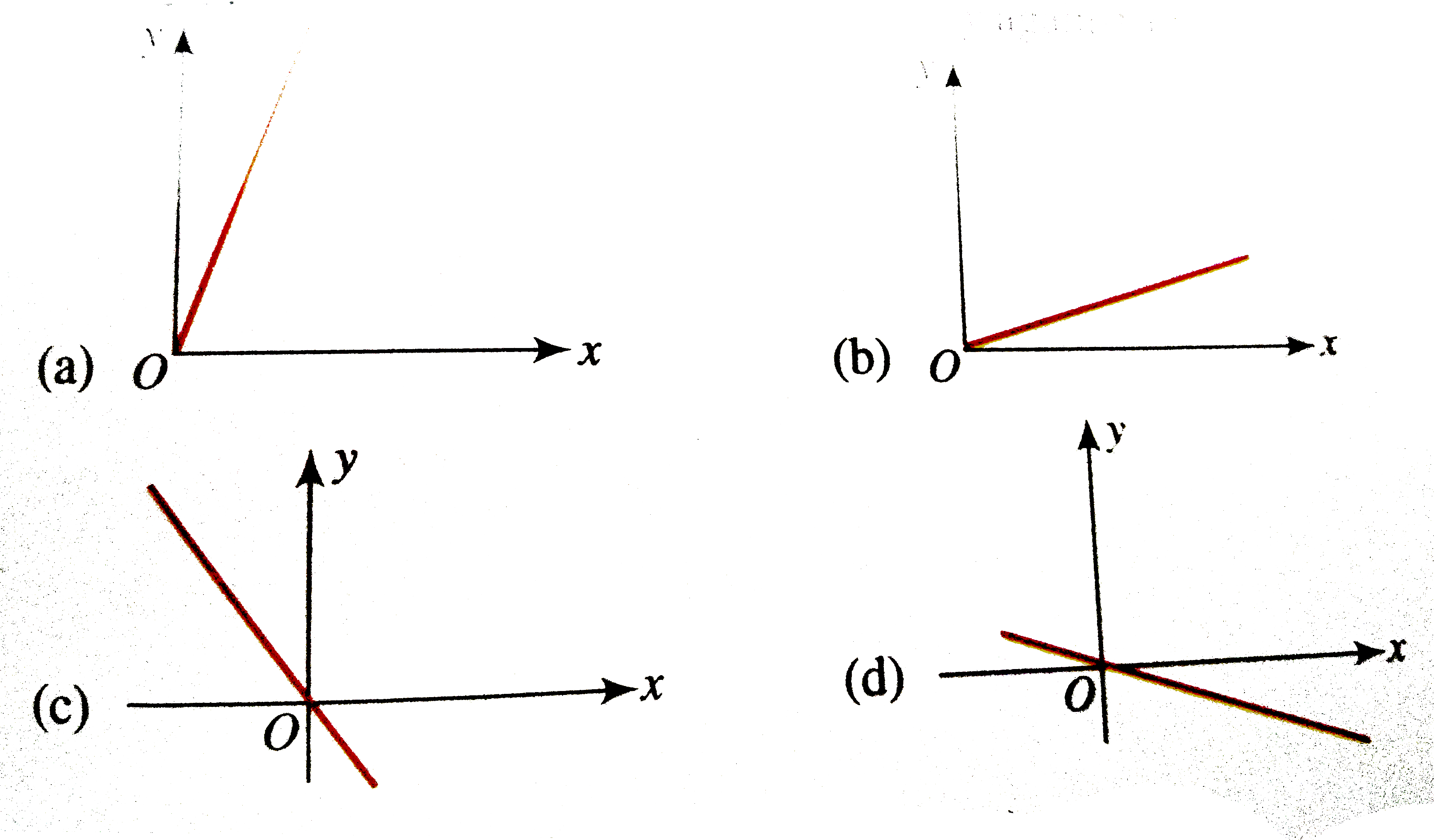
View SolutionGiven the graphs of the two functions, y=f(x)&y=g(x). In theadjacent figure from point A on the graph of the function y fx)corresponding to the given value of the independent variable (say x),a straight line is drawn parallel to the X-axis to intersect the bisectorof the first and the third quadrants at point B. From the point B astraight line parallel to the Y-axis is drawn to intersect the graph ofthe function y=g(x) at C. Again a straight line is drawn from the point C parallel to the X-axis, to intersect the line NN' at D. If thestraight line NN' is parallel to Y-axis, then the co-ordinates of the point D are
View Solution
Knowledge Check
When the parabola never intersects the X-axis then which of the following is certainly true?
AD>0Ba<0CD = 0Db2≤4acIf the graph intersects X-axis at two distinct points, then,the roots are necessarily
AcomplexBirrationalCrationalDrealThe parabola intersects the X-axis at most
A4 timesB3 timesC2 timesD1 time
Find the equation of the line which intersects the y-axis at a distance of 2 units above the origin and makes an angle of 300 with the positive direction of the x-axis.
View SolutionThe graph of a quadratic equation is symmetrical about X-axis, which ones of the following can't be the roots of a quadratic equation?
View SolutionThe tangent to the hyperbola xy=c2 at the point P intersects the x-axis at T and the y-axis at T. The normal to the hyperbola at P intersects the x-axis at N and the y-axis at N. The areas of the triangles PNT and PNT are Δ and Δ respectively, then
1Δ+1Δ is
View SolutionThe intersection point on the x-axis of 7x−2y=2 is
View SolutionSuppose R is relation whose graph is symmetric to both X-axis and Y-axis and that the point (1,2) is on the graph of R. Which one of the following is not necessarily on the graph of R?
View Solution
