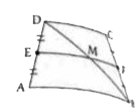
ABCD is a trapezium . AB ||CD and AB:CD=5:4, E and F are mid points of AD and BC, if CD= 20 cm , Find EF
The correct Answer is:19:17
To find the length of EF in trapezium ABCD where AB || CD and the ratio AB:CD = 5:4, and given that CD = 20 cm, we can follow these steps:
Step 1: Understand the Ratio
The ratio of AB to CD is given as 5:4. This means that if we let the length of AB be 5x and the length of CD be 4x, we can express both lengths in terms of a variable x.
Step 2: Find the Value of x
Given that CD = 20 cm, we can set up the equation:
4x=20
To find x, we divide both sides by 4:
x=204=5
Step 3: Calculate the Length of AB
Now that we have the value of x, we can find the length of AB:
AB=5x=5×5=25 cm
Step 4: Use the Midpoint Theorem
In a trapezium, the line segment joining the midpoints of the non-parallel sides (EF in this case) is given by the formula:
EF=AB+CD2
Step 5: Substitute the Values
Now we can substitute the values of AB and CD into the formula:
EF=25 cm+20 cm2
Step 6: Calculate EF
Now, we perform the addition and division:
EF=45 cm2=22.5 cm
Final Answer
Thus, the length of EF is:
EF=22.5 cm
---
The ratio of AB to CD is given as 5:4. This means that if we let the length of AB be 5x and the length of CD be 4x, we can express both lengths in terms of a variable x.
Given that CD = 20 cm, we can set up the equation:
4x=20
To find x, we divide both sides by 4:
x=204=5
Now that we have the value of x, we can find the length of AB:
AB=5x=5×5=25 cm
In a trapezium, the line segment joining the midpoints of the non-parallel sides (EF in this case) is given by the formula:
EF=AB+CD2
Now we can substitute the values of AB and CD into the formula:
EF=25 cm+20 cm2
Now, we perform the addition and division:
EF=45 cm2=22.5 cm
Thus, the length of EF is:
EF=22.5 cm
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
ABCD is a trapezium , in which AD∣∣BC, E and F are the mid-point of AB and CD respectively , then EF is :
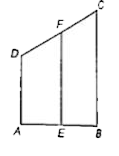 A(AD+BC)2B(AB+CD)2C(DF xx CF)/(AE xx BE)DAD+EF+BC2
A(AD+BC)2B(AB+CD)2C(DF xx CF)/(AE xx BE)DAD+EF+BC2 - Question 2 - Select One
ABCD is a trapezium with AB || CD. If AB = 10 cm, CD = 7 cm and area of trapezium = 102 cm2, then find the height of trapezium (in cm).
AA)10BB)12CC)14DD)16 - Question 3 - Select One
ABCDis a trapezium, such that AB=CD and AD∣∣BC.AD=10cmandBC=18cm. If the area of ABCDis 70cm2, what is the value (in cm) of CD?
A5B√29C√41D6
ABCD is a cyclic trapezium & ABII CD, AB is diameter. BC = 2cm, AB = 8 cm, CD = ?
View SolutionIn the given figure ABCD is a parallelogram in which AB||CD and E and F are the mid points of AB and BC respectively. What is the area of ∠BEF?
 View Solution
View SolutionABCD is a trapezium in which AB∣∣CD,AB=20cm,BC=10 cm, CD=10 cm and AD=10 cm . Find ∠ADC
View SolutionABCD is a trapezium, such that AB = CD and AD|BC. AD = 5 cm, BC = 9 cm. If area of ABCD is 35 sq.cm, then CD is :
View SolutionABCD is a trapezium, such that AB = CD and AD || BC. AD = 5cm, BC = 9cm. If area of ABCD is 35 sq.cm then CD is :
View Solution