A physical balance has its arms of length 60cm and 40cm. What weight kept on the pan of the longer arm will balance an object of weight 100 gf kept on the other pan?
66.67gf
Topper's Solved these Questions
- BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1B11videos
FORCE
- BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1(B) Multiple choice questions2videos
FORCE
- BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1(A) Multiple choice questions2videos
FORCE
- BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS1videos
ELECTRO-MAGNETISM
- BOOK - ICSECHAPTER - HOUSEHOLD CIRCUITS EXERCISE - EXERCISE -9(B) (MULTIPLE CHOICE TYPE)2videos
HOUSEHOLD CIRCUITS
Similar Questions
A physical balance has its arms of unequal length. A body weight 18 kg if kept in one pan and weight 8 kg. If kept in the other pan. The true weight of the body is
View SolutionThe two arms of a balanced are of unequal length. An object when placed in the left pan of the balance weighs 4 kg. The same object when placed in the right pan of the balance weighs 9 kg. The actual (or) true mass of the object is
View Solution
Knowledge Check
- Question 1 - Select One
Find the weight of half meter scale balanced at 5 cm mark when a weight of 10 gf is suspended at one of its ends.
A2.5gfB100gfC0.2gfD50gf
A 4 m long rod of negligible weight is supported at a point 125 cm from its one end and a load of 18 kgf is suspened at a point 60 cm from the support on the shorter arm.
(a) If a weight W is placed at a distance 250 cm from the support on the longer arm to balance the rod, find W.
(b) If a weight 5 kgf is kept to balance the rod, find its position.
(c) To which class of lever does it belong ?
View SolutionIn a physical balance working on the principle of moments, when 5 mg weight is placed on the left pan, the beam becomes horizontal. Both the empty pans of the balance are of equal mass. Which of the following statements is correct ?
View SolutionIn a physical balance working on the principle of moments, when 5 mg weight is placed on the left pan, the beam becomes horizontal. Both the empty pans of the balance are of equal mass. Which of the following statements is correct ?
View SolutionWhen the switch is open in lowerpoint loop of a potentiometer, the balance point length is 60cm. When the switch is closed with a known resistance of R=4Ω the balance point length decreases to 40cm. Find the internal resistance of the unknown battery.
View SolutionIf the eye is kept very close to a coverging lens (focal length =10cm) and at the optical centre of the lens and an object is kept at distance 'd' of the object from the lebs so that its image can be seen clearly by the defect free eye is :
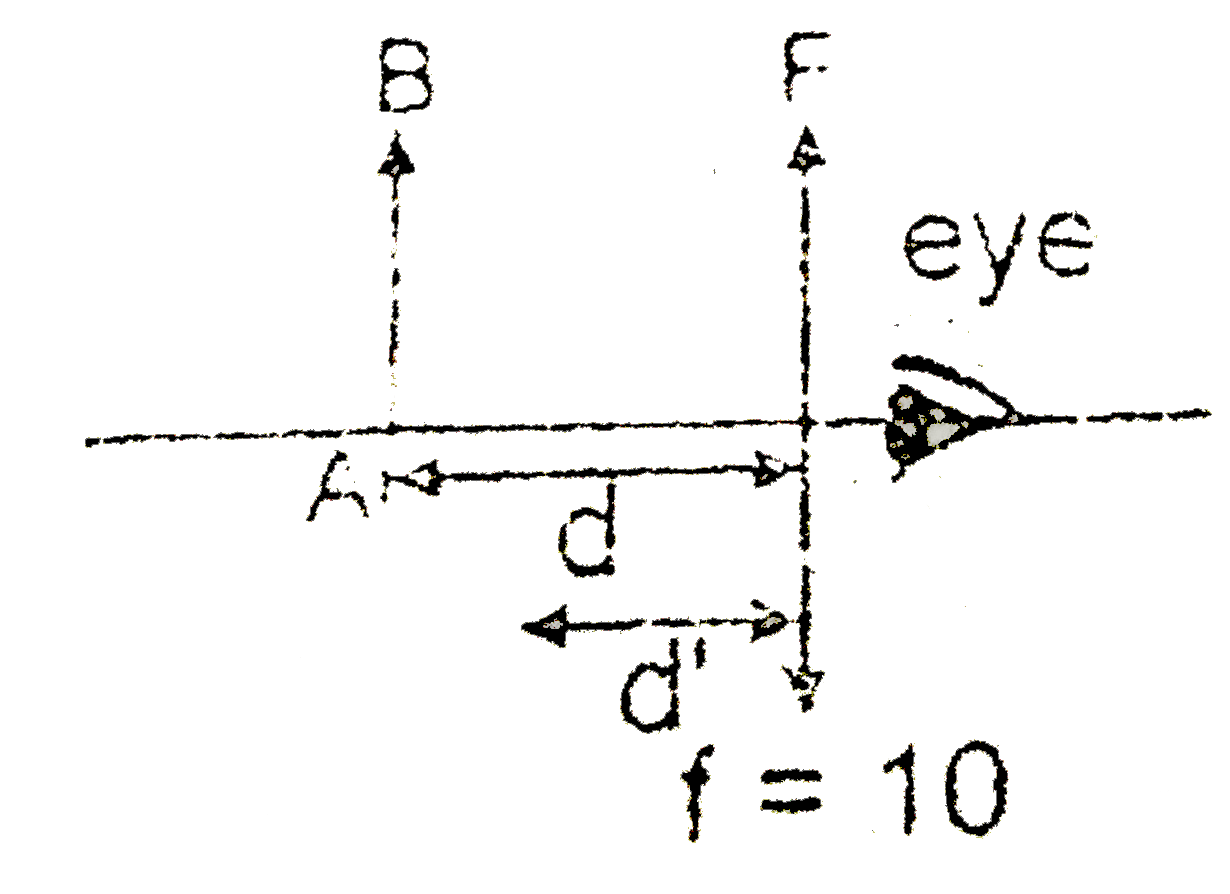 .View Solution
.View SolutionIf the eye is kept very close to a coverging lens (focal length =10cm) and at the optical centre of the lens and an object is kept at distance 'd' of the object from the lebs so that its image can be seen clearly by the defect free eye is :
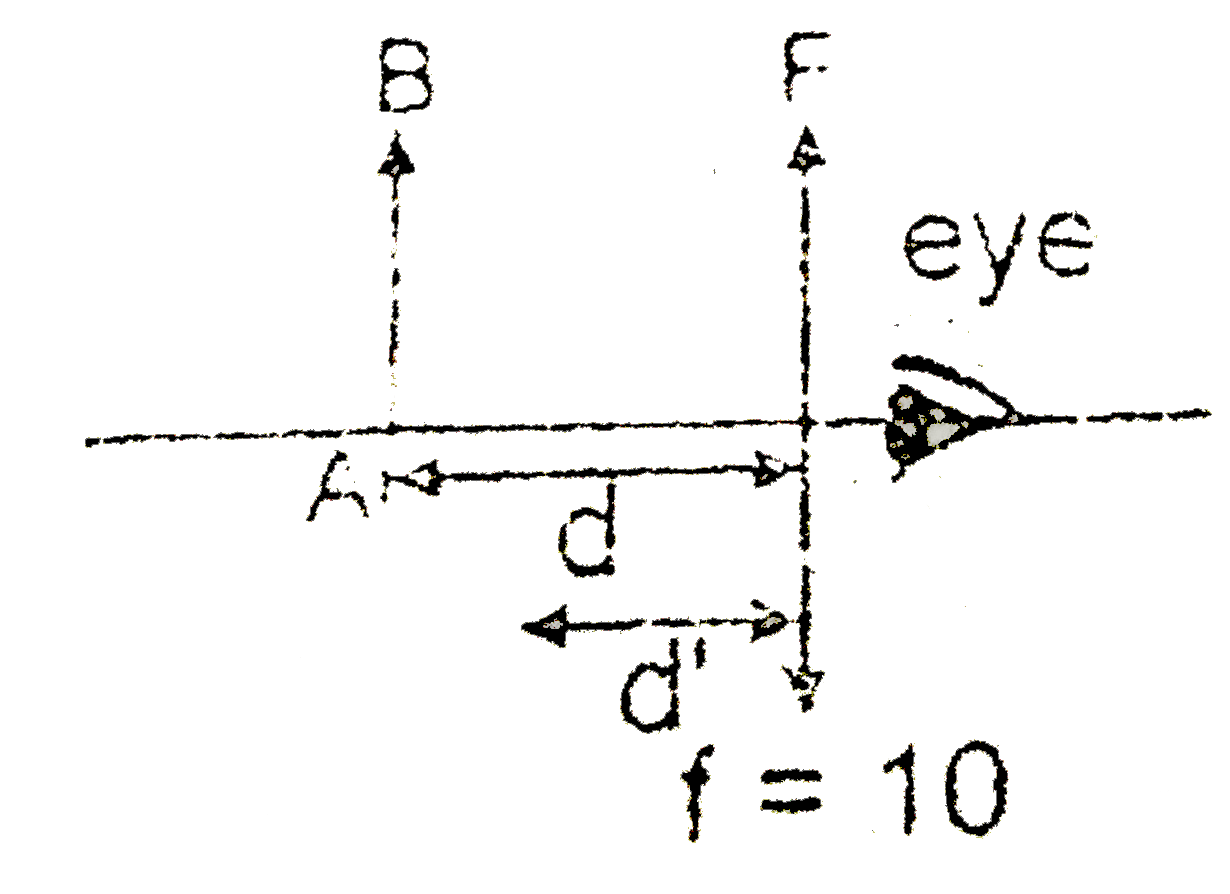 .View Solution
.View SolutionA beam balance of unequal arm length is used by an unscruplus trader. When an object is weighed on left pan. The weight i found to be W1. When the object is weighed on the right plane, the weight is found to be W2. If W1≠W2, the correct weight of the object is
View Solution
