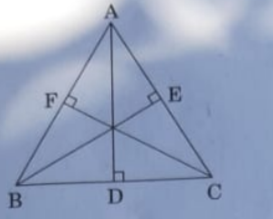
In a triangle ABC, AB = BC, AD is perpendicular to side BC and CE is perpendicular to side AB. Prove that : AD = CE.
To prove that AD=CE in triangle ABC where AB=BC, AD is perpendicular to BC, and CE is perpendicular to AB, we can follow these steps:
1. Draw Triangle ABC:
- Start by sketching triangle ABC with vertices A, B, and C.
- Label the sides such that AB=BC.
2. Draw Perpendiculars:
- Draw a perpendicular line AD from vertex A to side BC, meeting BC at point D.
- Draw another perpendicular line CE from vertex C to side AB, meeting AB at point E.
3. Identify Congruent Triangles:
- Now, we will consider triangles ABD and CBE.
- We know that AB=BC (given).
- Angle B is common to both triangles ABD and CBE.
- The angles ∠ADB and ∠CEB are both right angles (90 degrees) because AD and CE are perpendicular to BC and AB respectively.
4. Apply the Criteria for Congruence:
- We have:
- AB=BC (given)
- ∠ADB=∠CEB=90∘ (both are right angles)
- ∠ABD=∠CBE (common angle)
- By the Angle-Side-Angle (ASA) criterion for triangle congruence, we can conclude that △ABD≅△CBE.
5. Conclude the Equality of Heights:
- Since the triangles ABD and CBE are congruent, it follows that their corresponding parts are equal.
- Therefore, AD=CE.
Final Statement:
Thus, we have proved that AD=CE.
- Start by sketching triangle ABC with vertices A, B, and C.
- Label the sides such that AB=BC.
- Draw a perpendicular line AD from vertex A to side BC, meeting BC at point D.
- Draw another perpendicular line CE from vertex C to side AB, meeting AB at point E.
- Now, we will consider triangles ABD and CBE.
- We know that AB=BC (given).
- Angle B is common to both triangles ABD and CBE.
- The angles ∠ADB and ∠CEB are both right angles (90 degrees) because AD and CE are perpendicular to BC and AB respectively.
- We have:
- AB=BC (given)
- ∠ADB=∠CEB=90∘ (both are right angles)
- ∠ABD=∠CBE (common angle)
- By the Angle-Side-Angle (ASA) criterion for triangle congruence, we can conclude that △ABD≅△CBE.
- Since the triangles ABD and CBE are congruent, it follows that their corresponding parts are equal.
- Therefore, AD=CE.
Thus, we have proved that AD=CE.
Topper's Solved these Questions
- TRIANGLES BOOK - ICSECHAPTER - TRIANGLES EXERCISE - QUESTIONS
8 Videos
- TRIANGLES BOOK - ICSECHAPTER - TRIANGLES EXERCISE - 3 MARKS QUESTIONS
11 Videos
- TRIANGLES BOOK - ICSECHAPTER - TRIANGLES EXERCISE - 4 MARKS QUESTIONS
24 Videos
- STATISTICS AND GRAPH WORK BOOK - ICSECHAPTER - STATISTICS AND GRAPH WORK EXERCISE - TOPIC - 2 (4 Marks Questions)
14 Videos
- TRIGONOMETRICAL RATIOS BOOK - ICSECHAPTER - TRIGONOMETRICAL RATIOS EXERCISE - EXERCISE 22(B)
50 Videos
Similar Questions
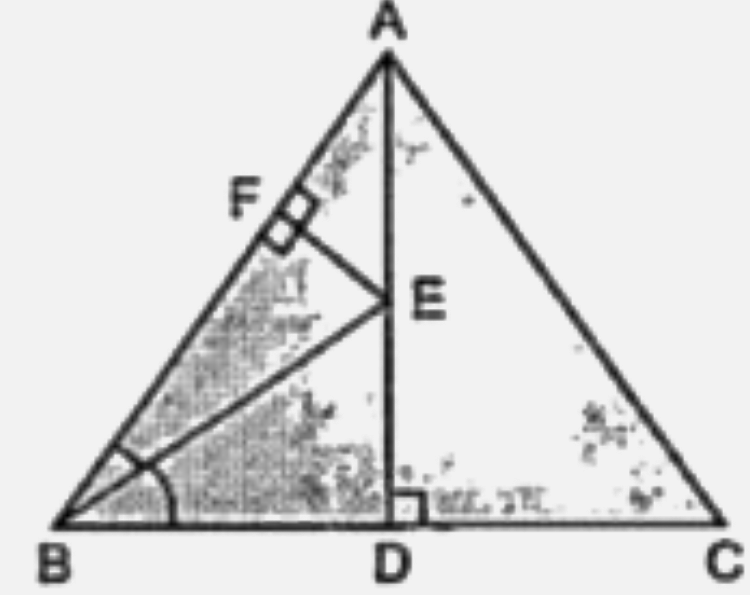
In the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB.
Prove that : ED=EF
View SolutionIn the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB.
Prove that :
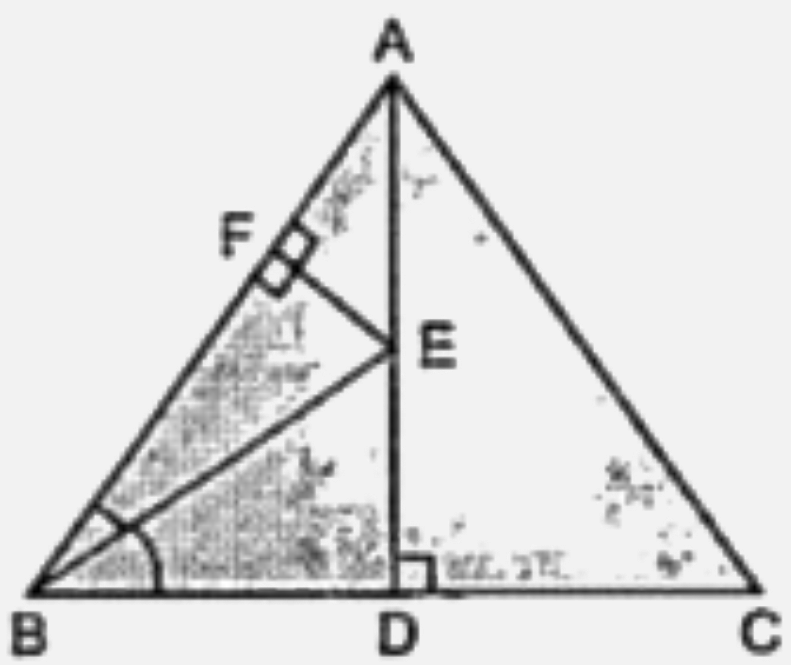
ED=EF
View SolutionIn Figure -6 , in an equilateral triangle ABC, AD is perpendicular to BC , BE is perpendicular to AC and CF is perpendicular to AB . Prove that 4(AD2+BE2+CF2)=9AB2
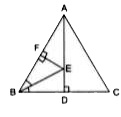
View SolutionIn the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB. Prove that :
(i) BD = CD
(ii) ED = EF
View SolutionIn triangle ABC, AB > AC. E is the mid-point of BC and AD is perpendicular to BC. Prove that : AB2+AC2=2AE2+2BE2
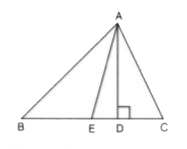
View SolutionThe given figure shows a triangle ABC, in which AB > AC. E is the mid-point of BC and AD is perpendicular to BC.
Prove that : AB2+AC2=2BE2+2AE2
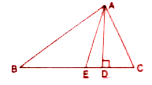
View SolutionThe given figure shows a triangle ABC, in which AB>AC. E is the mid-point of BC and AD is perpendicular to BC. Prove that AB2−AC2=2BC×ED.
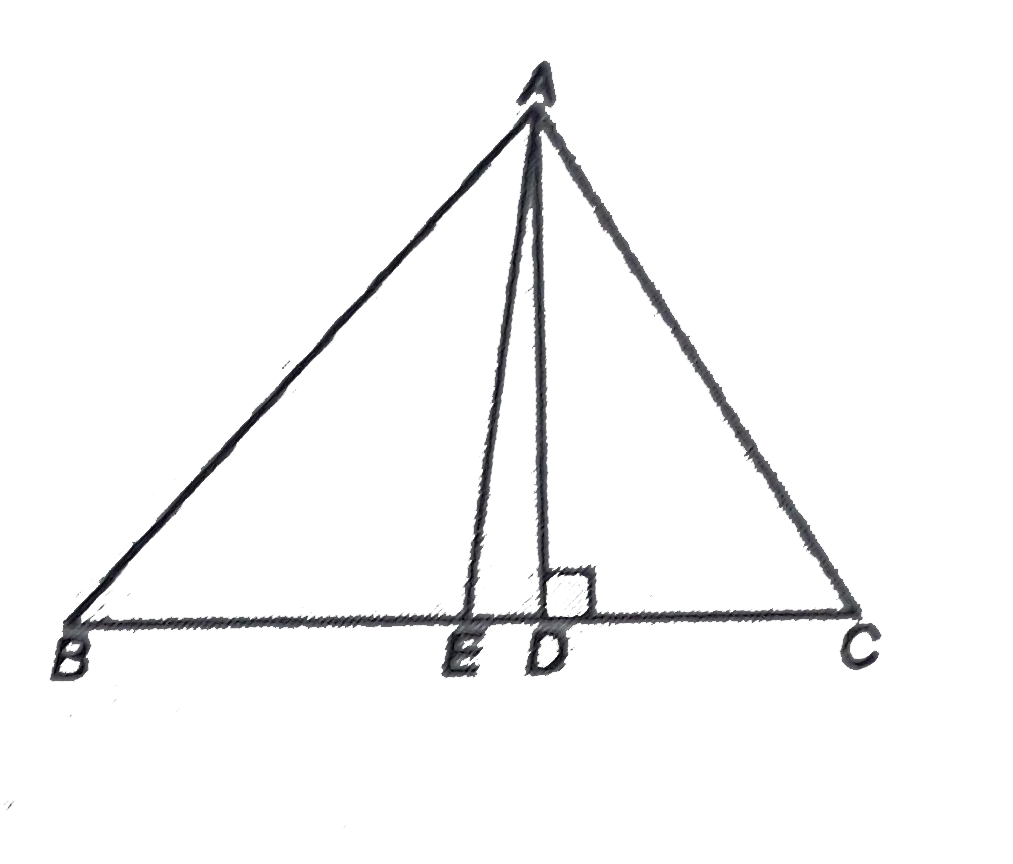
View SolutionIn the figure AB = BC and AD is perpendicular to CD. Prove that :
AC2=2.BC.DC
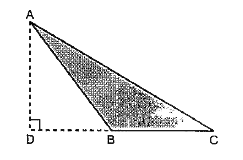
View SolutionABC is a right angled triangle with ABC=90∘. D is any point on AB and DE is perpendicular to AC. Prove that:

If AC = 13 cm, BC = 5 cm and AE = 4 cm. Find DE and AD.
View SolutionIn the figure, AD is perpendicular to BC, prove that AB+AC>2AD.

View Solution
