Introduce the following definition: the length of a moving rod is the product of its speed and the time interval between the moments when its two ends pass a static clock. llhe proper length is determined in a similar way with the aid of a clock moving at the same speed along a static rod. Find the relation between the length of a moving rod l and its proper length l0.
The correct Answer is:l=l0√1−β2
The length of a rod movfog wilh respect to the reference frame is l=rt0, where τ0 is the time Interval between the instants the front and the tail ends of the rod pass the clock at rest in the reference frame. On the other hand, the length of a stationary rod is measured with the aid of moving clocks: l0=vτ
Hence, ll0=τ0τ=√1−v2/c2, therefore l=l0√1−v2/c2
which is the same result as is given hy the Lorentz transformation.
Similar Questions
If the speed of a rod moving at a relativistic speed is parllel , to its length is doubled,
View SolutionTwo rods of the same proper length l0 move toward each other parallel to a common horizontal axis. In the reference frame fixed to one of the rods the time interval between the moments, when the right and left ends of the rods coincide, is equal to Δt. What is the velocity of one rod relative to the other?
View Solution
Knowledge Check
A metal rod of length I is moved with velocity v in a uniform magnetic field →B. Emf is induced between the two ends of rod
Awhen →l is parallel to →BBwhen →l is parallel to →vCwhen →v is parallel to →BDnone of the above cases
A rod moves lengthwise with a constant velocity v relative to the inertial reference frame K. At what value of v will the length of the rod in this frame be η=0.5% less than its proper length?
View SolutionA rod of length l is moving in a vertical plane (x−y) when the lowest point A of the rod is moved with a velocity v. find the a angular velocity of the rod and b velocity of the end B.

View SolutionA rod od length l is moving in a vertical plane (x−y) when the owest point A of the rod is moved with a velociy v. find the a angular velocity of the rod and b velocity of the end B.

View SolutionA rod AB oriented along the x axis of the reference frame K moves in the positive direction of the x axis with a constant velocity v. The point A is the forward end of the rod, and the point B its near end. Find:
(a) the proper length of the rod, if at the moment tA the coorniate of the point A is equal to xA, and at the moment tB the coordinate of the point B is equal to xB,
(b) what time interval should separate the markings of coordinates of the rod's ends in the frame K for the difference of coordinates to become equal to the proper length of the rod.
View SolutionTwo rods having the same proper length l0 move lengthwise toward each other parallel to a common axis with the same velocity v relative to the laboratory frame of reference. What is the length of each rod in the reference frame fixed to the other rod?
View SolutionA uniform rod of length l is spinning with an angular velocity ω=2vl while its centre of mass moves with a velocity v. Find the velocity of the end of the rod.
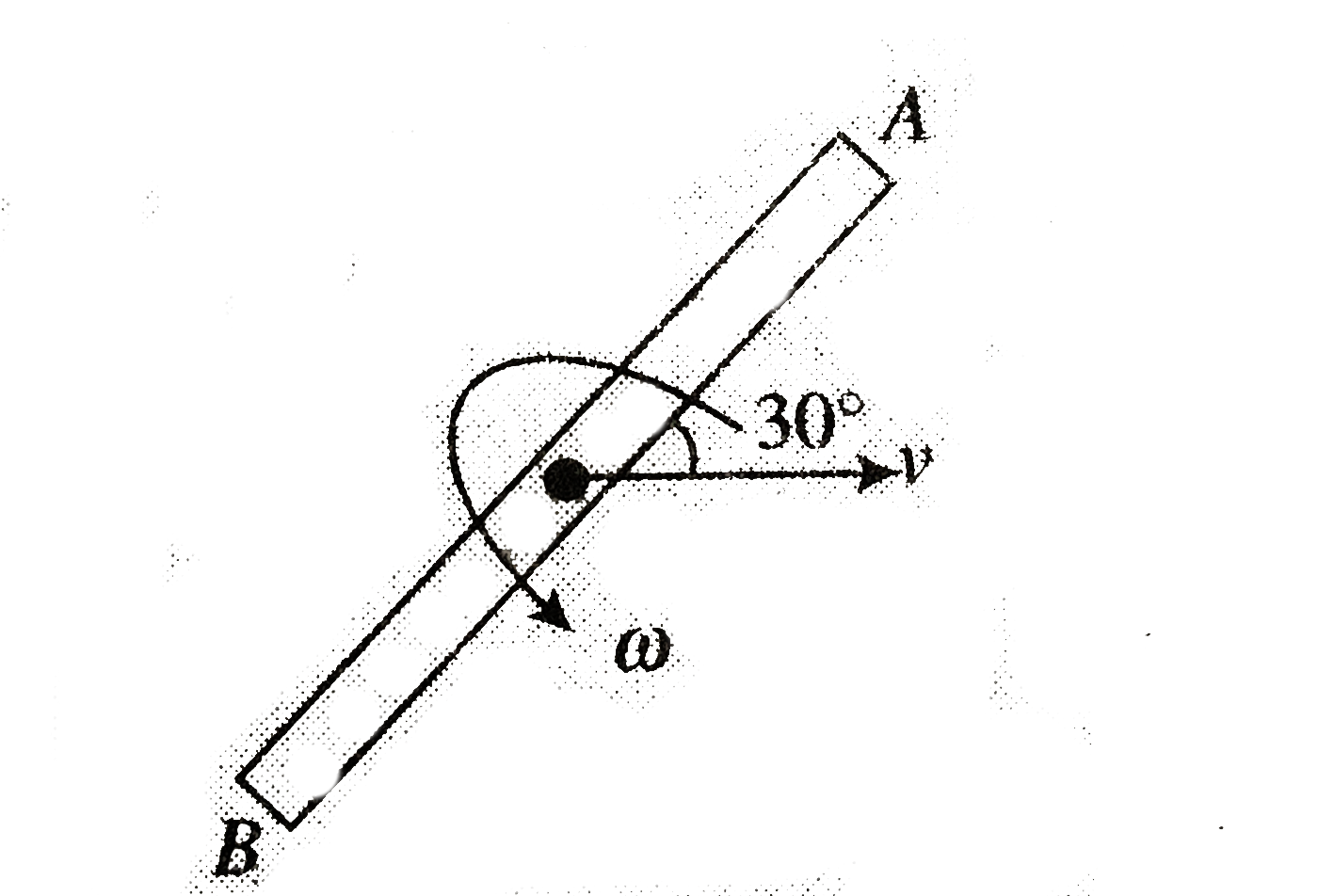
View SolutionA uniform rod of length l is spinning with an angular velocity ω=2vl while its centre of mass moves with a velocity v. Find the velocity of the end of the rod.
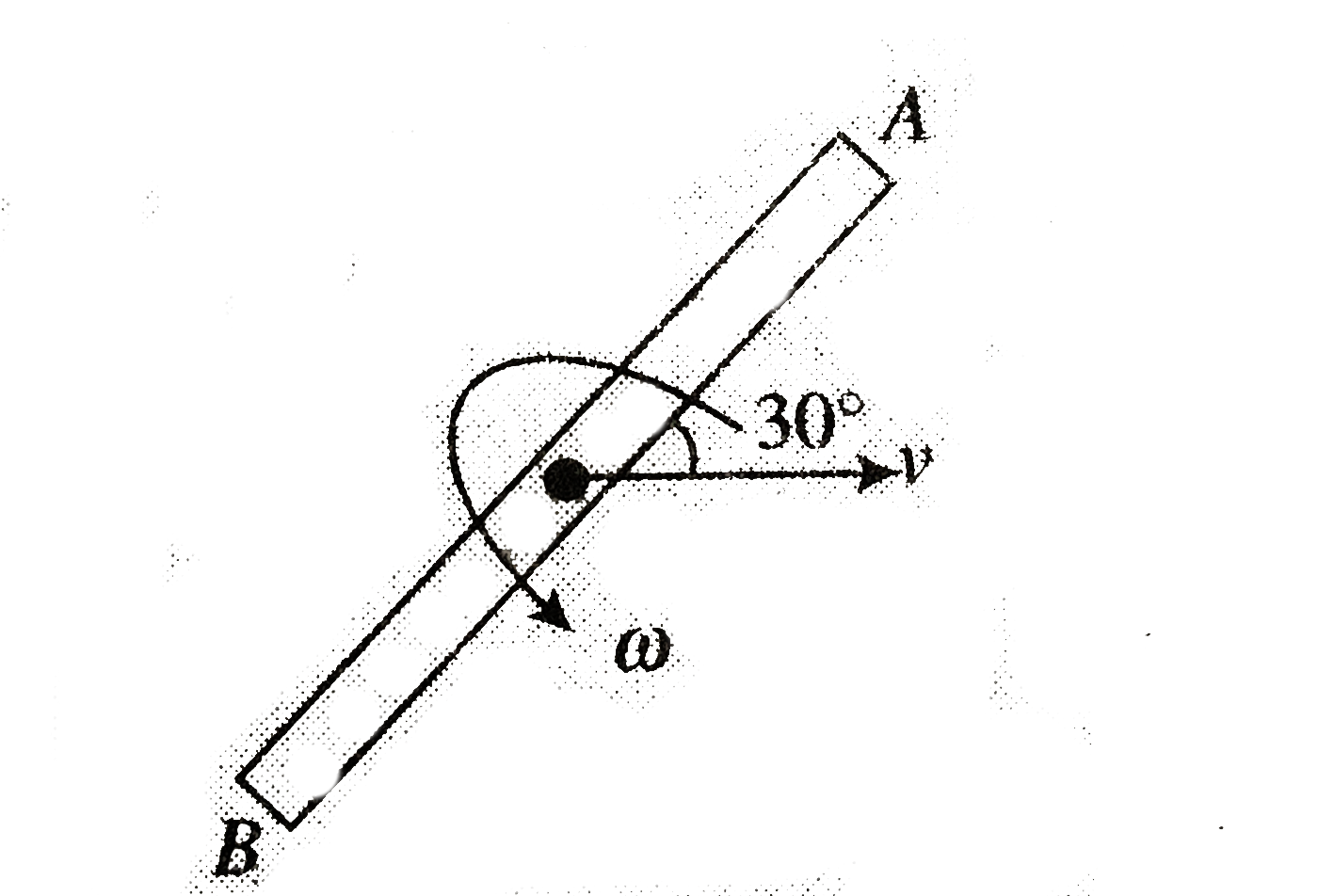
View Solution
