A relativistic proton with kinetic energy K collides with a stationary proton. Assuming the collision to he elastic and the energy to be partitioned equally between the particles, find the scattering angle. Calculate for the cases K = = 500 MeV and K = 10 GeV.
The correct Answer is:83∘;43∘
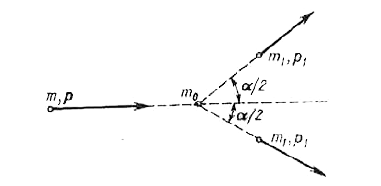
Since the energy is equipartitiened between the particles, the same will happen lo the momenta. Consequently, after the collisions the protons scatter at equal angles to the original direction of the proton projectile (see Fig. 10.6). From the laws of conservation of total momentum and of total kinetic energy we obtain
2p1cosα2=p,2K1=K
From the relation between the energy and the momentum E2=
=E20+p2c2 we obtain, nothing that E=E0+K
K2+2KE0=p2c2,K21+2K1E0=p21c2
Eliminating the momenta and the proton.s kinetic energy after collision, K1, from these equations, we obtain
cos2(α)2=2E0+K4E0+K
whence
cosα=2cos2(α)2−1=K4E0+K
Note that in the nonrelativistic case, when K<<E0 , we shall have cosα≈0 and α≈π2.
For K = 500 MeV we obtain, noting that E0=938MeV,cosα=0.117,α=0.46π.
For K = 10 GeV we obtain cosα:=0.728,α:=0.24π. We see that as the kinetic energy of the projectile particle rises, the scattering angle decreases, approaching zero for ultra-relativistic particles (K>>E0).
Similar Questions
An electron of kinetic energy K collides elastically with a stationary hydrogen atom in the ground state. Then,
View SolutionA neutron collides elastically with an initially stationary deuteron. Find the fraction of the kinetic energy lost by the neutron (a) in a head-on collision , (b) in scattering at right angles.
View Solution
Knowledge Check
A proton with kinetic energy K, strikes another proton at rest. If the collision is head-on, find the correct graph between K and the distance of closest approach, r.
A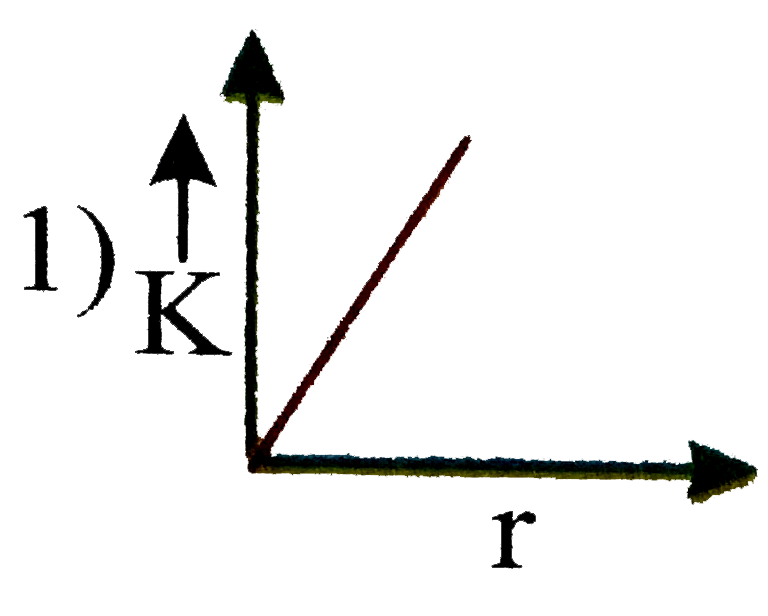 B
B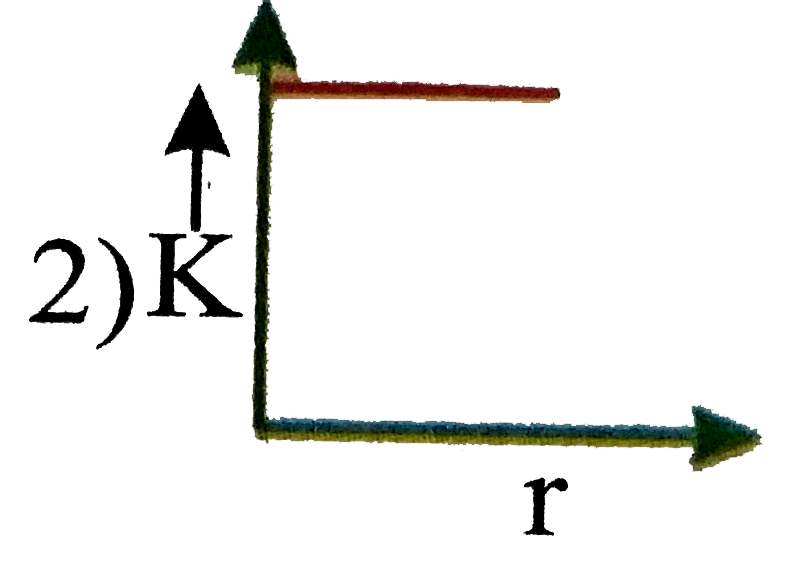 C
C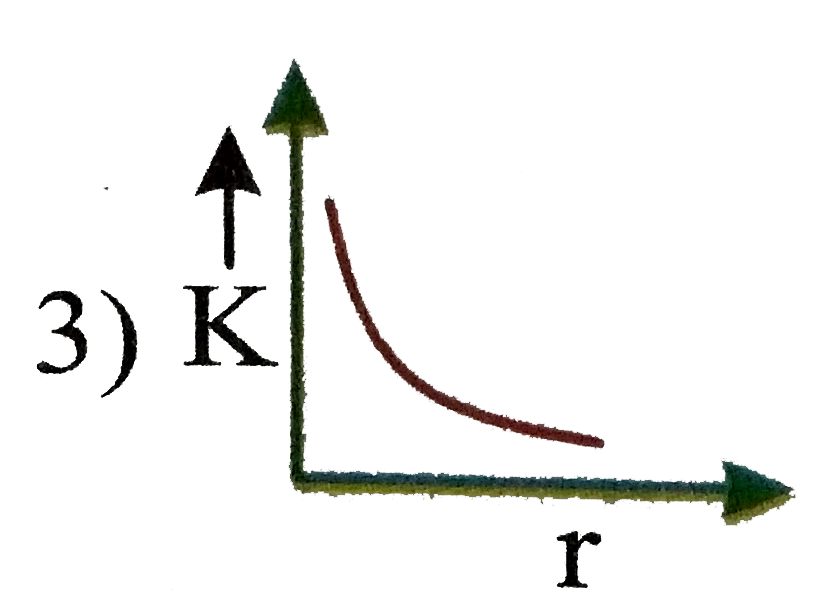 D
D
A particle of mass m moving with kinetic energy K, makes a head - on elastic collision with a stationary particle of mass ηm. The maximum potential energy stored in the system during the collision is
Aηη+1KBη+1ηKC(η−1)KD(η−1)KThe relation between kinetic energy K and linear momentum p of a particle is represented by
AK2=2mpB2K2=mpCp2=2mkD2mK=p
An α-particle with kinetic energy K is heading towards a stationary nucleus of atomic number Z. Find the distance of the closest approach.
View SolutionA neutron collides elastically with an initially stationary deuteron. Find the fraction of the kinetic energy lost by the neutron in a head-on collision?
View SolutionA neutron collides elastically with an initially stationary deuteron. Find the fraction of the kinetic energy lost by the neutron
(a)in a head-on collision
(b) in scatterting at right angles.
View SolutionA particle moving with kinetic energy K makes a head on elastic collision with an identical particle at rest. Find the maximum elastic potential energy of the system during collision.
View SolutionA particle moving with kinetic energy K makes a head on elastic collision with an identical particle at rest. Find the maximum elastic potential energy of the system during collision.
View Solution
