A monkey of mass 20kg is holding a vertical rope. The rope will not break when a mass of 25kg is suspended from it but will break it the mass exeeds 25kg . What is the maximum acceleration with which the monkey can climb up along the rope? (g=10m/s2) .
25m/s2
2.5m/s2
5m/s2
10m/s2
The correct Answer is:B
To solve the problem step by step, we will analyze the forces acting on the monkey and use Newton's second law of motion.
Step 1: Identify the forces acting on the monkey
The forces acting on the monkey are:
1. The gravitational force (weight) acting downward: Wm=mm⋅g
2. The tension T in the rope acting upward.
Where:
- mm=20kg (mass of the monkey)
- g=10m/s2 (acceleration due to gravity)
Step 2: Calculate the weight of the monkey
Using the formula for weight:
Wm=mm⋅g=20kg⋅10m/s2=200N
Step 3: Determine the maximum tension in the rope
The problem states that the rope can hold a maximum mass of 25kg before breaking. Therefore, the maximum tension Tmax in the rope is:
Tmax=mmax⋅g=25kg⋅10m/s2=250N
Step 4: Apply Newton's second law
According to Newton's second law, the net force acting on the monkey when it climbs up with acceleration a is given by:
T−Wm=mm⋅a
Substituting the known values:
T−200N=20kg⋅a
Step 5: Substitute the maximum tension into the equation
Using the maximum tension calculated:
250N−200N=20kg⋅a
This simplifies to:
50N=20kg⋅a
Step 6: Solve for the maximum acceleration a
Now, we can solve for a:
a=50N20kg=2.5m/s2
Conclusion
The maximum acceleration with which the monkey can climb up along the rope is:
2.5m/s2
The forces acting on the monkey are:
1. The gravitational force (weight) acting downward: Wm=mm⋅g
2. The tension T in the rope acting upward.
- mm=20kg (mass of the monkey)
- g=10m/s2 (acceleration due to gravity)
Using the formula for weight:
Wm=mm⋅g=20kg⋅10m/s2=200N
The problem states that the rope can hold a maximum mass of 25kg before breaking. Therefore, the maximum tension Tmax in the rope is:
Tmax=mmax⋅g=25kg⋅10m/s2=250N
According to Newton's second law, the net force acting on the monkey when it climbs up with acceleration a is given by:
T−Wm=mm⋅a
Substituting the known values:
T−200N=20kg⋅a
Using the maximum tension calculated:
250N−200N=20kg⋅a
This simplifies to:
50N=20kg⋅a
Now, we can solve for a:
a=50N20kg=2.5m/s2
The maximum acceleration with which the monkey can climb up along the rope is:
2.5m/s2
Similar Questions
A monkey of 25 kg is holding a vertical rope. The rope does not break if a body of mass less than 30 kg is suspended with the rope. What will be the maximum acceleration with which the monkey can climb up along the rope ? (Take g=10ms−2)
View SolutionA body of mass 60 kg is holding a vertical rope . The rope can break when a mass of 75 kg is suspended from it. The maximum acceleration with which the boy can climb the rope without breaking it is :(g=10m/s2)
View SolutionA rope of negligible mass passes over a pulley of negligible mass attached to the ceiling, as shown in figure. One end of the rope is held by Student A of mass 70kg, who is at rest on the floor. The opposite end of the rope is held by Student B of mass 60kg, who is suspended at rest above the floor. The minimum acceleration a0 with which the Student B should climb up the rope to lift the Student A upward off the floor. (g=10m/s2)
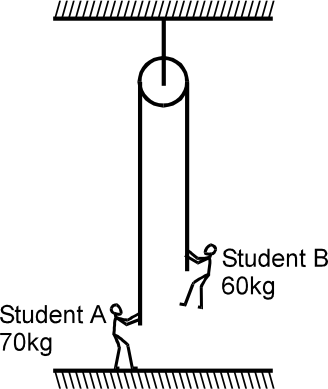
View SolutionA rope of negligible mass passes over a pulley of negligible mass attached to the ceiling, as shown in figure. One end of the rope is held by Student A of mass 70kg, who is at rest on the floor. The opposite end of the rope is held by Student B of mass 60kg, who is suspended at rest above the floor. The minimum acceleration a0 with which the Student B should climb up the rope to lift the Student A upward off the floor. (g=10m/s2)

View SolutionA monkey of mass 20 kg climbs on a rope which can stand a maximum tension of 300 N. In which of the following case will the rope break . The monkey
View SolutionA money of mass 40 kg climbs up a rope, of breaking load 600 N hanging from a ceiling. If it climbs up the rope with the maximum possible acceleration, then the time taken by monkey to climb up is [length of rope is 10 m]
View SolutionThe maximum tension a rope can withstand is 60 kg.wt. The ratio of maximum acceleration with which two boys of masses 20 kg and 30 kg can climb up the rope at the same time is
View SolutionA boy of mass 40 kg climbs up a rope with an acceleration of 2ms−2 . What is the tension in the rope ?
View SolutionA 50 kg man stuck in flood is being lifted vertically by an army helicopter with the help of light rope which can bear a maximum tension of 70 kg-wt. The maximum acceleration with which helicopter can rise so that rope does not breaks is (g=9.8ms−2)
View SolutionA can of paint with a mass of 6 kg hangs from a rope. If the can is to be pulled up to a rooftop with an acceleration of 1m/s, what must be the tension in the rope ?
View Solution
