In the given diagram ABCD is a parallelogram. ΔAPD and ΔBQC are equilateral triangles.
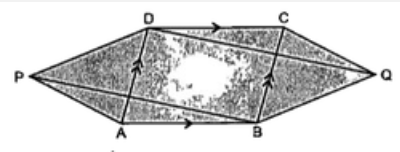
Prove that :
PB=QD
The correct Answer is:PB=QD
Step by step video & image solution for In the given diagram ABCD is a parallelogram. DeltaAPD and DeltaBQC are equilateral triangles. Prove that : PB=QD by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLES BOOK - ICSECHAPTER - TRIANGLES EXERCISE - 3 MARKS QUESTIONS
11 Videos
- STATISTICS AND GRAPH WORK BOOK - ICSECHAPTER - STATISTICS AND GRAPH WORK EXERCISE - TOPIC - 2 (4 Marks Questions)
14 Videos
- TRIGONOMETRICAL RATIOS BOOK - ICSECHAPTER - TRIGONOMETRICAL RATIOS EXERCISE - EXERCISE 22(B)
50 Videos
Similar Questions
In the given diagram ABCD is a parallelogram. ΔAPD and ΔBQC are equilateral triangles.
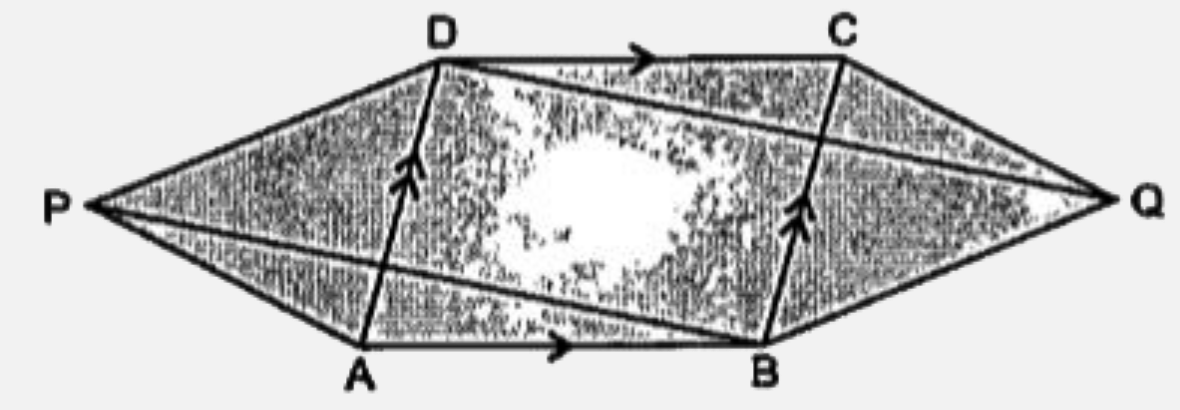
Prove that :
∠PAB=∠QCD
View SolutionIn the given figure ABCD is a parallelogram. Prove that AB = 2BC.
View SolutionIn the given figure, ABCD is a parallelogram. Find the area of ΔAED
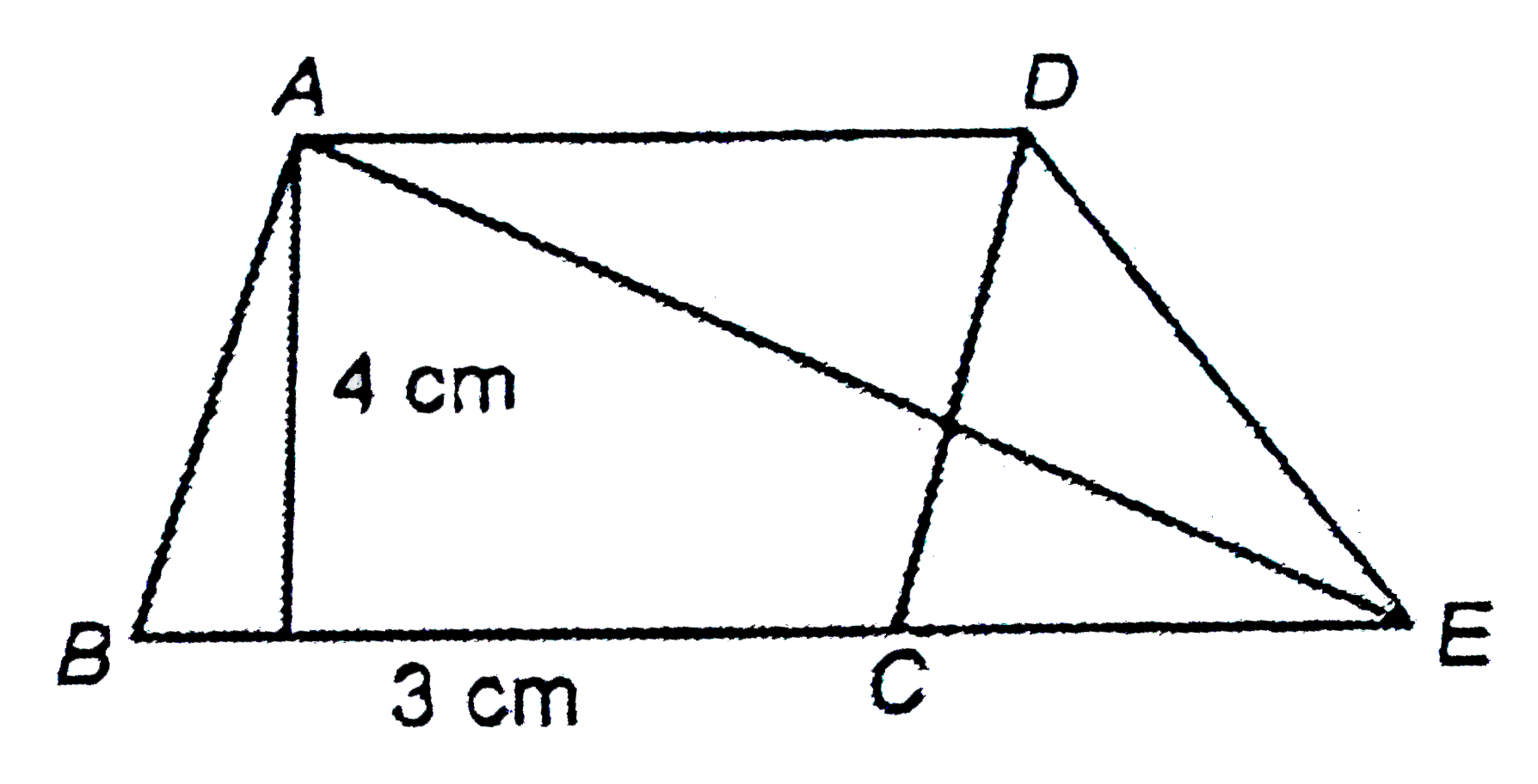
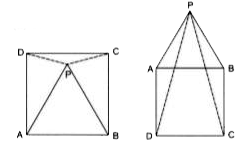
View SolutionIn the following diagrams, ABCD is a square and APB is an equilateral triangle. In each case,
(i) Prove that : ΔAPD≅ΔBPC
(ii) Find the angles of ΔDPC
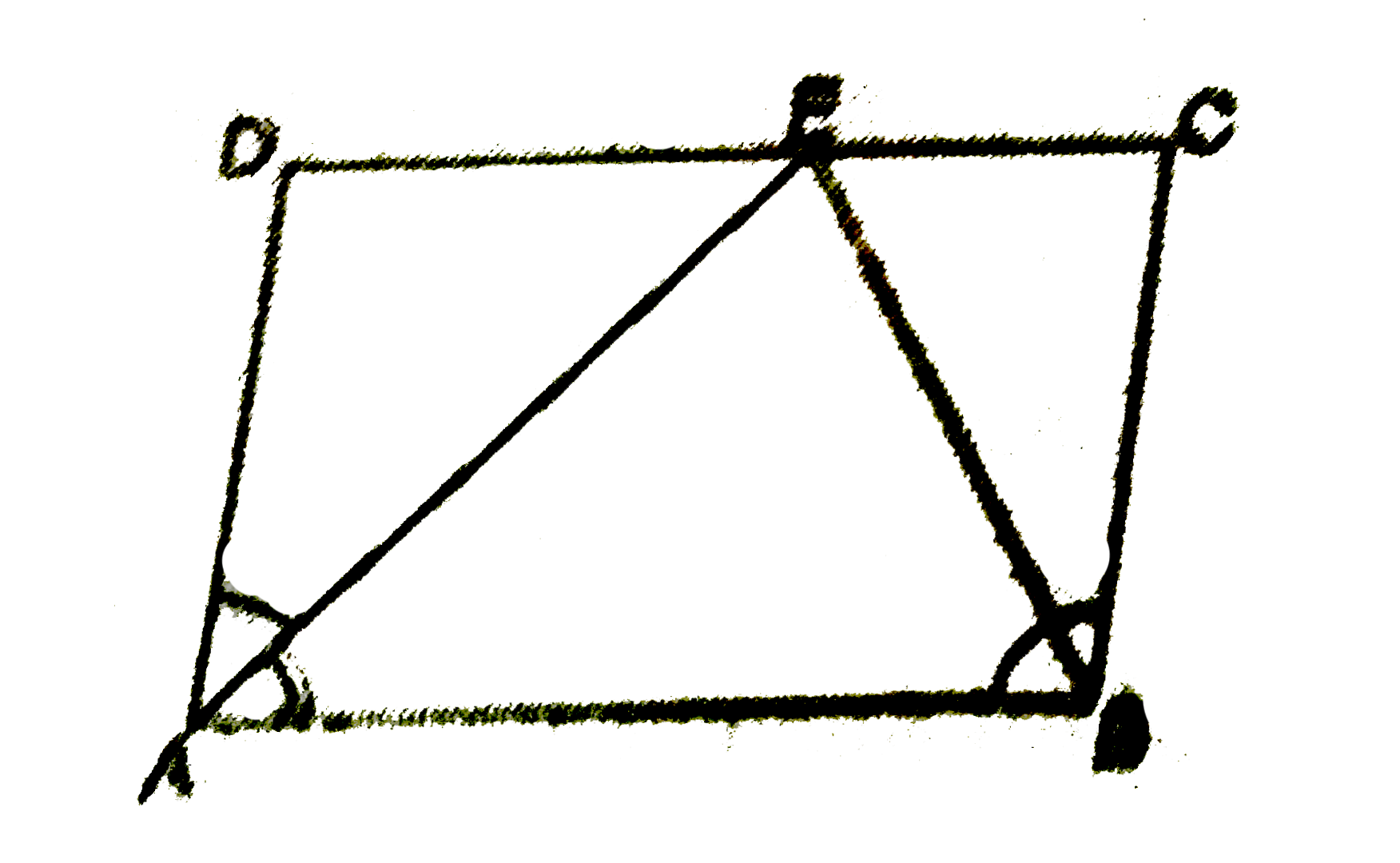
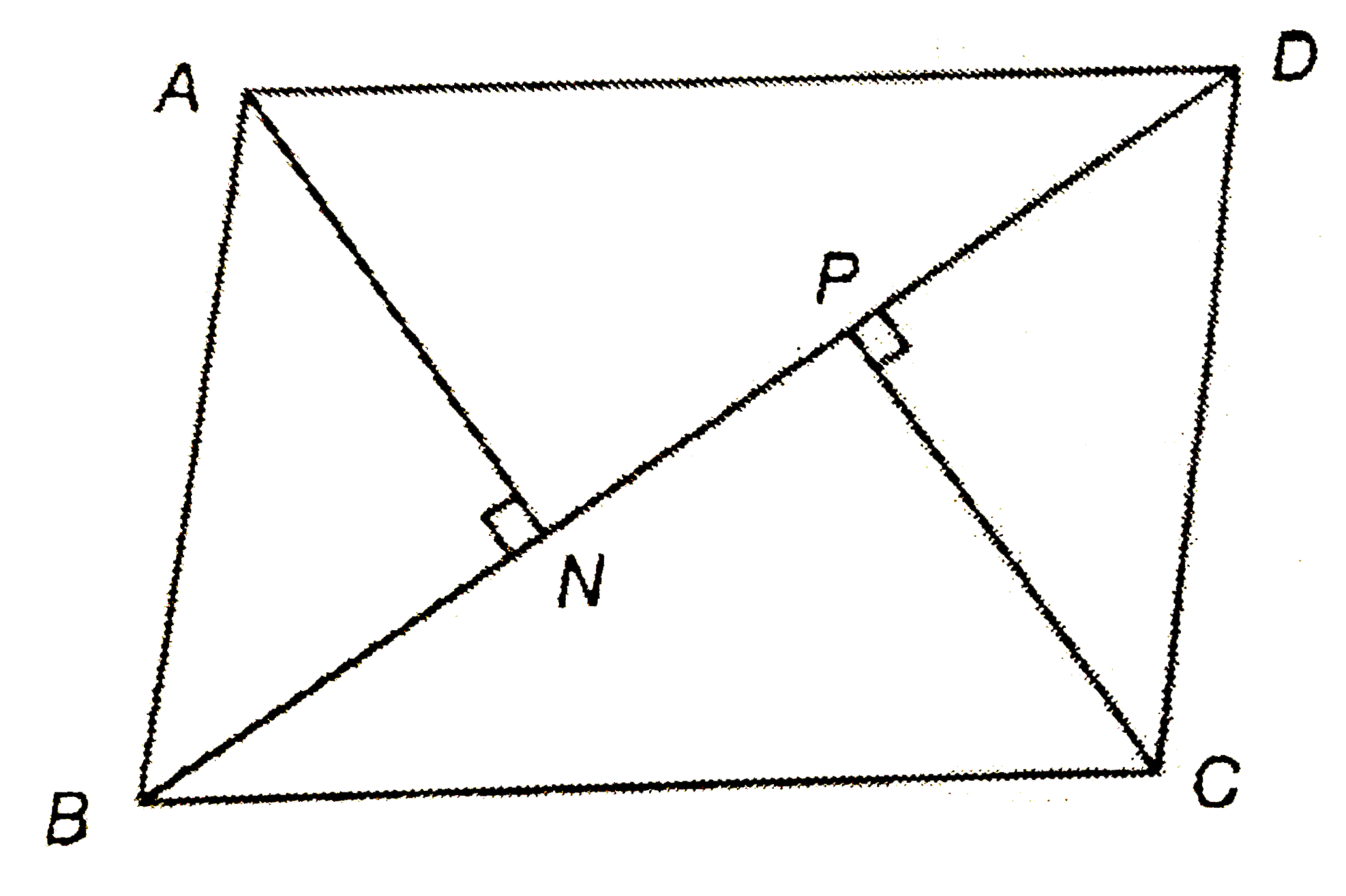
View SolutionIn the given figure, ABCD is a parallelogram in which AN and CP are perpendiculars on diagonal BD. Prove that :
(i) ΔADN=ΔCBP
(ii)AN=CP
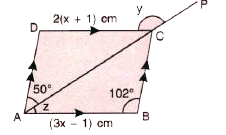
View SolutionIn the given figure, ABCD is a parallelogram. Find the values of x, y and z
View SolutionIn the following figure, ABCD is a parallelogram. Prove that:

BP bisects angle B
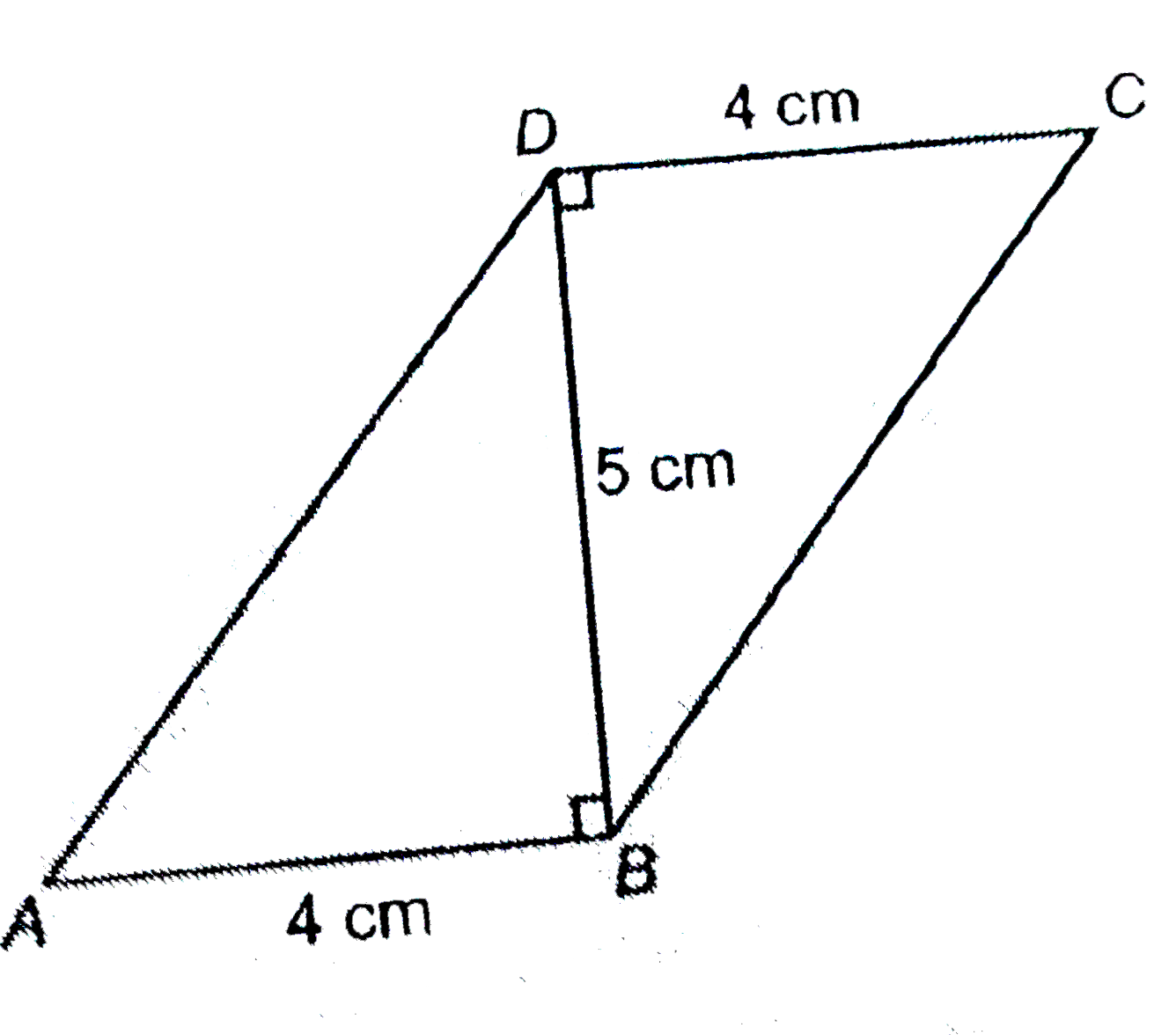
View SolutionIn the adjoining figure, prove that ABCD is a parallelogram. Also find its area.
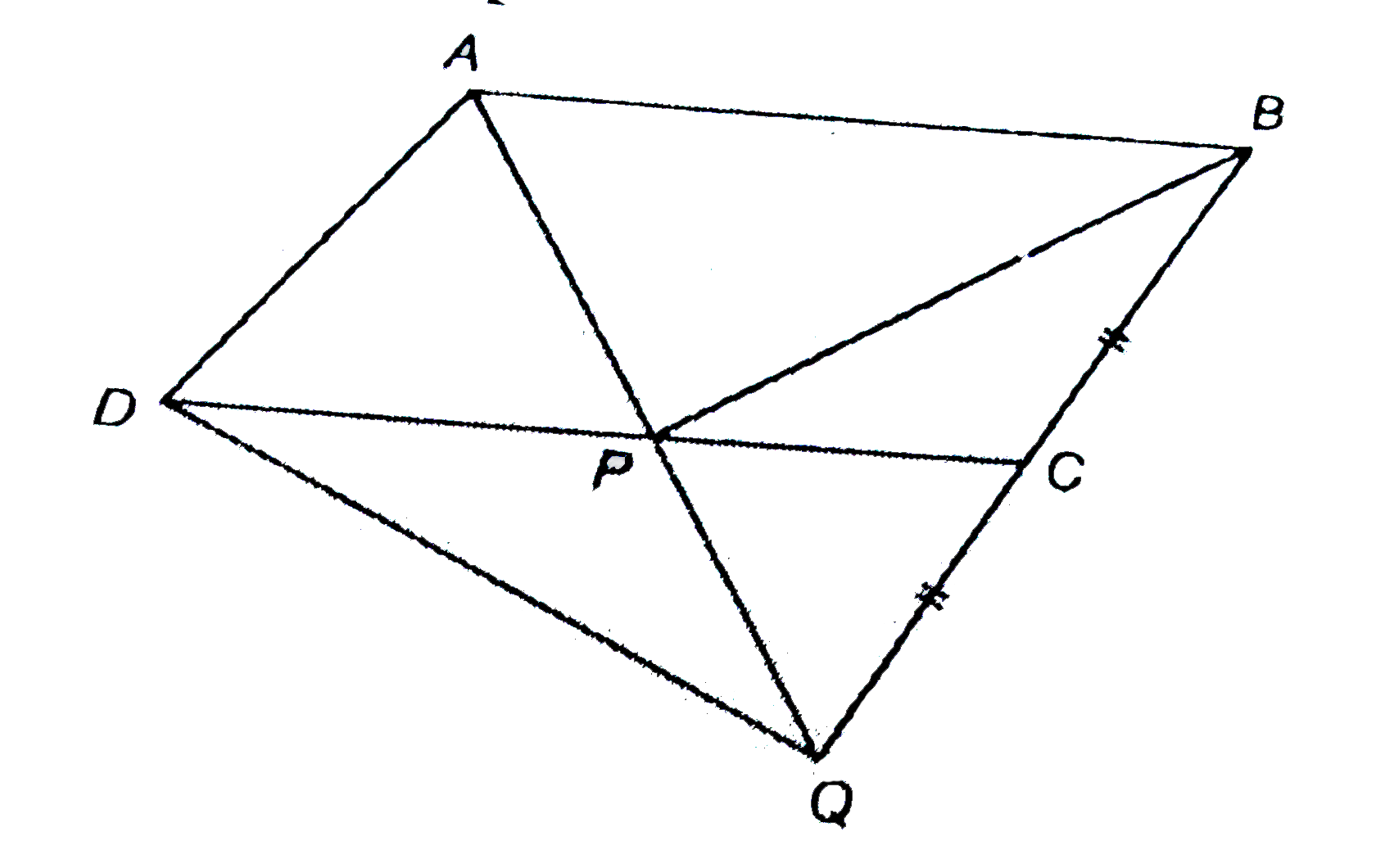
View SolutionIn the adjoning figure, ABCD is a parallelogram. Prove that : area of ΔBPC= area of ΔDPQ
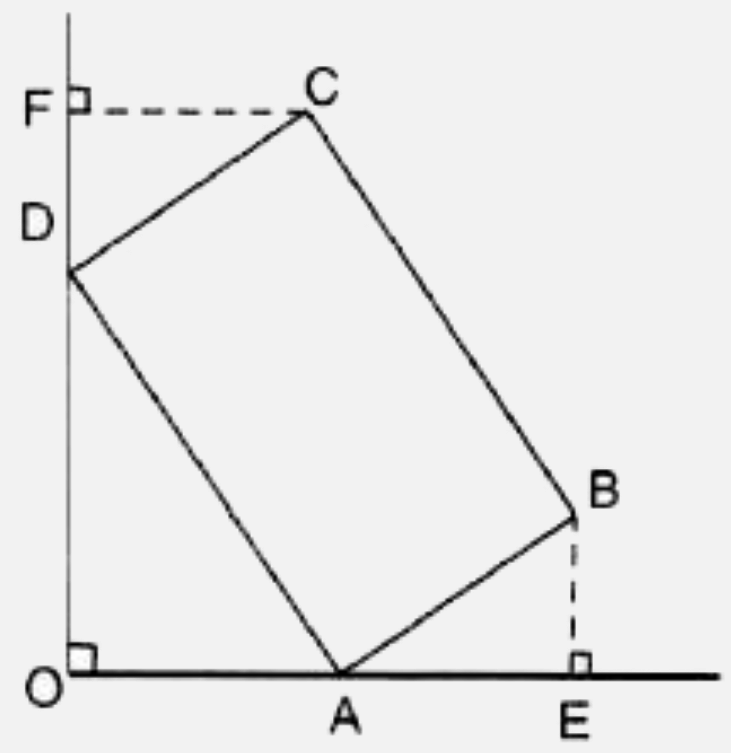
View SolutionIn the given figures , ABCD is a rectangle
Prove that : ΔABE≅ΔCDF.
View Solution
