A chord CD of a circles, whose centre is O, is bisected at P by a diameter AB.
Give 04 = OB = 15 cm and OP = 9 cm.

CD
The correct Answer is:24 cm
Step by step video & image solution for A chord CD of a circles, whose centre is O, is bisected at P by a diameter AB. Give 04 = OB = 15 cm and OP = 9 cm. CD by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A chord CD of a circles, whose centre is O, is bisected at P by a diameter AB.
Give 04 = OB = 15 cm and OP = 9 cm.

AD
View SolutionA chord CD of a circles, whose centre is O, is bisected at P by a diameter AB.
Give 0A = OB = 15 cm and OP = 9 cm.
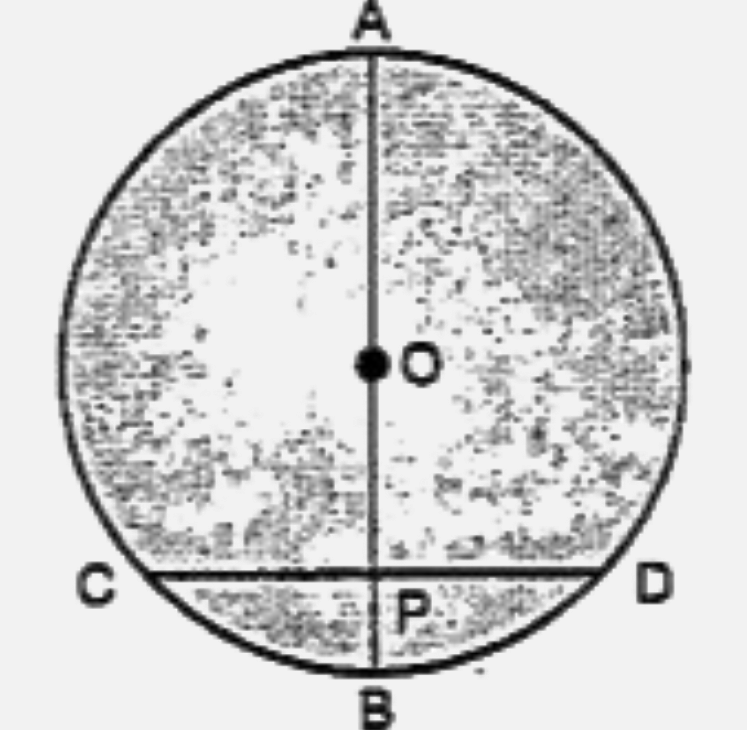
CB
View SolutionA chord CD of a circle, with centre o, is bisected by the diameter AB at point P. If OA = OB = 30 cm and OP = 18 cm, calcualte :
(i) CD (ii) AD
(##SEL_RKB_ICSE_MAT_IX_CR_03_E01_128_Q01.png" width="80%">
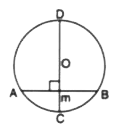
View SolutionIn the given figure, the diameter CD of a circle with centre 0 is perpendicular to the chord AB.
If AB = 8 cm and CM = 2 cm, find the radius of the circle.
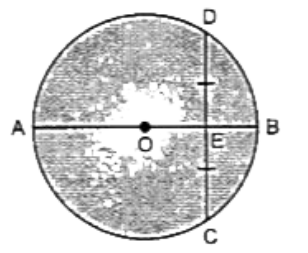
View SolutionThe figure, given below, show a circle with centre O in which diameter AB bisects the chord CD at point E. If CE = ED = 8 cm and EB = 4 cm. Find the radius of the circle.
View SolutionChords AB and CD of a circle with centre O, intersect at a point E. If OE bisects angle AED, prove that chord AB = chord CD.
View SolutionChords AB and CD of a circle when extended meet at point X. Given AB =4 cm, BX=6 cm and XD=6cm, calculate the length of CD.
View SolutionTwo equal chord AB and CD of a circle with centre O, intersect each other at point P inside the circle.

Prove that:
BP=DP
View SolutionAB and CD are two parallel chords of a circle on opposite sides of a diameter such that AB = 24 cm and CD = 10 cm. If the radius of the circle is 13 cm, find the distance between the two chords.
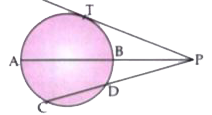
View SolutionIn the figure given below, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD =7.8 cm, PD = 5 cm, PB = 4 cm. Find
(i) AB.
(ii) The length of tangent PT.
View Solution
