Calculate the amount of work done to dissociate a system of three charges 1μC,1μCand−4μC placed on vertices of an equilateral triangle of side 10 cm.
To calculate the amount of work done to dissociate a system of three charges 1μC, 1μC, and −4μC placed at the vertices of an equilateral triangle with a side length of 10 cm, we will follow these steps:
Step 1: Identify the Charges and Configuration
We have three charges:
- QA=1μC=1×10−6C at vertex A
- QB=1μC=1×10−6C at vertex B
- QC=−4μC=−4×10−6C at vertex C
The distance between any two charges (the side of the triangle) is R=10 cm=0.1 m.
Step 2: Calculate the Potential Energy of the System
The potential energy U of the system of charges can be calculated using the formula for the potential energy between point charges:
U=k(QAQBR+QBQCR+QCQAR)
Where k=14πϵ0≈9×109N m2/C2.
Step 3: Substitute the Values into the Formula
1. Calculate the potential energy between QA and QB:
UAB=kQAQBR=9×109×(1×10−6)(1×10−6)0.1=9×109×1×10−120.1=9×10−3J
2. Calculate the potential energy between QB and QC:
UBC=kQBQCR=9×109×(1×10−6)(−4×10−6)0.1=9×109×−4×10−120.1=−36×10−3J
3. Calculate the potential energy between QC and QA:
UCA=kQCQAR=9×109×(−4×10−6)(1×10−6)0.1=9×109×−4×10−120.1=−36×10−3J
Step 4: Sum Up the Potential Energies
Now, we can find the total potential energy U:
U=UAB+UBC+UCA=9×10−3−36×10−3−36×10−3=9×10−3−72×10−3=−63×10−3J
Step 5: Calculate the Work Done to Dissociate the Charges
The work done W to dissociate the system is equal to the negative of the total potential energy:
W=−U=−(−63×10−3)=63×10−3J=0.063J
Final Answer
The amount of work done to dissociate the system of charges is 0.063J.
---
We have three charges:
- QA=1μC=1×10−6C at vertex A
- QB=1μC=1×10−6C at vertex B
- QC=−4μC=−4×10−6C at vertex C
The potential energy U of the system of charges can be calculated using the formula for the potential energy between point charges:
1. Calculate the potential energy between QA and QB:
UAB=kQAQBR=9×109×(1×10−6)(1×10−6)0.1=9×109×1×10−120.1=9×10−3J
UBC=kQBQCR=9×109×(1×10−6)(−4×10−6)0.1=9×109×−4×10−120.1=−36×10−3J
UCA=kQCQAR=9×109×(−4×10−6)(1×10−6)0.1=9×109×−4×10−120.1=−36×10−3J
Now, we can find the total potential energy U:
U=UAB+UBC+UCA=9×10−3−36×10−3−36×10−3=9×10−3−72×10−3=−63×10−3J
The work done W to dissociate the system is equal to the negative of the total potential energy:
W=−U=−(−63×10−3)=63×10−3J=0.063J
The amount of work done to dissociate the system of charges is 0.063J.
Similar Questions
Calculate the amount of work done to dissociate a system of 3 charges 1μC,1μC and 4μC placed on the vertices of an equilateral triangle of sides 10 cm.
View Solution(a) Derive an expression for the torque experienced by an electric dipole kept in a uniform electric field.
(b) Calculate the work done to dissociate the system of three charges placed on the vertices of a triangle as shown.
Here q=1.6×10−10C.

View SolutionHow much work has to be done in assembling three charged particles at the vertices of an equilateral triangle as shown in figure

View SolutionThree points charges of 1C,2Cand3C are placed at the corners of an equilateral triangle of side 100 cm. Find the work done to move these charges to the corners of a similar equilateral triangle of side 50 cm.
View SolutionThree points charges of 1C,2Cand3C are placed at the corners of an equilateral triangle of side 100 cm. Find the work done to move these charges to the corners of a similar equilateral triangle of side 50 cm.
View SolutionThree charges q1=1μC,q2=2μC and q3=3μC are placed on the vertices of an equilateral triangle of side 1.0 m. Find the net electric force acting on charge q1
View SolutionElectric charge of 1μC,−1μC and 2μC are placed in air at the corners A, B and C respectively of an equilateral triangle ABC having length of each side 10 cm. The resultant force on the charge at C is
View SolutionThree charges 2 μC , -4 μC and 8 μC are places at the three vertices of an equilateral triangle of side 10 cm. The potential at the centre of triangle is
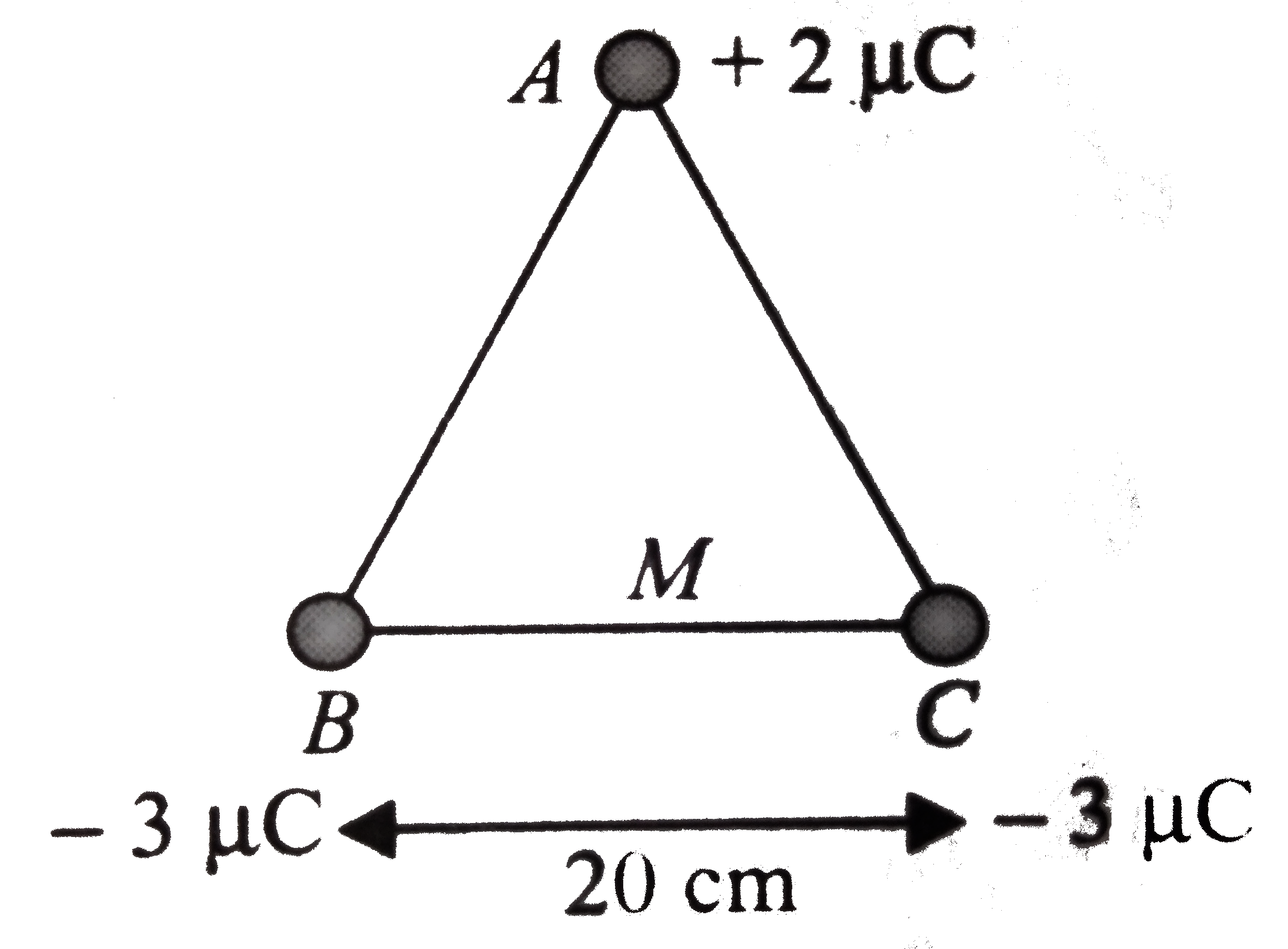
View SolutionThree point charges of +2μC,−3μC , and −3μC are kept at the vertices A, B, and C, respectively of an equilatreral triangle of side 20 cm. what should be the sign and magnitude of the charge (q) to be placed at the midpoint (M) of side BC so that the charge at A remains in equilibrium?
View SolutionCalculate electrostatic potential energy stored in a system consisting of two point charges 100μC and 40μC separated by a distance of 9cm, in vacuum.
View Solution
