Three points charges of 1C,2Cand3C are placed at the corners of an equilateral triangle of side 100 cm. Find the work done to move these charges to the corners of a similar equilateral triangle of side 50 cm.
To solve the problem of finding the work done to move the charges from one equilateral triangle to another, we will follow these steps:
Step 1: Understand the Configuration
We have three point charges:
- q1=1C
- q2=2C
- q3=3C
These charges are placed at the corners of an equilateral triangle with a side length of 100cm (or 1m). We need to find the work done to move these charges to a new equilateral triangle with a side length of 50cm (or 0.5m).
Step 2: Calculate the Initial Potential Energy
The potential energy U of a system of point charges is given by the formula:
U=k∑i<jqiqjrij
where k is Coulomb's constant (k≈8.99×109Nm2/C2), qi and qj are the charges, and rij is the distance between the charges.
For the initial triangle (side = 1m):
- The potential energy between q1 and q2:
U12=kq1q21=8.99×1091×21=17.98×109J
- The potential energy between q2 and q3:
U23=kq2q31=8.99×1092×31=53.94×109J
- The potential energy between q3 and q1:
U31=kq3q11=8.99×1093×11=26.97×109J
Total initial potential energy Ui:
Ui=U12+U23+U31=17.98×109+53.94×109+26.97×109=98.89×109J
Step 3: Calculate the Final Potential Energy
For the final triangle (side = 0.5m):
- The potential energy between q1 and q2:
U′12=kq1q20.5=8.99×1091×20.5=35.96×109J
- The potential energy between q2 and q3:
U′23=kq2q30.5=8.99×1092×30.5=107.88×109J
- The potential energy between q3 and q1:
U′31=kq3q10.5=8.99×1093×10.5=53.94×109J
Total final potential energy Uf:
Uf=U′12+U′23+U′31=35.96×109+107.88×109+53.94×109=197.78×109J
Step 4: Calculate the Work Done
The work done W in moving the charges is equal to the change in potential energy:
W=Uf−Ui=197.78×109−98.89×109=98.89×109J
Final Answer
The work done to move the charges to the corners of the smaller triangle is:
W=98.89×109J
We have three point charges:
- q1=1C
- q2=2C
- q3=3C
The potential energy U of a system of point charges is given by the formula:
U=k∑i<jqiqjrij
where k is Coulomb's constant (k≈8.99×109Nm2/C2), qi and qj are the charges, and rij is the distance between the charges.
- The potential energy between q1 and q2:
U12=kq1q21=8.99×1091×21=17.98×109J
- The potential energy between q2 and q3:
U23=kq2q31=8.99×1092×31=53.94×109J
- The potential energy between q3 and q1:
U31=kq3q11=8.99×1093×11=26.97×109J
Ui=U12+U23+U31=17.98×109+53.94×109+26.97×109=98.89×109J
For the final triangle (side = 0.5m):
- The potential energy between q1 and q2:
U′12=kq1q20.5=8.99×1091×20.5=35.96×109J
- The potential energy between q2 and q3:
U′23=kq2q30.5=8.99×1092×30.5=107.88×109J
- The potential energy between q3 and q1:
U′31=kq3q10.5=8.99×1093×10.5=53.94×109J
Uf=U′12+U′23+U′31=35.96×109+107.88×109+53.94×109=197.78×109J
The work done W in moving the charges is equal to the change in potential energy:
W=Uf−Ui=197.78×109−98.89×109=98.89×109J
The work done to move the charges to the corners of the smaller triangle is:
W=98.89×109J
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.1
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.2
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
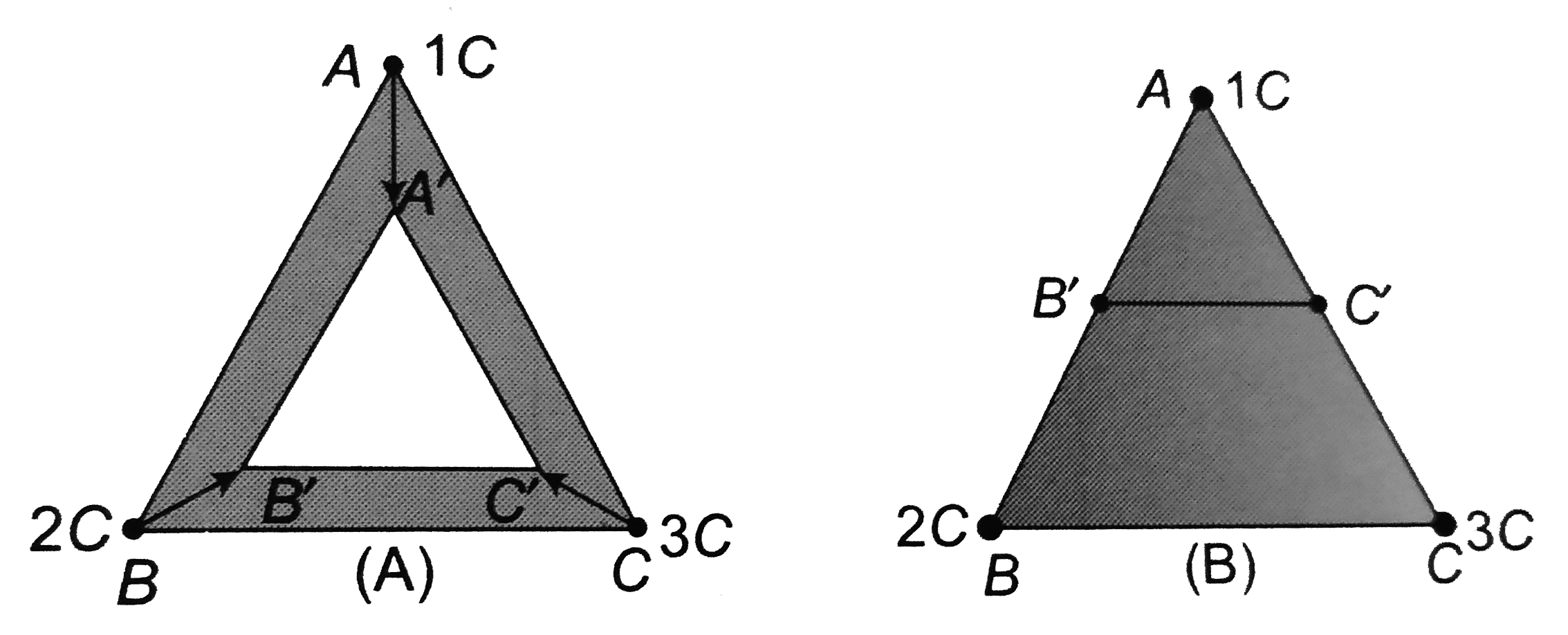
Three point charges 1C,2C and 3C are placed at the corners of an equilaternal triangle of side 1m. The work required to move these charges to the corners of a smaller equilaternal triangle of side 0.5m in two differenct ways as in fig. (A) and fig. (B) are Wa and Wb then:
View SolutionFind the perimeter of an equilateral triangle of side 13 cm.
View SolutionFind the altitude of an equilateral triangle of side 8 cm.
View SolutionFind the area of an equilateral triangle whose side is a cm.
View SolutionFind the perimeter of an equilateral triangle with side 9cm.
View SolutionConstruct an equilateral triangle of sides 6 cm each.
View SolutionConstruct an equilateral triangle whose side is 5 cm.
View SolutionFind the area of an equilateral triangle having each side 4 cm.
View SolutionThe area of an equilateral triangle with side 2√3 cm is
View SolutionFind the area of an equilateral triangle, whose one side is a cm.
View Solution
