In a triangle ABC, right angled at A. The radius of the inscribed circle is 2cm. Radius of the circle touching the side BC and also sides AB and AC produced is 15cm. The length of the side BC measured in cm is a, then 9a/13 is........Solution in Bengali
[9]
Topper's Solved these Questions
Similar Questions
If A={x:x=4n+1,n≤5 and n∈N} and B={3n:n≤8andn∈N} then (A-B) is
View SolutionFor a frequency distribution, mean deviation about mean is computed by
View SolutionThe sides AB,BC and AC of a triangle ABC touch a circle with centre O and radius r at P Q,and R respectively.
View SolutionDelta ABC is a right angled triangle with /_A=90^(0)AB=bcm,AC=acm, and BC=ccmA circle is inscribed in this triangle.The radius of the circle,in cm, is:
View SolutionIf a circle is inscribed in right angled triangle ABC with right angle at B, show that the diameter of the circle is equal to AB+BC−AC.
View SolutionABC किसी वृत्त में अंकित समद्विबाहु त्रिकोण है यदि AB=AC=12√5 तथा BC=24 सेमी है तो वृत्त की त्रिज्या क्या होगी ?
View SolutionA circle with centre P is inscribed in the ΔABC Side AB, side BC and side A C touch the circle at points L,M and N respectively . Prove that :
A(ΔABC)=12(AB+BC+AC)×r
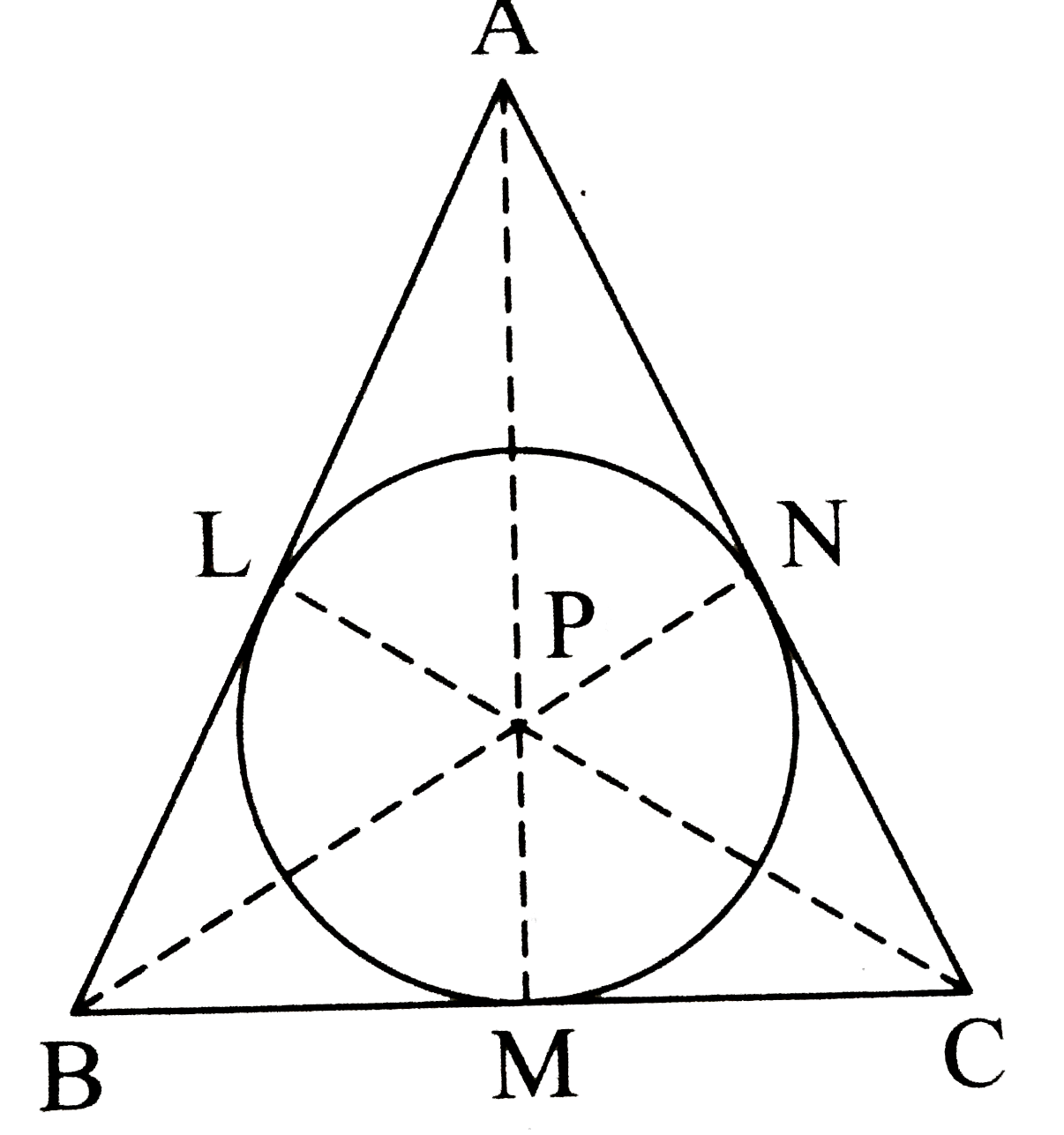
View SolutionA circle with centre P is inscribed in the △ ABC. Side AB, side BC and side AC touch the circle at points L,M and N respectively. Radius of the circle is r. Prove that: A(△ABC)=12(AB+BC+AC)×r.
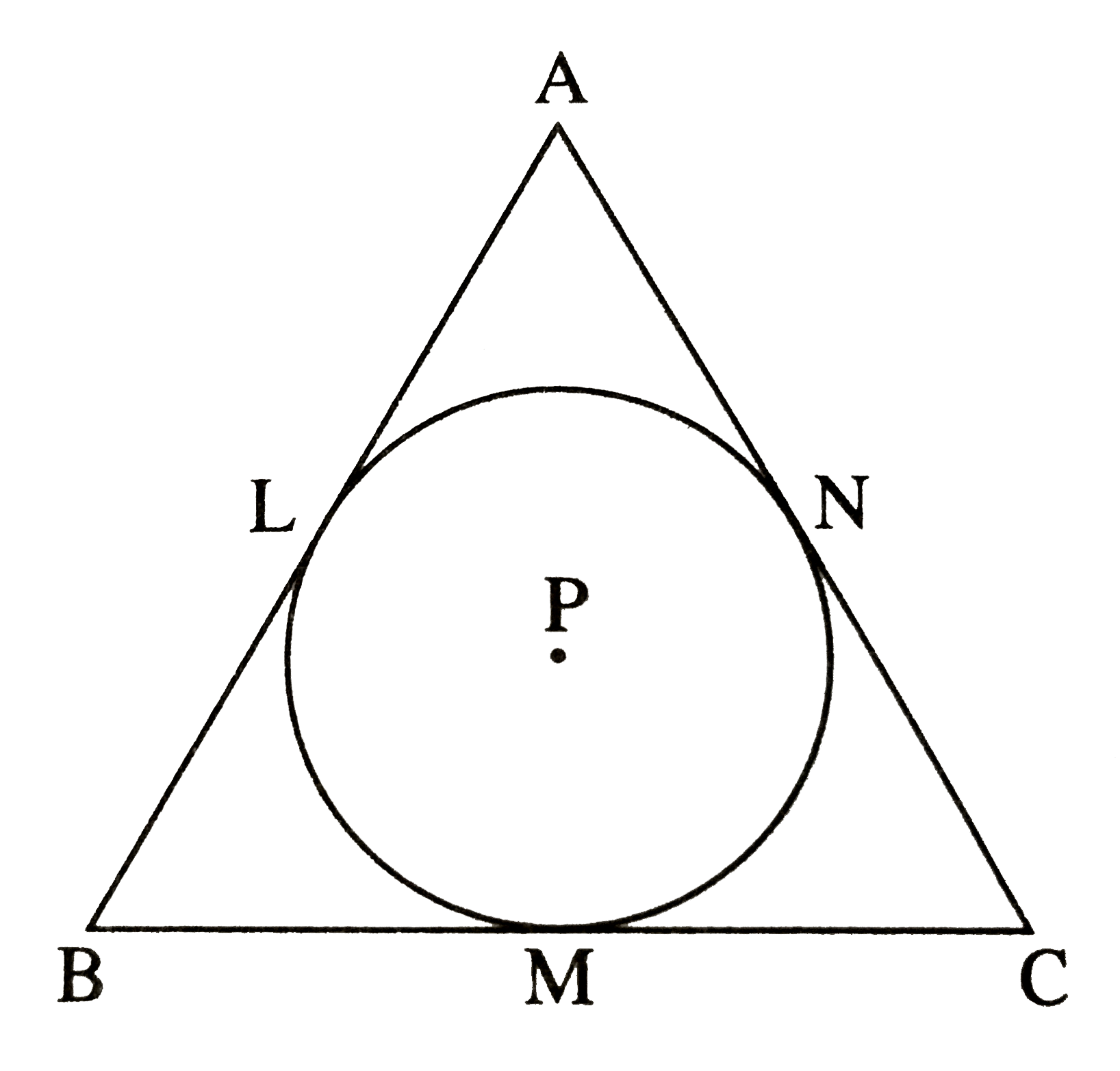
View SolutionThe radius of the circle touching two sides AB and AC of a triangle ABC and having its center on BC is equal to………………..
View SolutionIn a right triangle ABC, right angled at B , BC = 15 cm and AB = 8 cm . A circle is inscribed in the traiangle ABC . The radius of the circle is ……
View SolutionIn ΔABC, right-angled at B, AB = 3 cm and ∠BAC =60∘ . Determine the lengths of the sides BC and AC.
View SolutionABC is an isosceles triangle inscribed in a circle. If AB=AC12√5 and BC = 24 cm then radius of circle is:
View SolutionFind the ex-radius of a ex-circle which touches side BC.
AB=13cm,BC=14cm,CA=15cm.
View SolutionThe radius of the circle touching two sides AB and AC of a triangle ABC and having its center on BC is equal to………………..
View SolutionIf a circle is inscribed in right angled triangle ABC with right angle at B, show that the diameter of the circle is equal to AB+BC−AC.
View Solution
