For a given velocity of projection from a point on the inclined plane, the maximum range down the plane is three times the maximum range up the incline. Then find the angle of inclination of the inclined plane.
To solve the problem, we will use the formulas for the maximum range of projectile motion on an inclined plane. Let's break down the solution step-by-step.
Step 1: Understand the problem
We need to find the angle of inclination (θ) of the inclined plane given that the maximum range down the plane (R2) is three times the maximum range up the incline (R1).
Step 2: Write the formulas for maximum range
The maximum range on an inclined plane can be expressed as:
- For upward projection (R1):
R1=u2g⋅1+sinθ1−sinθ
- For downward projection (R2):
R2=u2g⋅1−sinθ1+sinθ
Step 3: Set up the equation based on the given condition
According to the problem, the maximum range down the plane is three times the maximum range up the incline:
R2=3R1
Substituting the expressions for R1 and R2, we get:
u2g⋅1−sinθ1+sinθ=3(u2g⋅1+sinθ1−sinθ)
Step 4: Simplify the equation
Since u2g is common on both sides, we can cancel it out:
1−sinθ1+sinθ=3⋅1+sinθ1−sinθ
Step 5: Cross-multiply to eliminate the fractions
Cross-multiplying gives us:
(1−sinθ)(1−sinθ)=3(1+sinθ)(1+sinθ)
Expanding both sides:
1−2sinθ+sin2θ=3(1+2sinθ+sin2θ)
1−2sinθ+sin2θ=3+6sinθ+3sin2θ
Step 6: Rearrange the equation
Rearranging gives:
1−3−2sinθ−6sinθ+sin2θ−3sin2θ=0
−2−8sinθ−2sin2θ=0
Dividing through by -2:
sin2θ+4sinθ+1=0
Step 7: Solve the quadratic equation
Using the quadratic formula sinθ=−b±√b2−4ac2a:
Here, a=1,b=4,c=1:
sinθ=−4±√16−42=−4±√122=−4±2√32=−2±√3
Since sinθ must be positive, we take:
sinθ=−2+√3
Step 8: Find the angle θ
Calculating the value gives:
sinθ=12⇒θ=30∘
Final Answer
The angle of inclination of the inclined plane is:
θ=30∘
We need to find the angle of inclination (θ) of the inclined plane given that the maximum range down the plane (R2) is three times the maximum range up the incline (R1).
The maximum range on an inclined plane can be expressed as:
- For upward projection (R1):
R1=u2g⋅1+sinθ1−sinθ
- For downward projection (R2):
R2=u2g⋅1−sinθ1+sinθ
According to the problem, the maximum range down the plane is three times the maximum range up the incline:
R2=3R1
Substituting the expressions for R1 and R2, we get:
u2g⋅1−sinθ1+sinθ=3(u2g⋅1+sinθ1−sinθ)
Since u2g is common on both sides, we can cancel it out:
1−sinθ1+sinθ=3⋅1+sinθ1−sinθ
Cross-multiplying gives us:
(1−sinθ)(1−sinθ)=3(1+sinθ)(1+sinθ)
Expanding both sides:
1−2sinθ+sin2θ=3(1+2sinθ+sin2θ)
1−2sinθ+sin2θ=3+6sinθ+3sin2θ
Rearranging gives:
1−3−2sinθ−6sinθ+sin2θ−3sin2θ=0
−2−8sinθ−2sin2θ=0
Dividing through by -2:
sin2θ+4sinθ+1=0
Using the quadratic formula sinθ=−b±√b2−4ac2a:
Here, a=1,b=4,c=1:
sinθ=−4±√16−42=−4±√122=−4±2√32=−2±√3
Since sinθ must be positive, we take:
sinθ=−2+√3
Calculating the value gives:
sinθ=12⇒θ=30∘
The angle of inclination of the inclined plane is:
θ=30∘
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.3
12 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.4
11 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.1
15 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
A body has maximum range R1 when projected up the plane. The same body when projected down the inclined plane, it has maximum range R2. Find the maximum horizontal range. Assume equal speed of projection in each case and the body is projected onto the inclined plane in the line of the greatest slope.
View SolutionA body takes ''n'' times as much time to slide down a rough inclined plane as it takes to slide down an identical but smooth inclined plane. If the angle of inclination of the inclined plane is ''θ''. What is the coefficient of friction between the body and the rough plane ?
View SolutionA particle is projected on an inclined plane with a speed u as shown in (Fig. 5.61). Find the range of the particle on the inclined plane.
 .
.
View SolutionThe force required to move a body up a rough inclined plane is double the force required to prevent the body from sliding down the plane. The coefficient of friction when the angle of inclination of the plane is 60∘ is .
View SolutionThe velocity of a sphere rolling down an inclined plane of height h at an inclination θ with the horizontal, will be :
View SolutionA Sphere pure rolls on a rough inclined plane with initial velocity 2.8 m/s. Find the maximum distance on the inclined plane
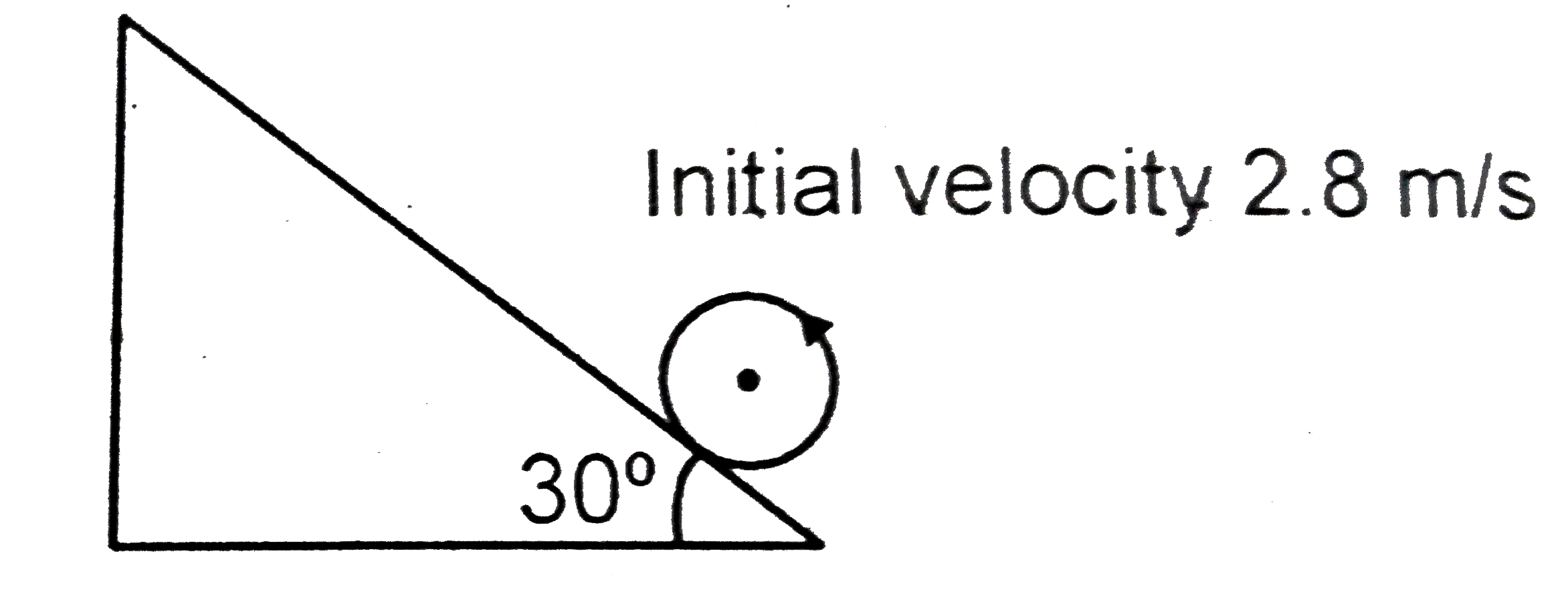
View SolutionA ball starts falling freely from a height h from a point on the inclined plane forming an angle α with the horizontal as shown. After collision with the incline it rebounds elastically off the plane. Then it again strikes the incline at
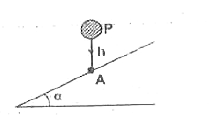
View SolutionA ball is projected from point A with velocity 20 m/s prependicular to the inclined plane as shown in figure. What is the range of the ball on the inclined plane ?

View SolutionA body is sliding down an inclined plane having coefficient of friction 1/3. If the normal reaction is three times that of the resultant downward force along the incline, the angle between the inclined plane and the horizontal is
View SolutionA block kept on a rough inclined plane, as shown in the figure, remains at rest upto a maximum force 2N down the inclined plane. The maximum external force up the inclined plane that does not move the block is 10N. The coefficient of static friction between the block and the plane is : : [Taking g=10m/s2].

View Solution
