Two men of masses M and M+m start simultaneously from the ground and climb with uniform accelerations up from the free ends of a massless inextensible rope whick passes over a smooth pulley at a height h from the ground.
(a) Which man reaches the pulley first?
(b) If the mam who reaches first takes time t to reach the pulley, then find the distance of the second man from the pulley at this instant.
The correct Answer is:ligher man; m(M+m)[gt22+h]
To solve the problem step by step, we will break it down into parts (a) and (b) as specified in the question.
Part (a): Which man reaches the pulley first?
1. Identify the forces acting on each man:
- For the man with mass M:
- Weight: W1=Mg
- Tension in the rope: T
- For the man with mass M+m:
- Weight: W2=(M+m)g
- Tension in the rope: T
2. Write the equations of motion:
- For the first man (mass M):
T−Mg=Ma1(1)
- For the second man (mass M+m):
T−(M+m)g=(M+m)a2(2)
3. Rearranging the equations to find accelerations:
- From equation (1):
a1=TM−g(3)
- From equation (2):
a2=TM+m−g(4)
4. Compare the accelerations:
- To determine which man reaches the pulley first, we need to compare a1 and a2:
a1>a2ifTM>TM+m
- Since M<M+m, it follows that:
1M>1M+m⟹a1>a2
5. Conclusion for part (a):
- The man with mass M reaches the pulley first.
Part (b): Distance of the second man from the pulley when the first man reaches the pulley
1. Time taken by the first man to reach the pulley:
- Let the time taken by the first man to reach the pulley be t. The distance traveled by the first man is:
h=12a1t2(5)
2. Substituting a1 from equation (3):
- From equation (3):
h=12(TM−g)t2
3. Distance traveled by the second man:
- The distance traveled by the second man in time t is:
s2=12a2t2(6)
4. Substituting a2 from equation (4):
- From equation (4):
s2=12(TM+m−g)t2
5. Distance from the pulley:
- The distance of the second man from the pulley when the first man reaches it is:
d=h−s2(7)
6. Substituting h and s2:
- From equations (5) and (6):
d=(12(TM−g)t2)−(12(TM+m−g)t2)
7. Simplifying the expression:
- Factor out 12t2:
d=12t2((TM−g)−(TM+m−g))
- This simplifies to:
d=12t2(TM−TM+m)
8. Final expression for distance:
- The distance of the second man from the pulley is:
d=12t2(T(M+m)−TMM(M+m))=12t2(TmM(M+m))
- For the man with mass M:
- Weight: W1=Mg
- Tension in the rope: T
- For the man with mass M+m:
- Weight: W2=(M+m)g
- Tension in the rope: T
- For the first man (mass M):
T−Mg=Ma1(1)
- For the second man (mass M+m):
T−(M+m)g=(M+m)a2(2)
- From equation (1):
a1=TM−g(3)
- From equation (2):
a2=TM+m−g(4)
- To determine which man reaches the pulley first, we need to compare a1 and a2:
a1>a2ifTM>TM+m
- Since M<M+m, it follows that:
1M>1M+m⟹a1>a2
- The man with mass M reaches the pulley first.
- Let the time taken by the first man to reach the pulley be t. The distance traveled by the first man is:
h=12a1t2(5)
- From equation (3):
h=12(TM−g)t2
- The distance traveled by the second man in time t is:
s2=12a2t2(6)
- From equation (4):
s2=12(TM+m−g)t2
- The distance of the second man from the pulley when the first man reaches it is:
d=h−s2(7)
- From equations (5) and (6):
d=(12(TM−g)t2)−(12(TM+m−g)t2)
- Factor out 12t2:
d=12t2((TM−g)−(TM+m−g))
- This simplifies to:
d=12t2(TM−TM+m)
- The distance of the second man from the pulley is:
d=12t2(T(M+m)−TMM(M+m))=12t2(TmM(M+m))
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Single Correct75videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Multiple Correct11videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.413videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE10videos
MISCELLANEOUS VOLUME 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type1videos
NEWTON'S LAWS OF MOTION 2
Similar Questions
A balloon starts from rest from the ground and moves with uniform acceleration g/8. When it reaches a height h a ball is dropped from it. Find the time taken by the ball to reach the ground.
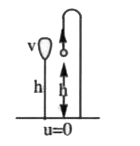 View Solution
View SolutionIn the given figure all the surfaces are smooth. Find the time taken by the block to reach from the free end to the pulley attached to the plank.
Distance between free end and pulley is l.
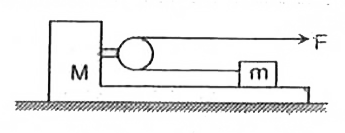 View Solution
View SolutionIn the arrangement shown, the mass m will ascend with an acceleration ( Pulley and rope are massless)
 View Solution
View SolutionIn the arrangement shown, the mass m will ascend with an acceleration ( Pulley and rope are massless)
 View Solution
View SolutionA particle is projected vertically upwards from a point A on the ground. It takes t1 time to reach a point B but it still continues to move up. If it takes further t2 time to reach the ground from point B then height of point B from the ground is :-
View SolutionA stone is thrown vertically from the ground. It reaches the maximum height of 500 m in 10 sec. After what time it will reach the ground from the maximum height reached?
View SolutionA body is falling from height 'h' it takes t1 time to reach the ground. The time taken to cover the first half of height is:-
View SolutionA man of mass m stands on a frame of mass M. He pulls on a light rope, which passes over a pulley. The other end of the rope is attached to the frame. For the system to be in equilibrium, what force must the man exert on the rope?
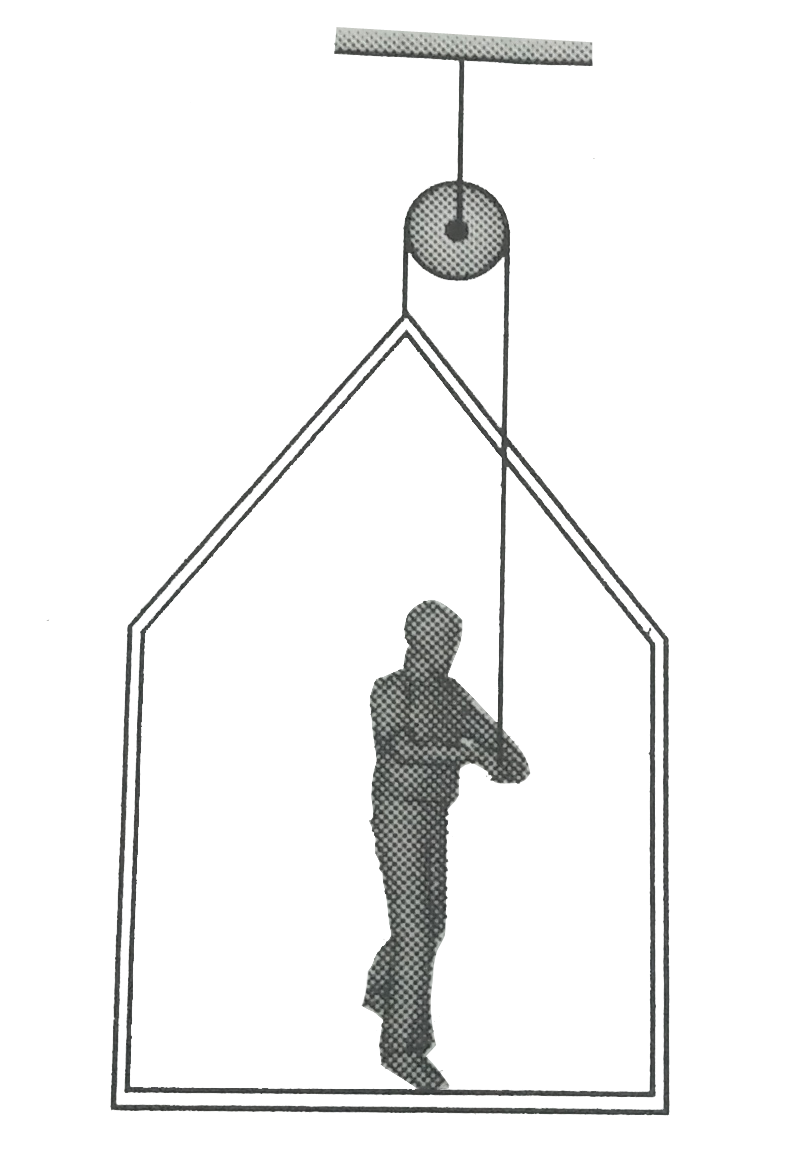 View Solution
View SolutionA stone is dropped from a height h. simultaneously, another stone is thrown up from the ground which reaches a height 4h. The two stones will cross each other after time:-
View SolutionA mass m rests on a horizontal table. It is attached to a light inextensible string which passes over a smooth pulley and carries a mass m at the other end. If the mass m is raised vertically through a distance h and is then dropped, what is the speed with which the mass 2m begins to rise?
 View Solution
View Solution

