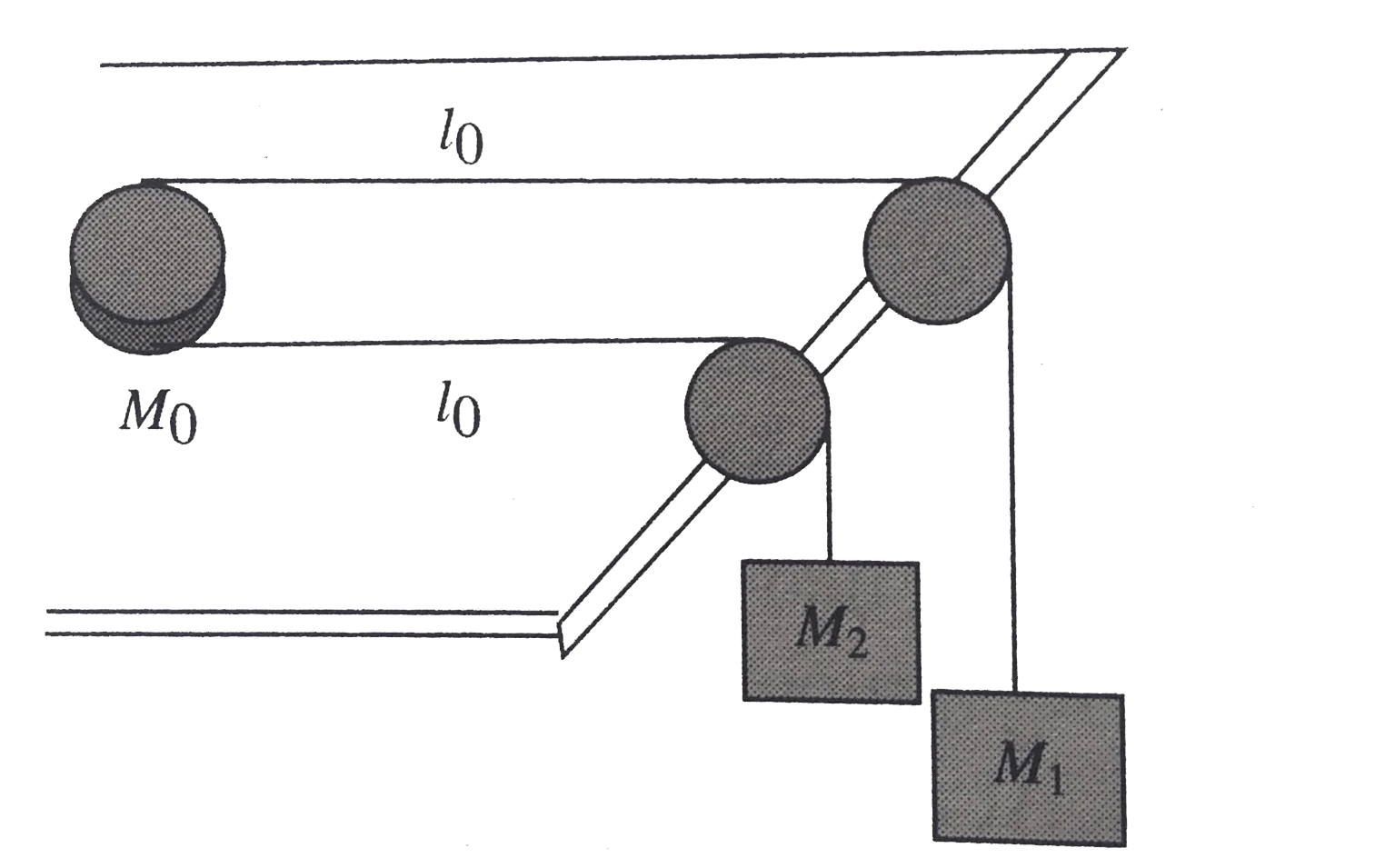
A smooth pulley A of mass M0 is lying on a frictionless table. A massless rope passes round the pulley and has masses M1 and M2 tied to its ends, the two portions of the string being perpendicular to the edge of the table so that the masses hang vertically. Find the acceleration of the pulley.
The correct Answer is:4M1M2g4M1M2+M0(M1+M2)
If a0a1 and a2 are accelerations of M0M1, and M2, respectively, then
a0=a1−a22⇒2a0=a1−a2 ..(i)
For motion of M0,2T=M0a0 ..(ii)
For motion of mass M1,M1g−T=M1a1 ...(iii)
For motion of mass M2,T−M2g=M2a2 ..(iv)
Substituting value of a0,a1 and a2 From Eqs. (ii), (iii) and (iv) in (i) we get,
2(2TM0)=(g−TM1)=(TM2−g)
4TM0=2g−T(1M1+1M2)
i.e. (4M0+1M1+1M2)T=2g
This gives T=2M0M1M2g4M1M2+M0(M1+M2) ...(v)
Acceleration of the pulley of A from Eq. (ii) is
a0=2TM0=4M1M2g4M1M2+M0(M1+M2).
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Single Correct75videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Multiple Correct11videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.413videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE10videos
MISCELLANEOUS VOLUME 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type1videos
NEWTON'S LAWS OF MOTION 2
Similar Questions
Two bodies of masses 4 kg and 6 kg are tied to the ends of a massless string. The string passes over a frictionless pulley. The acceleration of the system is :
View SolutionA block of mass 20 kg is set into motion on a frictionless horizontal surface with the help of massless smooth pulley and rope as shown in the figure. Find the acceleration of the pulley
_PHY_E05_192_Q05.png) View Solution
View SolutionA pulley fixed to the ceiling carried a thread with bodies of masses m1 and m2 attached to its ends. The mases of the pulley and the thread are negligible and friction is absent. Find the acceleration of the centre of mass of this system.
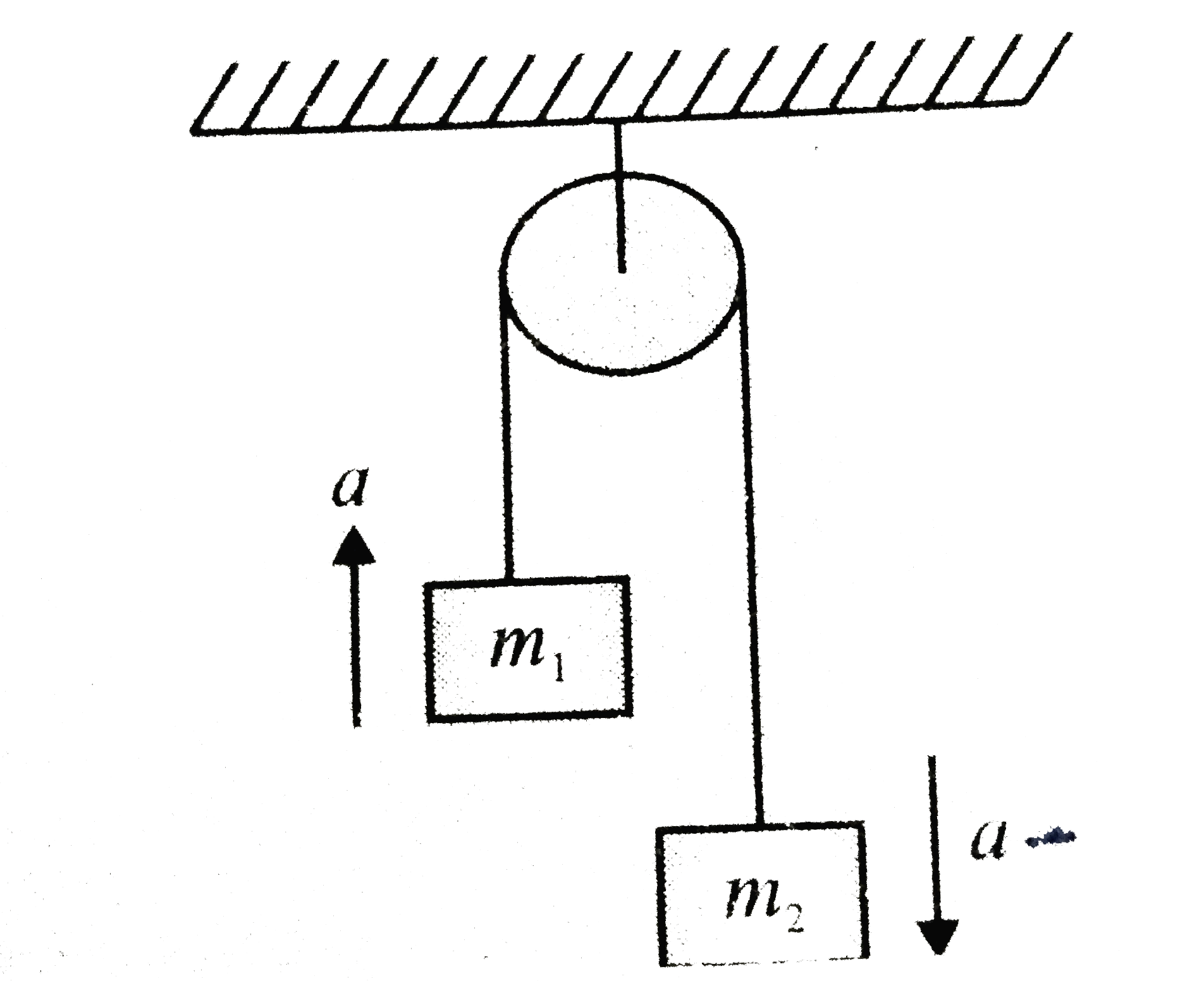 View Solution
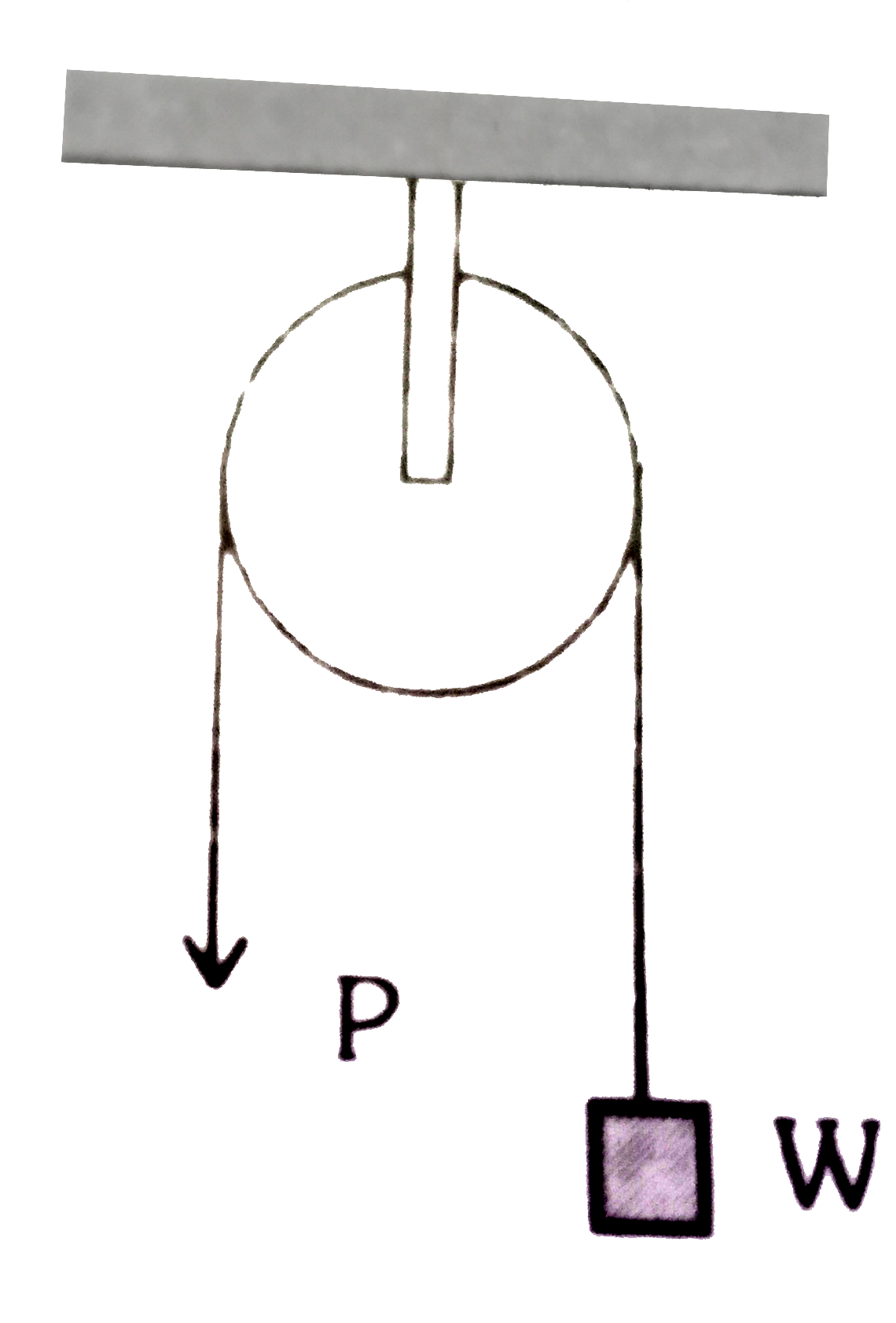
View SolutionWhat is the mechanical advantage of single fixed pulley:-
 View Solution
View SolutionIn the arrangement shown in fig. pulleys are smooth and massless. Threads are massless and inextensible. Find acceleration of mass m1.
 View Solution
View SolutionA block of mass of 20kg is set into motion on a frictionless horizontal surface with the help of massless smooth pulley and rope as shown in figure.Find the acceleration of the pulley
_PHY_E05_034_Q01.png) .jpgView Solution
.jpgView SolutionTwo mass m1andm2 are attached to a flexible inextensible massless rope, which passes over a frictionless and massless pully. Find the accelerations of the masses and tension in the rope.
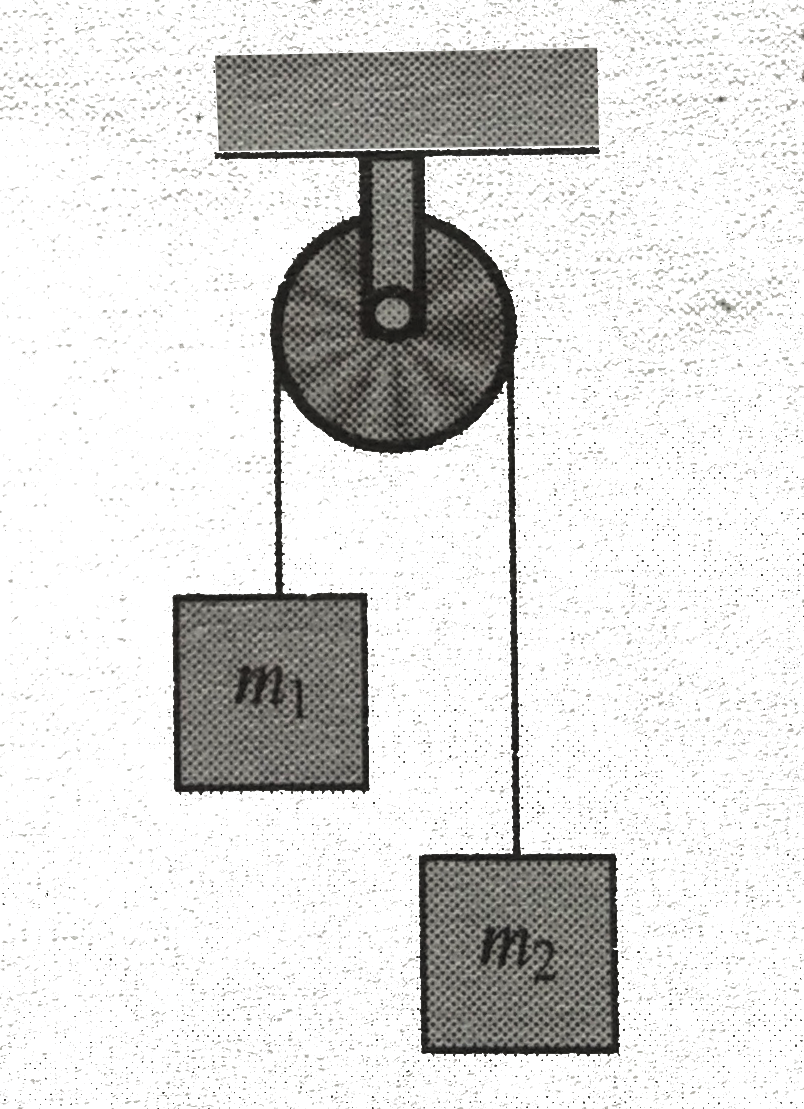 View Solution
View SolutionIn the arrangement shown, the mass m will ascend with an acceleration ( Pulley and rope are massless)
 View Solution
View SolutionIn the arrangement shown, the mass m will ascend with an acceleration ( Pulley and rope are massless)
 View Solution
View SolutionA block of mass m1 rests on a horizontal table. A string tied to the block is passed on a frictionless pulley fixed at the end of the table and to the other end of string is hung another block of mass m2. The acceleration of the system is
View Solution

