A car moving along a circular track of radius 50.0m acceleration from rest at 3.00ms2 Consider a situation when the car's centripetal acceleration equal its tangential acceleration
The angle around the track does the car travel is 1 red
The magnitude of the car's total acceleration at that is 3√2ms−1
Time elapses before this situation is √503s
The distance travelled by the car during this time 25m
The correct Answer is:b,c,d
To solve the problem, we need to analyze the situation where a car is moving along a circular track with a radius of 50.0 m and is accelerating from rest at a rate of 3.00 m/s². We are particularly interested in the condition where the car's centripetal acceleration is equal to its tangential acceleration.
1. Understanding the Accelerations:
- The tangential acceleration at is given as 3.00m/s2.
- The centripetal acceleration ac is given by the formula:
ac=v2r
where v is the tangential velocity and r is the radius of the circular path.
2. Setting Up the Condition:
- We need to find the condition when ac=at.
- Thus, we set the two accelerations equal:
v2r=at
- Substituting the known values:
v250=3
3. Solving for Velocity v:
- Rearranging the equation gives:
v2=3×50
v2=150
v=√150≈12.25m/s
4. Finding Time Elapsed:
- The car starts from rest, so we can use the equation of motion to find the time t taken to reach this velocity:
v=u+att
- Since the initial velocity u=0:
12.25=0+3t
t=12.253≈4.08s
5. Finding the Distance Travelled:
- The distance s travelled during this time can be calculated using the equation:
s=ut+12att2
- Again, since u=0:
s=12×3×(4.08)2
s≈12×3×16.64≈24.96m≈25m
6. Finding the Angle Travelled:
- The angle θ in radians that the car travels can be found using the relationship:
θ=sr
- Substituting the values:
θ=2550=0.5radians
Final Results:
- The distance travelled by the car is approximately 25 m.
- The angle travelled by the car is approximately 0.5 radians.
- The tangential acceleration at is given as 3.00m/s2.
- The centripetal acceleration ac is given by the formula:
ac=v2r
where v is the tangential velocity and r is the radius of the circular path.
- We need to find the condition when ac=at.
- Thus, we set the two accelerations equal:
v2r=at
- Substituting the known values:
v250=3
- Rearranging the equation gives:
v2=3×50
v2=150
v=√150≈12.25m/s
- The car starts from rest, so we can use the equation of motion to find the time t taken to reach this velocity:
v=u+att
- Since the initial velocity u=0:
12.25=0+3t
t=12.253≈4.08s
- The distance s travelled during this time can be calculated using the equation:
s=ut+12att2
- Again, since u=0:
s=12×3×(4.08)2
s≈12×3×16.64≈24.96m≈25m
- The angle θ in radians that the car travels can be found using the relationship:
θ=sr
- Substituting the values:
θ=2550=0.5radians
- The distance travelled by the car is approximately 25 m.
- The angle travelled by the car is approximately 0.5 radians.
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Multiple Correct
2 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Linked Comprehension
52 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Subjective
21 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A point starts from rest and moves along a circular path with a constant tangential acceleration. After one rotation, the ratio of its radial acceleration to its tangential acceleration will be equal to
View SolutionA small toy car moves round a circular track of radius 4 m. The car makes one revolution in 10 s. Calculate (a) the speed of the car, (b) its centripetal acceleration, (c) the centripetal force exerted,by the track if the.mass of the car is 200 g, (d) the safe speed with which the car can move around without toppling, if the distance between the wheels is 4 cm and the height of the centre of gravity of the car from the horizontal is 2 cm.
View SolutionA particle is moving on a circular track of radius 30 cm with a constant speed of 6ms−1. Its acceleration is
View SolutionA particle moves in a circle with constant tangential acceleration starting from rest. At t = 1/2 s radial acceleration has a value 25% of tangential acceleration. The value of tangential acceleration will be correctly represented by
View SolutionAssertion:- A particle is moving in a circle with constant tangential acceleration such that its speed v is increasing. Angle made by resultant acceleration of the particle with tangential acceleration increases with time.
Reason:- Tangential acceleration =∣∣∣d→vdt∣∣∣ and centripetal acceleration =v2R
View SolutionA particle is moving with a constant angular acceleration of 4rad/s2 in a circular path. At time t=0 , particle was at rest. Find the time at which the magnitudes of centripetal acceleration and tangential acceleration are equal.
View SolutionFigur shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5m at a given instant of time. At this instant, find:
(a) the radius acceleration,
(b) the speed of the acceleration,
(c) its tangential acceleration.
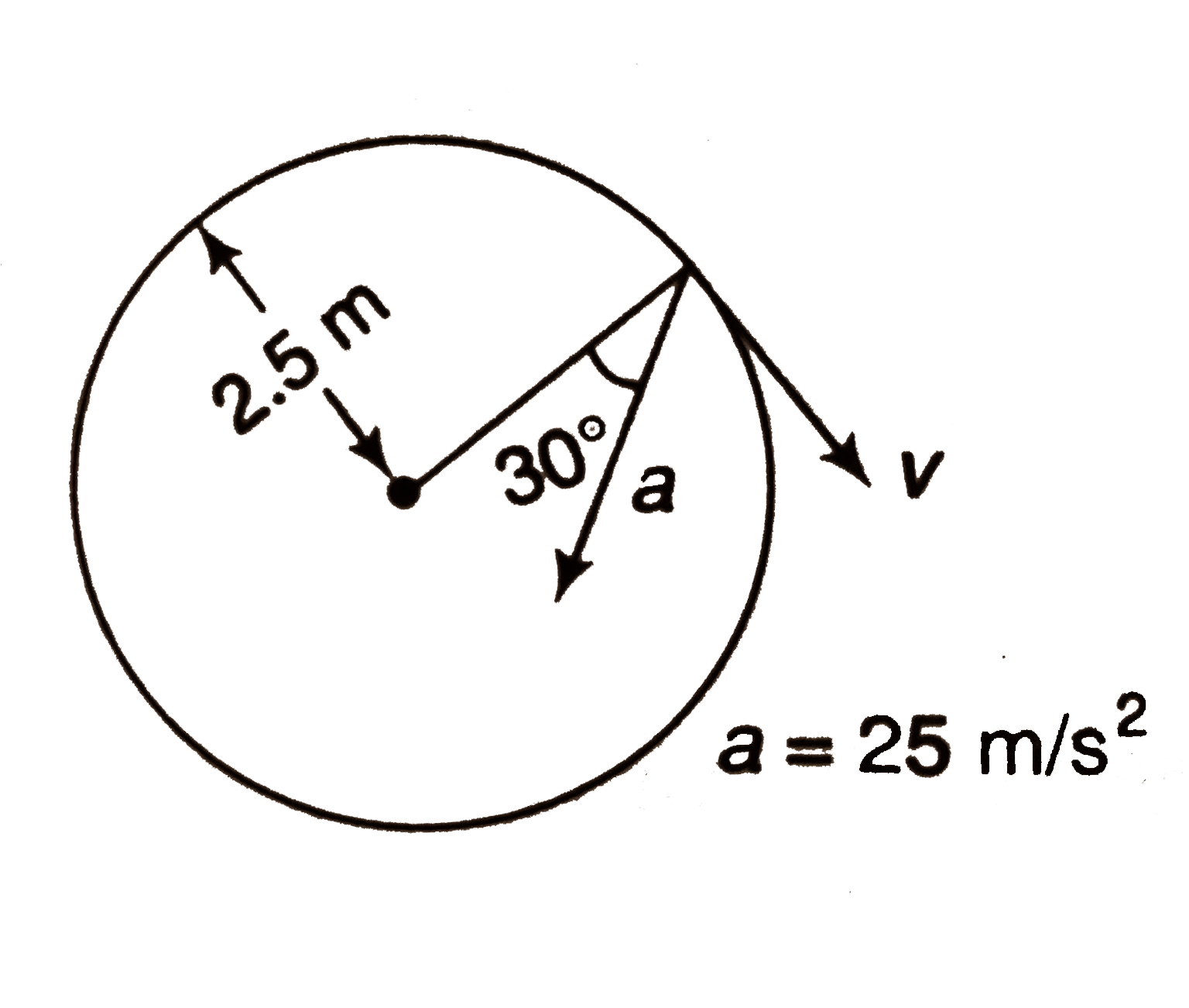
View SolutionA point on the periphery of a rotating disc has its acceleration vector making angle of 30∘ with the velocity . The ratio (ac/at(ac is centripetal acceleration and at is tangential acceleration) equals
View SolutionA racing car travelling at 35ms−1 along a circular path of radius 700 m, starts picking up speed at the rate of 2ms−2 What is its total acceleration ?
View SolutionA car is moving at a speed of 40 m/s on a circular track of radius 400 m. this speed is increasing at the rate of 3 m/s2. The acceleration of car is
View Solution
