A cube of iron of edge 5cm floats on the surface of mercury, contained in a tank. Water is then poured on top, so that the cube just gets immersed. Find the depth of water layer. (Specific gravities of iron-and mercury are 7.8 and 13.6, respectively.)
The correct Answer is:2cm
To solve the problem of finding the depth of the water layer above the iron cube that is floating on mercury, we will follow these steps:
1. Understand the Problem:
We have a cube of iron with an edge of 5 cm floating on mercury. When water is poured on top, the cube becomes fully immersed. We need to find the depth of the water layer (denoted as x).
2. Calculate the Volume of the Iron Cube:
The volume V of the cube can be calculated using the formula for the volume of a cube:
V=edge3=(5cm)3=125cm3
3. Calculate the Weight of the Iron Cube:
The weight W of the iron cube can be calculated using the formula:
W=Volume×Density×g
The density of iron can be found using its specific gravity:
Density of iron=Specific gravity×Density of water=7.8×1g/cm3=7.8g/cm3
Therefore, the weight of the cube is:
W=125cm3×7.8g/cm3×g=975g×g
4. Set Up the Buoyant Force Equation:
The buoyant force Fb acting on the cube is equal to the weight of the fluid displaced by the cube. The total buoyant force is the sum of the buoyant forces from both the water and the mercury:
Fb=Fwater+Fmercury
Where:
- Fwater=ρwater⋅Vsubmerged, water⋅g=1⋅(52⋅x)⋅g=25x⋅g
- Fmercury=ρmercury⋅Vsubmerged, mercury⋅g=13.6⋅(52⋅(5−x))⋅g=340(5−x)⋅g
5. Equate the Weight and Buoyant Forces:
Setting the total buoyant force equal to the weight of the cube:
975g=25xg+340(5−x)g
Dividing through by g:
975=25x+340(5−x)
6. Solve for x:
Expanding and rearranging the equation:
975=25x+1700−340x
975−1700=−315x
−725=−315x
x=725315≈2.3cm
Final Answer
The depth of the water layer x is approximately 2.3cm.
We have a cube of iron with an edge of 5 cm floating on mercury. When water is poured on top, the cube becomes fully immersed. We need to find the depth of the water layer (denoted as x).
The volume V of the cube can be calculated using the formula for the volume of a cube:
V=edge3=(5cm)3=125cm3
The weight W of the iron cube can be calculated using the formula:
W=Volume×Density×g
The density of iron can be found using its specific gravity:
Density of iron=Specific gravity×Density of water=7.8×1g/cm3=7.8g/cm3
Therefore, the weight of the cube is:
W=125cm3×7.8g/cm3×g=975g×g
The buoyant force Fb acting on the cube is equal to the weight of the fluid displaced by the cube. The total buoyant force is the sum of the buoyant forces from both the water and the mercury:
Fb=Fwater+Fmercury
Where:
- Fwater=ρwater⋅Vsubmerged, water⋅g=1⋅(52⋅x)⋅g=25x⋅g
- Fmercury=ρmercury⋅Vsubmerged, mercury⋅g=13.6⋅(52⋅(5−x))⋅g=340(5−x)⋅g
Setting the total buoyant force equal to the weight of the cube:
975g=25xg+340(5−x)g
Dividing through by g:
975=25x+340(5−x)
Expanding and rearranging the equation:
975=25x+1700−340x
975−1700=−315x
−725=−315x
x=725315≈2.3cm
The depth of the water layer x is approximately 2.3cm.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Single Correct
95 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Multipe Correct
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.4
10 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A cubical metal block of edge 12 cm floats in mercry with one fifth of the height inside the mercury. Water poured till the surface of the block is just immersed in it. Find the height of the water column to be poured. Specific gravity of mercury =13.6.
View SolutionA block of iron floats on mercury. Find the fractionof volume which remains immersed in mercury. (Densities of iron and mercury are 7.8gcm−3 and 13.6gcm−3 respectively).
View SolutionA rectangular container has base of length 12 cm and width 9 cm . A cube of edge 6 cm is placed in the container and then sufficient water is filled into it so that the cube is just submerged . Find the fall in level of the water. In the container , when the cube is removed.
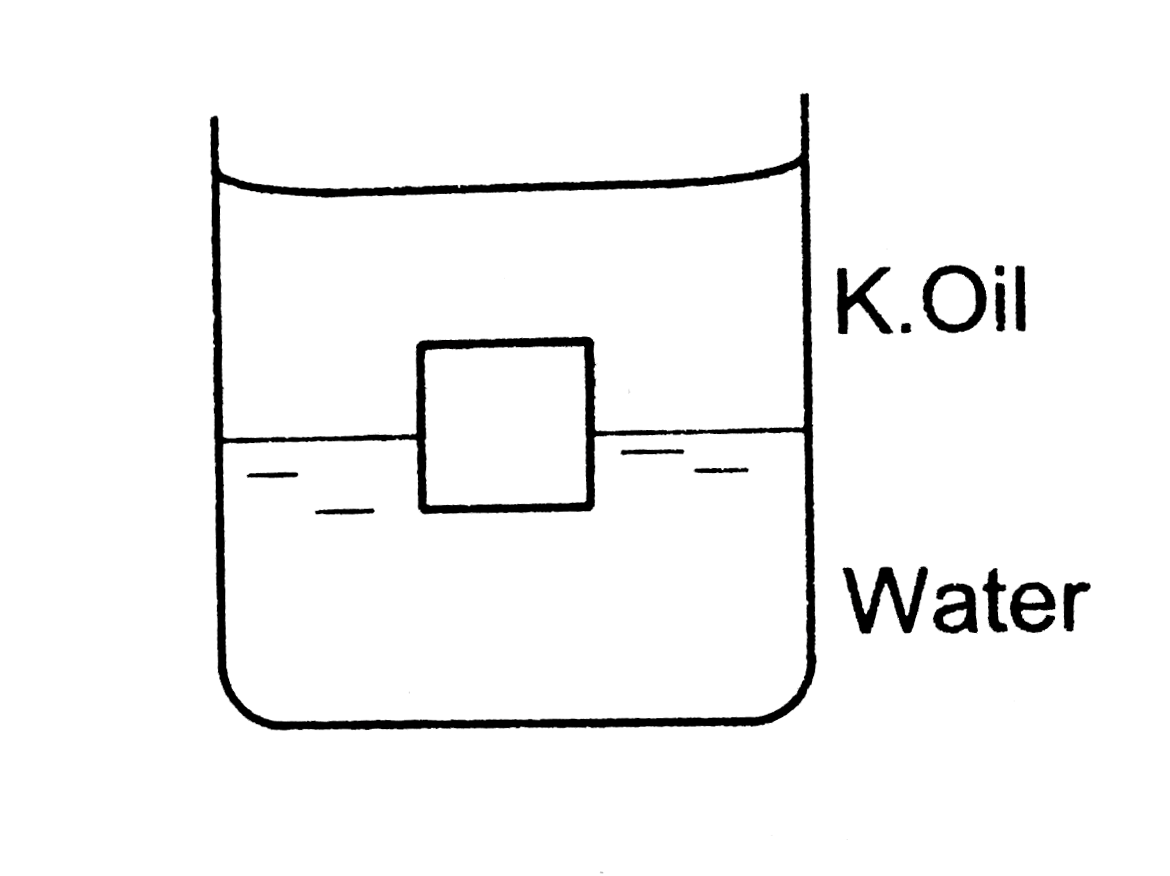
View SolutionA cube of ice floats partly in water and partly in K.oil figure. Find the ratio of the volume of ice immersed in water to that in K.oil. Specific gravity of K.oil is 0.8 and that of ice is 0.9.
View SolutionA tank contains water on top of mercury . A cube of iron, 60mm along each edge, is sitting upright in equilibrium in the liquids. Find how much of it is in each liquid. The densities of iron and mercury are 7.7×103kg/m3 and 13.6×103kg/m3 respectively
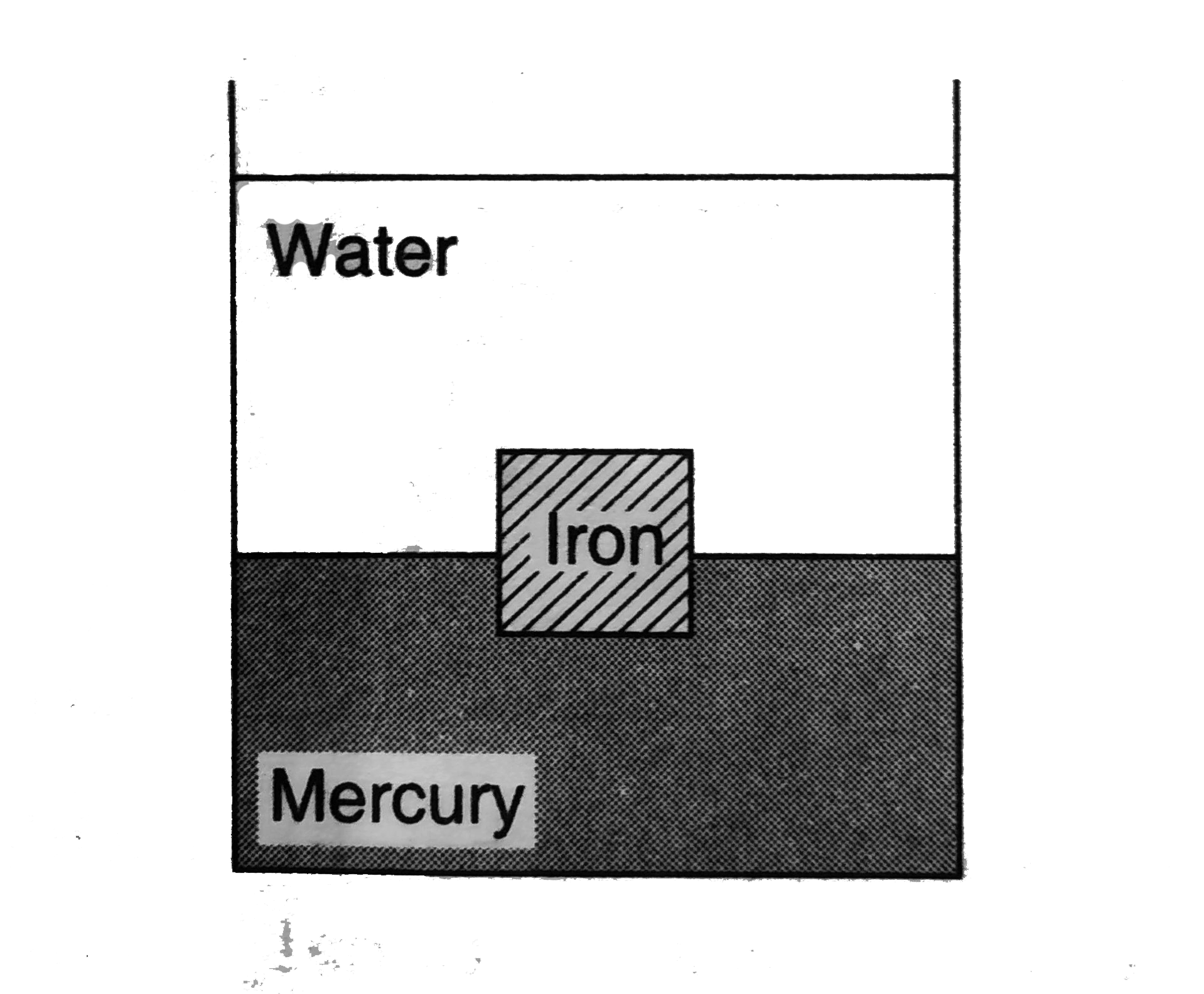
View SolutionA tank contains water on top of mercury . A cube of iron, 60mm along each edge, is sitting upright in equilibrium in the liquids. Find how much of it is in each liquid. The densities of iron and mercury are 7.7×103kg/m3 and 13.6×103kg/m3 respectively

View SolutionAn ice cube of side 1cm is floating at the interface of kerosene and water in a beaker of base area 10cm2. The level of kerosene is just covering the top surface of the ice cube.
a. Find the depth of submergence in the kerosene and that in the water.
b. Find the change in the total level of the liquid when the whole ice melts into water.

View SolutionA cubic body floats on mercury with 0.25 fraction of its volume below the surface. What fraction of the volume of the body will be immeresed in the mercury if a layer of water poured on top of the mercury covers the body conpletely.
View SolutionIf 15.0 cm of water and spirit each are further poured into the respective arms of the tube, what is the difference in the levels of mercury in the two arms? (Relative density of mercury = 13.6).
View SolutionA cube of 9cm edge is immersed completely in a rectangular vessel containing water. If the dimensions of the base are 15cmand12cm. Find the rise in water level in the vessel.
View Solution
