Eight charges, 1muC, -7muC , -4muC, 10muC, 2muC, -5muC, -3muC, and 6muC, are situated at the eight cornersof a cube of side 20 cm. A spherical surface of radius 80 cm encloses this cube. The center of the sphere coincides with the center of the cube. Then, the total outgoing flux from the spherical surface (in units of Vm) is
36π×103
684π×103
zero
none of these
The correct Answer is:C
To solve the problem, we need to calculate the total outgoing electric flux from a spherical surface that encloses a cube with charges located at its corners. We will follow these steps:
Step 1: Identify the Charges
The charges located at the corners of the cube are:
- Q1=1μC
- Q2=−7μC
- Q3=−4μC
- Q4=10μC
- Q5=2μC
- Q6=−5μC
- Q7=−3μC
- Q8=6μC
Step 2: Calculate the Total Charge Enclosed
We need to sum all the charges to find the total charge enclosed within the spherical surface.
Qtotal=Q1+Q2+Q3+Q4+Q5+Q6+Q7+Q8
Substituting the values:
Qtotal=1+(−7)+(−4)+10+2+(−5)+(−3)+6
Calculating step-by-step:
1. 1−7=−6
2. −6−4=−10
3. −10+10=0
4. 0+2=2
5. 2−5=−3
6. −3−3=−6
7. −6+6=0
So, the total charge enclosed Qtotal=0μC.
Step 3: Apply Gauss's Law
According to Gauss's Law, the electric flux Φ through a closed surface is given by:
Φ=Qenclosedϵ0
Where ϵ0 is the permittivity of free space. Since Qtotal=0:
Φ=0ϵ0=0
Conclusion
The total outgoing electric flux from the spherical surface is 0Vm.
Final Answer
The total outgoing flux from the spherical surface is 0Vm.
---
The charges located at the corners of the cube are:
- Q1=1μC
- Q2=−7μC
- Q3=−4μC
- Q4=10μC
- Q5=2μC
- Q6=−5μC
- Q7=−3μC
- Q8=6μC
We need to sum all the charges to find the total charge enclosed within the spherical surface.
2. −6−4=−10
3. −10+10=0
4. 0+2=2
5. 2−5=−3
6. −3−3=−6
7. −6+6=0
According to Gauss's Law, the electric flux Φ through a closed surface is given by:
The total outgoing electric flux from the spherical surface is 0Vm.
The total outgoing flux from the spherical surface is 0Vm.
Topper's Solved these Questions
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Multiple Correct
8 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Comprehension
36 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Subjective
12 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Interger
8 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.5
15 Videos
Similar Questions
Electric charges of +10μC,+5μC,−3μCand+8μC are placed at the corners of a square of side √2 m, the potential at the centre of the square is
View SolutionCharges 5μC,−2μC,3μCand−9μC are placed at the corners A,B,C and D of a square ABCD of side 1m. The net electric potential at the centre of th square is
View SolutionA charge of 1 coulomb is located sat the centre of a sphere of raidus 10 cm and a cube of side 20 cm. The ratio of outgoing flux the sphere and cube will be
View SolutionA charge Q μc is placed at the centre of cube, the flux coming out from any surfaces will be : -
View SolutionFour point charges 1μC,3μC,−2μCand−2μC are arranged on the four vertices of a square of side 1 cm. The dipole moment of this charge assembly is
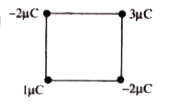
View SolutionFour charges of 6μC,2μC,−12μCand4μC are placed at the corners of a square of side 1m. The square is in x-y plane and its centre is at origin. Electric potential due to these charges is zero everywhere or the line
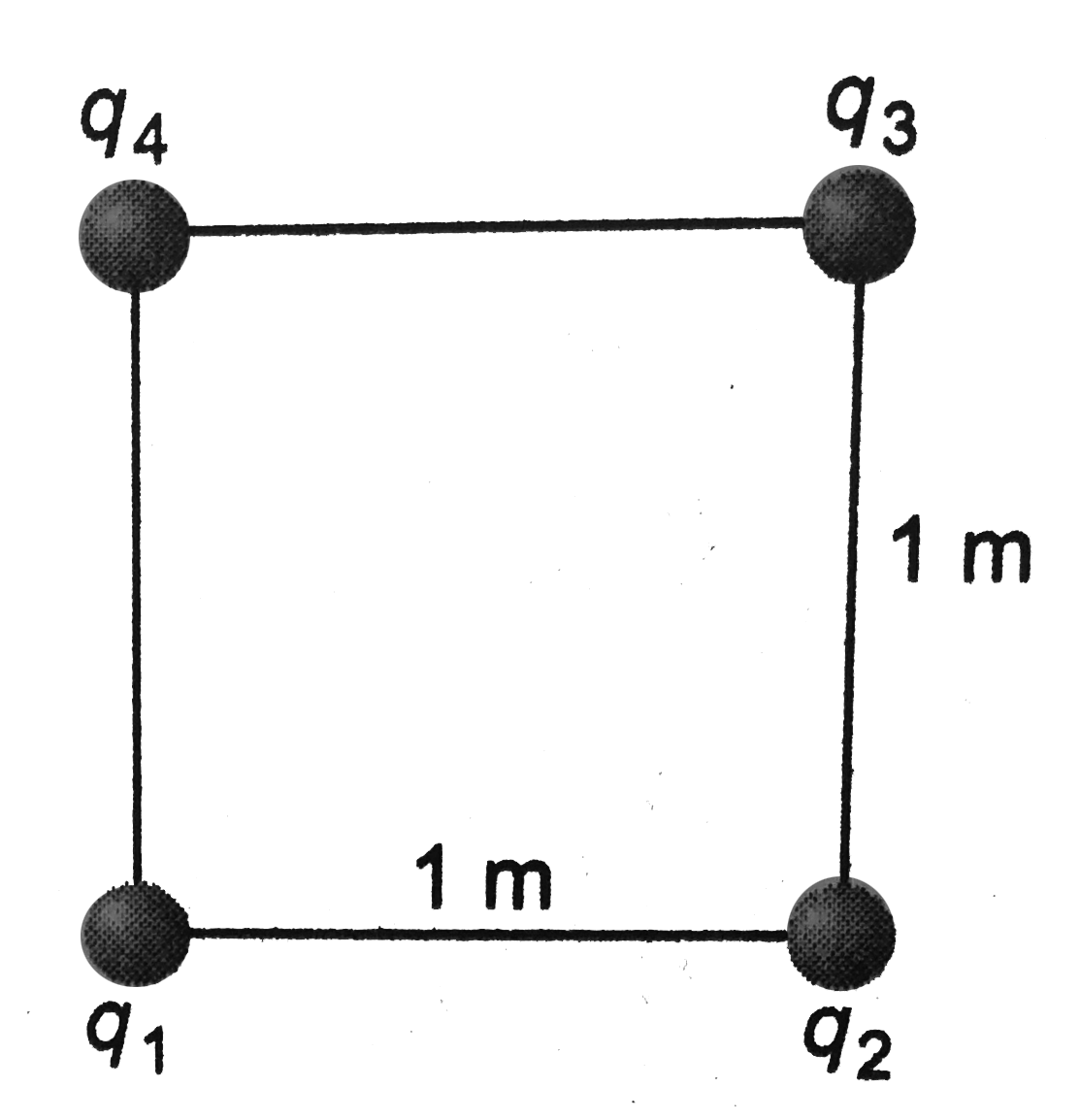
View SolutionFor charges q1=1μC,q2=2μC,q3=−3μC and q4=4μC are kept on the vertices of a square of side 1m. Find the electric potential energy of this system of charges.
View SolutionA charge qμC is placed at the centre of a cube of a side 0.1m, then the electric flux diverging from each face of the cube is
View SolutionA charge 10μC is placed at the centre of a hemisphere of radius R=10cm as shown. The electric flux through the hemisphere (in MKS units)is

View SolutionFour point charges are placed at the corners of a square ABCD of side 10 cm, as shown in figure. The force on a charge of 1μC placed at the centre of square is

View Solution
