Electric charges of +10μC,+5μC,−3μCand+8μC are placed at the corners of a square of side √2 m, the potential at the centre of the square is
1.8 V
1.8×106V
1.8×105V
1.8×104V
The correct Answer is:C
To find the electric potential at the center of a square with charges placed at its corners, we can follow these steps:
Step 1: Understand the Configuration
We have a square with side length √2 m. The charges at the corners are:
- Charge QA=+10μC
- Charge QB=+5μC
- Charge QC=−3μC
- Charge QD=+8μC
Step 2: Calculate the Distance from the Center to Each Charge
The distance from the center of the square to each corner can be calculated using the properties of a square. The distance from the center to any corner (let's denote it as r) can be derived as follows:
- The diagonal of the square can be calculated using the Pythagorean theorem:
d=√(√2)2+(√2)2=√2+2=√4=2m
- The distance from the center to a corner is half of the diagonal:
r=d2=22=1m
Step 3: Calculate the Electric Potential from Each Charge
The electric potential V due to a point charge is given by the formula:
V=kQr
where k=9×109N m2/C2 is Coulomb's constant, Q is the charge, and r is the distance from the charge to the point where the potential is being calculated.
Step 4: Calculate the Total Potential at the Center
Since the potential is a scalar quantity, we can simply add the potentials due to each charge:
Vtotal=VA+VB+VC+VD
Substituting the values:
VA=kQAr=9×10910×10−61=90,000V
VB=kQBr=9×1095×10−61=45,000V
VC=kQCr=9×109−3×10−61=−27,000V
VD=kQDr=9×1098×10−61=72,000V
Now, adding these potentials together:
Vtotal=90,000+45,000−27,000+72,000
Calculating this gives:
Vtotal=90,000+45,000+72,000−27,000=180,000V
Step 5: Express the Final Answer
The total potential at the center of the square is:
Vtotal=180,000V=1.8×105V
Final Answer
The potential at the center of the square is 1.8×105V.
---
We have a square with side length √2 m. The charges at the corners are:
- Charge QA=+10μC
- Charge QB=+5μC
- Charge QC=−3μC
- Charge QD=+8μC
The distance from the center of the square to each corner can be calculated using the properties of a square. The distance from the center to any corner (let's denote it as r) can be derived as follows:
- The diagonal of the square can be calculated using the Pythagorean theorem:
d=√(√2)2+(√2)2=√2+2=√4=2m
- The distance from the center to a corner is half of the diagonal:
r=d2=22=1m
The electric potential V due to a point charge is given by the formula:
V=kQr
where k=9×109N m2/C2 is Coulomb's constant, Q is the charge, and r is the distance from the charge to the point where the potential is being calculated.
Since the potential is a scalar quantity, we can simply add the potentials due to each charge:
Vtotal=VA+VB+VC+VD
Substituting the values:
VA=kQAr=9×10910×10−61=90,000V
VB=kQBr=9×1095×10−61=45,000V
VC=kQCr=9×109−3×10−61=−27,000V
VD=kQDr=9×1098×10−61=72,000V
Vtotal=90,000+45,000−27,000+72,000
Calculating this gives:
Vtotal=90,000+45,000+72,000−27,000=180,000V
The total potential at the center of the square is:
Vtotal=180,000V=1.8×105V
The potential at the center of the square is 1.8×105V.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.5
20 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
Four point charges are placed at the corners of a square of side l. Calculate potential at the centre of square.

View SolutionCharges 5μC,−2μC,3μCand−9μC are placed at the corners A,B,C and D of a square ABCD of side 1m. The net electric potential at the centre of th square is
View SolutionFour charges of 6μC,2μC,−12μCand4μC are placed at the corners of a square of side 1m. The square is in x-y plane and its centre is at origin. Electric potential due to these charges is zero everywhere or the line
View SolutionFour charges q1=1μC,q2=2μC,q3=−3μCandq4=4μC are kept on the vertices of a square of side 1m. Find the electric potential energy of this system of charges.
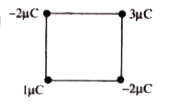
View SolutionFour point charges 1μC,3μC,−2μCand−2μC are arranged on the four vertices of a square of side 1 cm. The dipole moment of this charge assembly is
View SolutionElectric charge of 1μC,−1μC and 2μC are placed in air at the corners A, B and C respectively of an equilateral triangle ABC having length of each side 10 cm. The resultant force on the charge at C is
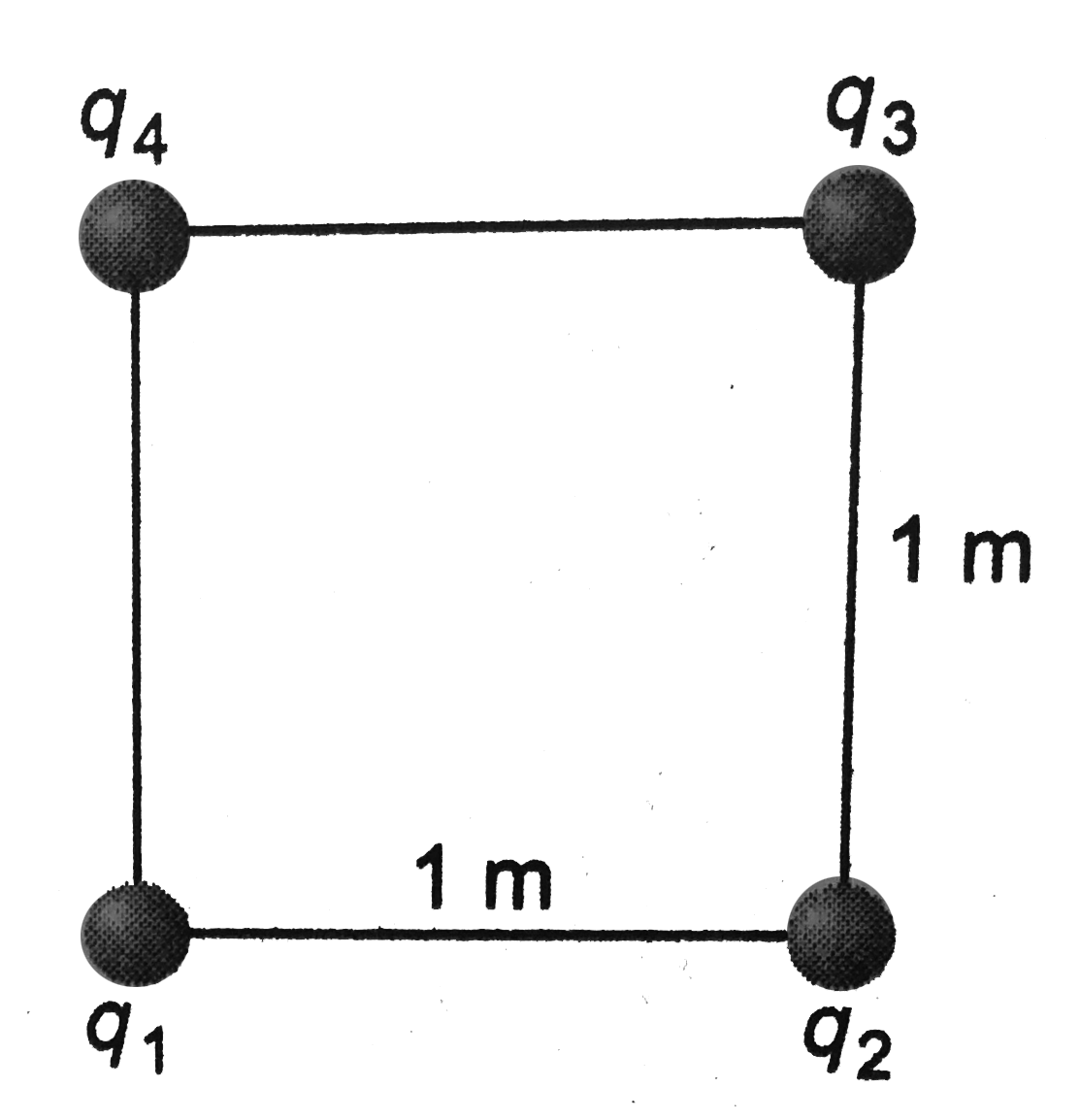
View SolutionFor charges q1=1μC,q2=2μC,q3=−3μC and q4=4μC are kept on the vertices of a square of side 1m. Find the electric potential energy of this system of charges.
View SolutionEight charges, 1muC, -7muC , -4muC, 10muC, 2muC, -5muC, -3muC, and 6muC, are situated at the eight cornersof a cube of side 20 cm. A spherical surface of radius 80 cm encloses this cube. The center of the sphere coincides with the center of the cube. Then, the total outgoing flux from the spherical surface (in units of Vm) is
View SolutionFour point charges are placed at the corners of a square ABCD of side 10 cm, as shown in figure. The force on a charge of 1μC placed at the centre of square is

View SolutionA charge qμC is placed at the centre of a cube of a side 0.1m, then the electric flux diverging from each face of the cube is
View Solution
