A point charge q1 is placed inside cavity 1 and another point charge q2 is inside cavity 2. A point charge q is placed outside the conductor. For the situation described above, answer the following questions.
If charge q2 is at point Q (inside cavity 2), then →E at the center of cavity 2 due to induced charge on the surface of cavity 2 would be
q2/(4πε0r'22) toward q2
q2/(4πε0r22) away from q2
zero
cannot be determined
The correct Answer is:D
To solve the problem, we need to analyze the situation step by step:
Step 1: Understanding the Setup
We have a conductor with two cavities. Inside cavity 1, there is a point charge q1, and inside cavity 2, there is another point charge q2. Additionally, there is an external point charge q placed outside the conductor.
Step 2: Induced Charges on the Cavity Surfaces
When a charge q2 is placed inside cavity 2, it will induce a charge on the inner surface of cavity 2. The induced charge will be equal in magnitude but opposite in sign to q2, thus it will be −q2. However, this induced charge will not be uniformly distributed over the surface of cavity 2 due to the point nature of q2.
Step 3: Electric Field Due to Induced Charge
The electric field →E at any point inside a cavity due to induced charges on the surface of that cavity can be calculated using Gauss's law. However, because the induced charge on the surface of cavity 2 is non-uniform (due to the point charge q2), we cannot simply calculate the electric field at the center of cavity 2.
Step 4: Conclusion
Since the induced charge on the surface of cavity 2 is non-uniform, the electric field →E at the center of cavity 2 cannot be determined. Therefore, the answer is that the electric field at the center of cavity 2 due to the induced charge on the surface of cavity 2 is not defined.
Final Answer
The electric field →E at the center of cavity 2 due to the induced charge on the surface of cavity 2 is undefined (option D).
---
We have a conductor with two cavities. Inside cavity 1, there is a point charge q1, and inside cavity 2, there is another point charge q2. Additionally, there is an external point charge q placed outside the conductor.
When a charge q2 is placed inside cavity 2, it will induce a charge on the inner surface of cavity 2. The induced charge will be equal in magnitude but opposite in sign to q2, thus it will be −q2. However, this induced charge will not be uniformly distributed over the surface of cavity 2 due to the point nature of q2.
The electric field →E at any point inside a cavity due to induced charges on the surface of that cavity can be calculated using Gauss's law. However, because the induced charge on the surface of cavity 2 is non-uniform (due to the point charge q2), we cannot simply calculate the electric field at the center of cavity 2.
Since the induced charge on the surface of cavity 2 is non-uniform, the electric field →E at the center of cavity 2 cannot be determined. Therefore, the answer is that the electric field at the center of cavity 2 due to the induced charge on the surface of cavity 2 is not defined.
The electric field →E at the center of cavity 2 due to the induced charge on the surface of cavity 2 is undefined (option D).
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - Interger Answer type
20 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - Fill in the blanks
10 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - Assertion and Reason Type
8 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Multiple Correct Answer type
2 Videos
- MISCELLANEOUS VOLUME 5BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Integer
12 Videos
Similar Questions
A point charge q1 is placed inside cavity 1 and another point charge q2 is inside cavity 2. A point charge q is placed outside the conductor. For the situation described above, answer the following questions.
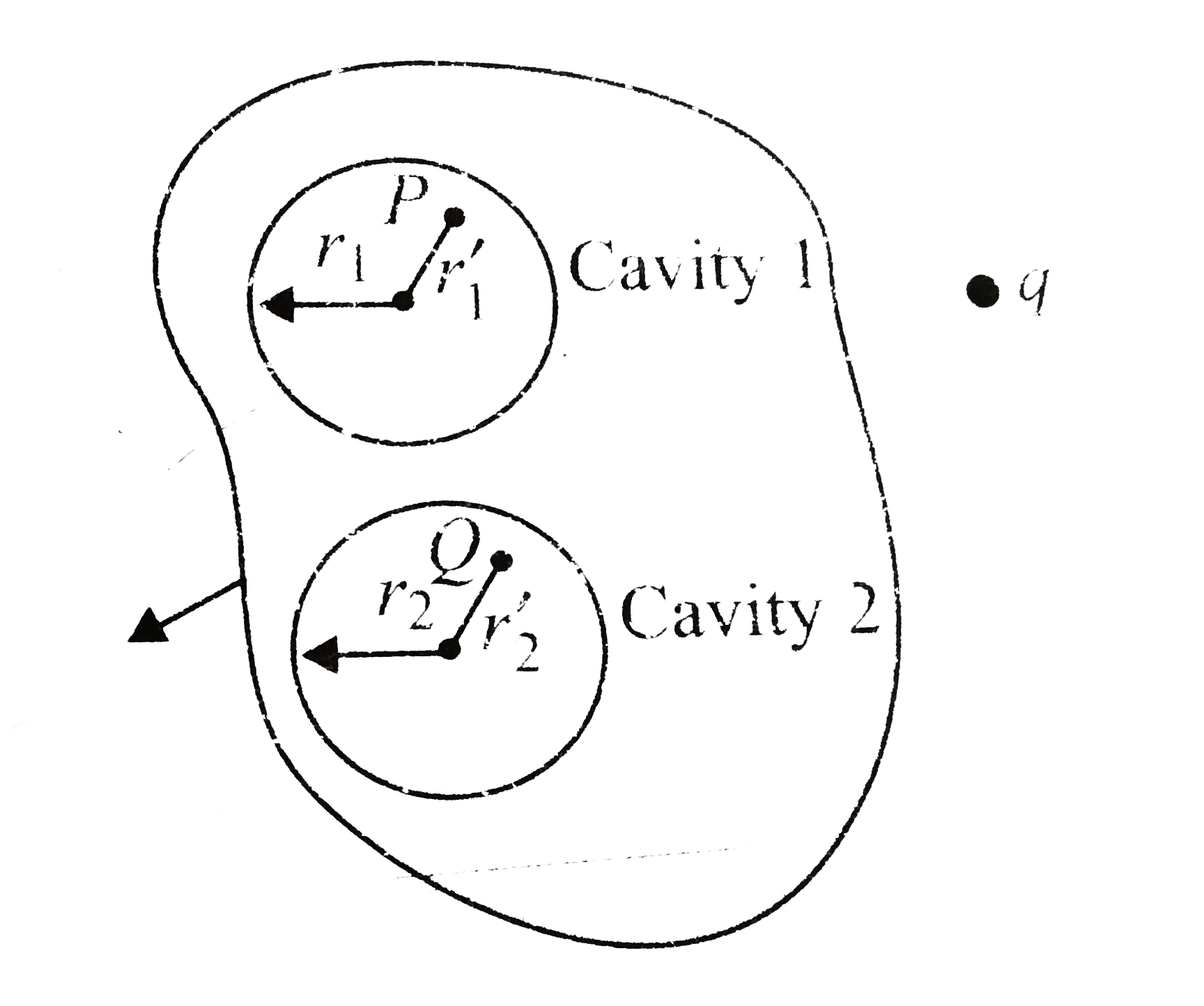
The charge on outer surface of the conductor would be
View SolutionA point charge q1 is placed inside cavity 1 and another point charge q2 is inside cavity 2. A point charge q is placed outside the conductor. For the situation described above, answer the following questions.
If the potential of the conductor is V0 and charge q2 is placed at the center of cavity 2, then potential at point Q is
View SolutionA point charge q1 is placed inside cavity 1 and another point charge q2 is inside cavity 2. A point charge q is placed outside the conductor. For the situation described above, answer the following questions.
→E inside the conductor at point S distant r from point charge q, due to charge on outer surface of the conductor, would be
View SolutionA positive point charge q1 is placed at (a,0) and another positive point charge q2 is placed at (0,a) . Find the force acting on q1.
View SolutionA point charge q is placed in a cavity in a metal block. If a charge Q is brought outside the metal, will the charge q feel an electric force?
View SolutionA conducting sphere of radius R2 has a spherical cavity of radius R1 which is non concentric with the sphere. A point charge q1 is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions.
Electric potential at the centre of the cavity will be :
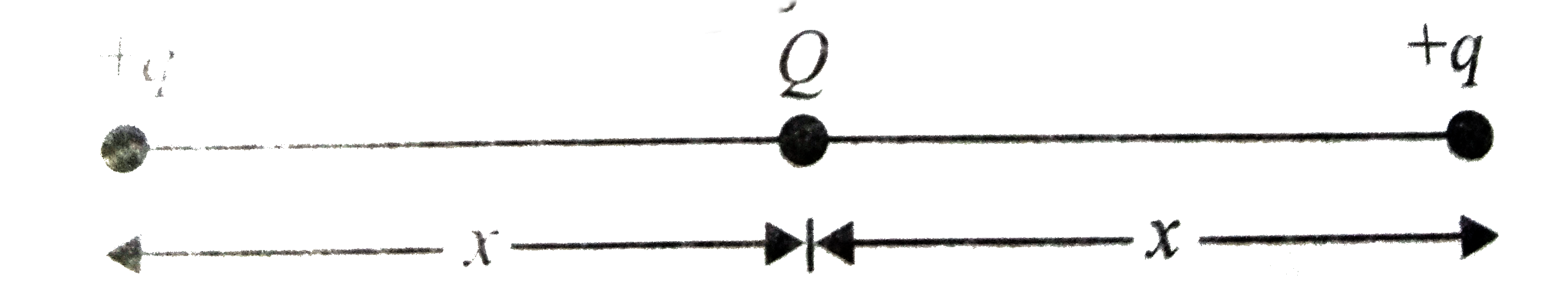
View SolutionA charge Q is placed at the centre of the line joining two point charges +q and +q as shown in figure. The ratio of charges Q and q is
View SolutionA charge q is placed at O in the cavity in a sphereical uncharged conductor. Points S is outside the conductor. If q is displaced from O towards S (still remaining within the cavity)

View SolutionA conducting sphere of radius R2 has a spherical cavity of radius R1 which is non concentric with the sphere. A point charge q1 is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions.
If q1 is shifted to the centre of the cavity, then: (choose the correct alternative)
View SolutionA positive charge q is placed in a spherical cavity made in a positively charged sphere. The centres of sphere and cavity are displaced by a small distance →l. Force on charge q is :
View Solution
