A spherical metal shell A of radius RA and a solid metal sphere B of radius RB(<RA) are kept far apart and each is given charge '+Q'. Now they are connected by a thin metal wire. Then
EinsideA = 0
QA>QB
σAσB=RBRA
Eon surfaceA<Eon surfaceB
From Eqs. (I) and (ii)
QAQB=RARB
Solving Eqs. (i) and (ii) we get
QA=2QRARA+RB and QB=2QRBRA+RB or QA>QB
σAσB=QA/4πR2AQB/4πR2B=RBRA using Eq. (iii) ,
QB>QA (as RA>RB)
On surface, EA=σAε0 and EB=σBε0
or EA<EB (∵σA<σB)
Similar Questions
A spherical conductor A of radius r is placed concentrically inside a conducting shell B of radius R(R>r). A charge Q is given to A , and then A is joined to B by a metal wire. The charge flowing from A to B will be
View SolutionFigure shows a solid metal sphere of radius a surrounded by a concentric thin metal shell of radius 2a. Initially both are having charges Q each, When the two are connected by a conducting wire as shown in the figure, then amount of heat produced in this process will be :
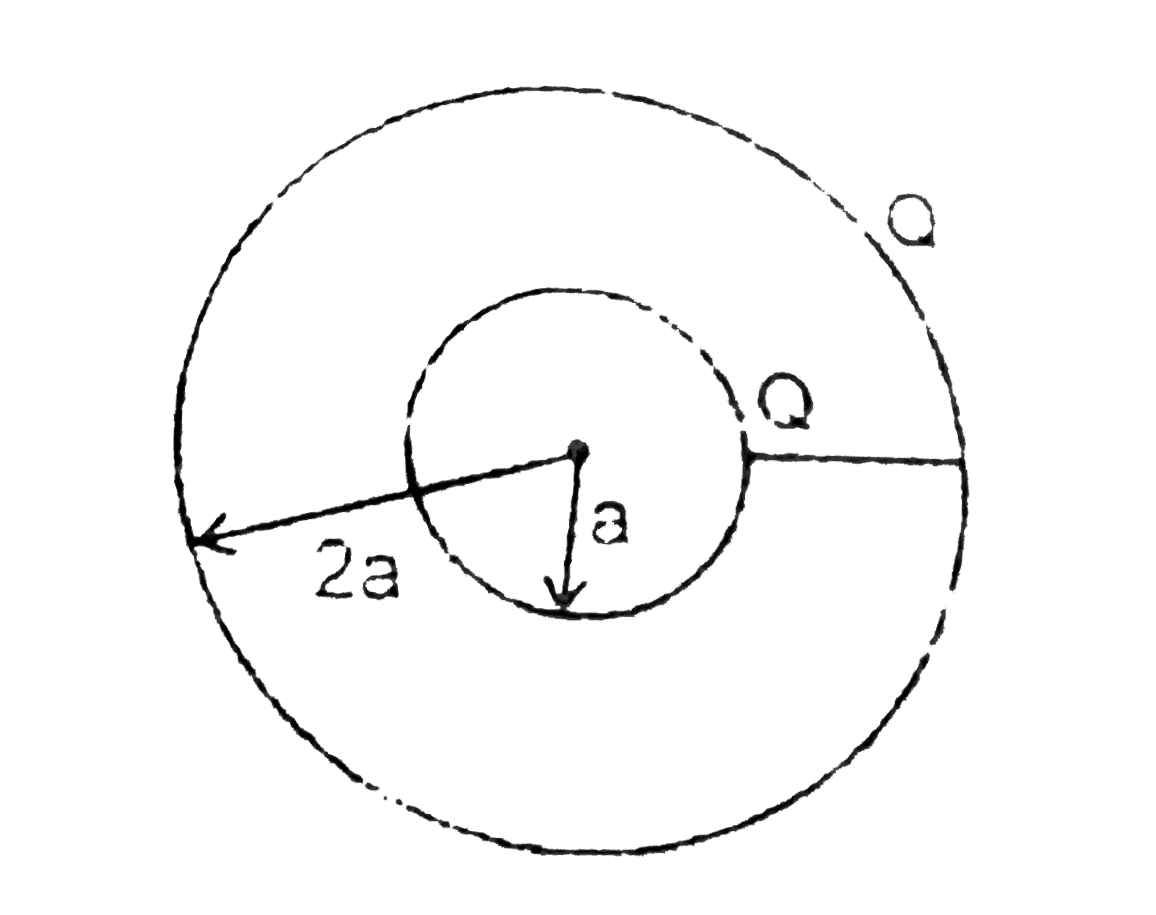 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Metallic sphere of radius R is charged to potential V. Then charge q is proportional to
AVBRCboth V and RDnone of these
Two metal spheres A and B of radii a & b (a < b) respectively are at a large distance apart. Each sphere carries a charge of 100μO. the sphere are connected by a conducting wire, then
View SolutionA conducting sphere of radius R, carrying charge Q, lies inside uncharged conducting shell of radius 2R. If they are joined by a metal wire,
View SolutionFigure shows a system of three concentric metal shells, A, B and C with radii a, 2a and 3a respectively. Shell B is earthed and shell C is given a charge Q. Now if shell C is connected to shell A, then find the final charge on the shell B
 View Solution
View SolutionTwo metal spheres of radius r have centers at a distance d apart in air. Find the capacitance of the system.
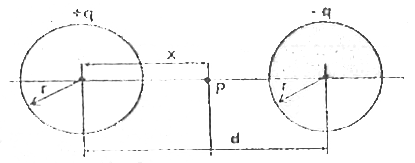 View Solution
View SolutionA solid metal sphere of radius R has a charg +2Q. A hollow spherical shell of radius 3R placed concentric with the frist sphere has net charge -Q.
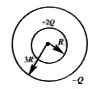
What would be the final distribution of charges if the sphres are joined by a conducting wireView SolutionConsider two concentric metalic shell's of radii R and 2R. The inner shell is having charge Q and outer shell is uncharged. If they are connected with a conducting wire. Then,
View SolutionA solid metallic sphere of radius a is surrounded by a conducting spherical shell of radius b(b>a). The solid sphere is given a charge Q. A student measures the potential at the surface of the solid sphere as V and the potential at the surface of spherical shell as Vb. After taking these readings, he decides . to put charge of −4Q on the shell. He then noted the readings of the potential of solid sphere and the shell and found that the potential difference is △V .
He then connected the outer spherical shell to the earth by a conducting wire and found that the charge on the outer surface of the shell as He then decides to remove the earthing connection from the shell and earthed the inner solid sphere. Connecting the inner sphere with the earth he observes the charge on the solid sphere as q2. He then wanted to check what happens if the two are connected by the conducting wire. So he removed the earthing connection and connected a conducting wire between the solid sphere and the spherical shelll. After the connections were made he found the charge on the outer shell as q3.
Potential difference (△CV) measured by the student between the inner solid shere and outer shell after putting a charge −4Q isView Solution

