In the path of a uniform light beam of large cross-sectional area and intensity I. a solid sphere of redius R which is perfectly reflecting is placed . Find the force exerted on this sphere due to the light beam.
To find the force exerted on a perfectly reflecting solid sphere placed in the path of a uniform light beam of intensity I, we can follow these steps:
Step 1: Understand the Concept of Intensity and Area
The intensity I of the light beam is defined as the power per unit area. The projected area A of the sphere that faces the light beam is given by:
A=πR2
where R is the radius of the sphere.
Step 2: Relate Power to Intensity
The power P incident on the sphere can be expressed as:
P=I⋅A=I⋅πR2
Step 3: Determine the Momentum Change of Photons
Photons have momentum given by:
p=hλ
where h is Planck's constant and λ is the wavelength of the light. When a photon strikes a perfectly reflecting surface, it reverses its momentum. Therefore, the change in momentum Δp for one photon is:
Δp=pfinal−pinitial=hλ−(−hλ)=2hλ
Step 4: Calculate the Number of Photons per Second
The number of photons N striking the sphere per second can be found from the power:
P=N⋅(energy of one photon)=N⋅hλ
Thus, we can express N as:
N=P⋅λh=I⋅πR2⋅λh
Step 5: Calculate the Force
The force F exerted on the sphere due to the change in momentum of the photons can be calculated using:
F=N⋅Δp
Substituting the expressions for N and Δp:
F=(I⋅πR2⋅λh)⋅(2hλ)
Simplifying this, we find:
F=I⋅πR2⋅2hh=2I⋅πR2
Final Result
Thus, the force exerted on the sphere due to the light beam is:
F=2πIR2c
where c is the speed of light.
---
The intensity I of the light beam is defined as the power per unit area. The projected area A of the sphere that faces the light beam is given by:
A=πR2
where R is the radius of the sphere.
The power P incident on the sphere can be expressed as:
P=I⋅A=I⋅πR2
Photons have momentum given by:
p=hλ
where h is Planck's constant and λ is the wavelength of the light. When a photon strikes a perfectly reflecting surface, it reverses its momentum. Therefore, the change in momentum Δp for one photon is:
Δp=pfinal−pinitial=hλ−(−hλ)=2hλ
The number of photons N striking the sphere per second can be found from the power:
P=N⋅(energy of one photon)=N⋅hλ
Thus, we can express N as:
N=P⋅λh=I⋅πR2⋅λh
The force F exerted on the sphere due to the change in momentum of the photons can be calculated using:
F=N⋅Δp
Substituting the expressions for N and Δp:
F=(I⋅πR2⋅λh)⋅(2hλ)
Simplifying this, we find:
F=I⋅πR2⋅2hh=2I⋅πR2
Thus, the force exerted on the sphere due to the light beam is:
F=2πIR2c
where c is the speed of light.
Topper's Solved these Questions
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Examples
8 Videos
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Exercise 3.1
15 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - ddp.5.5
14 Videos
- RAY OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.6
12 Videos
Similar Questions
A sphere of radius 1.00 cm is placed in the path of a parallel beam of light of large aperture. The intensity of the light is 0.50 W cm−2. If the sphere completely absorbs the radiation falling on it, find the force exerted by the light beam on the sphere.
View SolutionA point source of light is placed at the centre of curvature of a hemispherical surface. The radius of curvature is r and the inner surface is completely reflecting. Find the force on the hemisphere due to the light falling on its if the source emits a power W.
View Solution
Knowledge Check
A uniform ring of mass m and radius a is placed directly above a uniform sphere of mass M and of equal radius. The centre of the ring is at distance √3a from the centre of the sphere. Find the gravitational force exerted by the sphere on the ring.
AGMm8r2BGMm4r2C√3GMm8r2DGMm8r3√3
A light beam of wavelength λ incident on a silver sphere of radius r. suspended by a nonconducting thread. Find potentical aquired by sphere
View Solution_S01_083_Q01.png)
Water (density ρ) is flowing through the uniform tube of cross-sectional area A with a constant speed v as shown in the figure. Find the magnitude of force exerted by the water on the curved corner of the tube is (neglect viscous forces)
View SolutionA horizontal ray of light is incident on a solid glass sphere of radius R and refractive index μ . What is net deviation of the beam when it emerged from the other side of the sphere?
View SolutionA uniform ring of mas m and radius a is placed directly above a uniform sphere of mass M and of equal radius. The centre of the ring is at a distance √3 a from the centre of the sphere. Find the gravitational force exerted by the sphere on the ring.
View SolutionA ray of light, travelling parallel to diameter of a sphere enters the sphere through a hole at point A as shown in the figure. The inner surface of the sphere is made perfectly reflecting. Find the number of reflections suffered by the ray before coming out of the sphere.

View SolutionA uniform ring of mas m and radius a is placed directly above a uniform sphere of mass M and of equal radius. The center of the ring is at a distance √3a from the center of the sphere. Find the gravitational force exerted by the sphere on the ring.
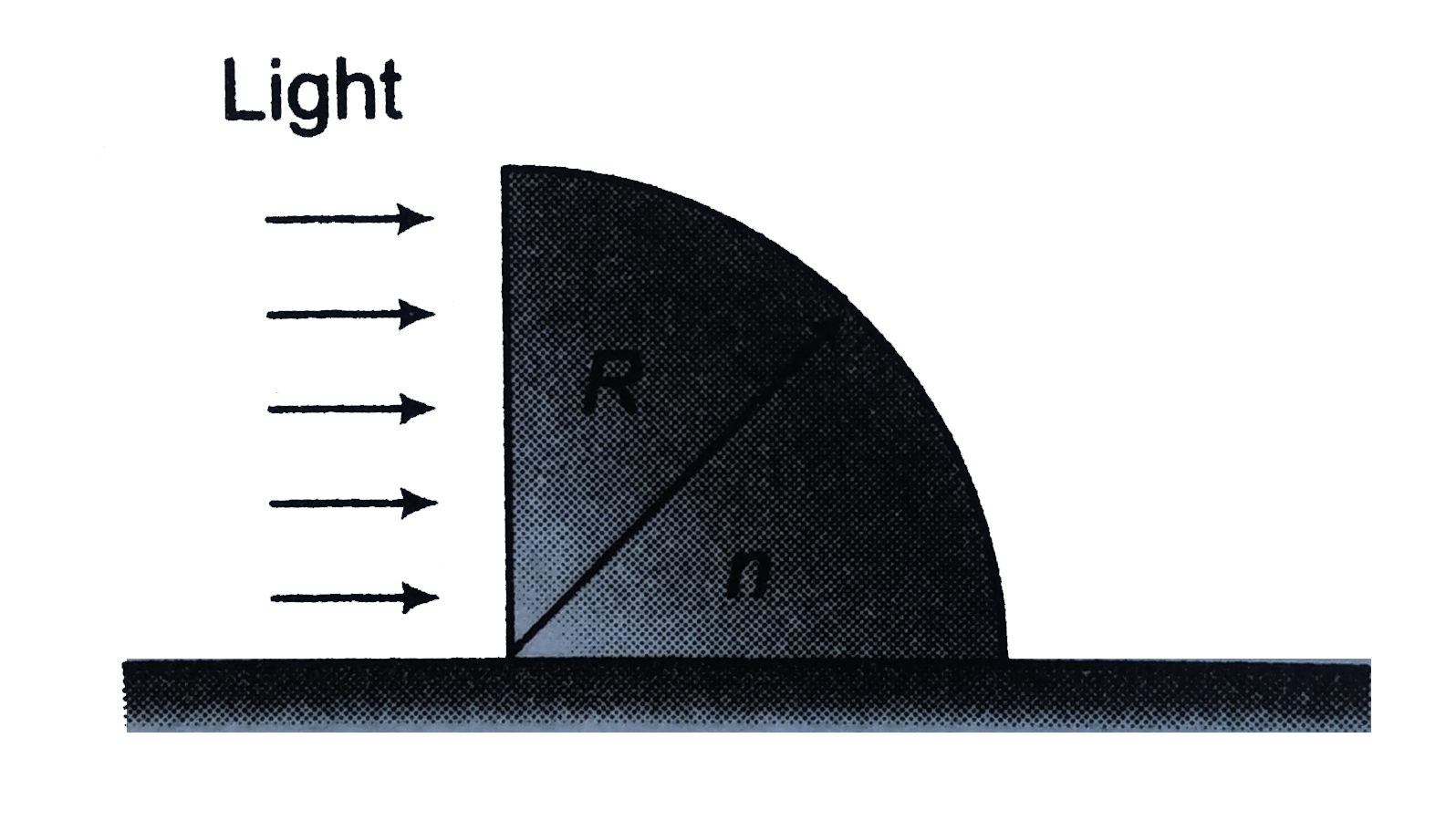
View SolutionA glass prism in the shape of a quarter cylinder lies on a horizontal table. A uniform, horizontal light beam falls on its vertical plane surface as shown in the figure. If the radius of the cylinder is R=5cm and the refractive index of the glass is n=1.5, where on the table beyond the cylinder, will a path of light be found?
View Solution
